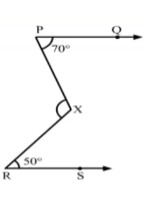
In Figure, if QT⊥PR, ∠TQR=40∘ and ∠SPR=30∘, find x and y.
To solve the problem step by step, we will use the properties of triangles and the fact that the sum of the angles in a triangle is always 180 degrees.
Step 1: Identify the angles in triangle QTR
Since QT is perpendicular to PR, we know that angle TQR is 40 degrees and angle T is 90 degrees (because of the perpendicularity).
Step 2: Calculate angle QRT (denote it as x)
Using the triangle angle sum property:
Angle T+Angle TQR+Angle QRT=180∘
Substituting the known values:
90∘+40∘+x=180∘
This simplifies to:
130∘+x=180∘
Now, isolate x:
x=180∘−130∘=50∘
Step 3: Identify the angles in triangle SPR
In triangle SPR, we know angle SPR is 30 degrees and we just found angle QRT (which is x) to be 50 degrees.
Step 4: Calculate angle PSQ (denote it as y)
Using the exterior angle theorem, we know that the exterior angle (angle PSQ) is equal to the sum of the two opposite interior angles (angle SPR and angle PRS):
Angle PSQ=Angle SPR+Angle PRS
Substituting the known values:
y=30∘+50∘
Thus:
y=80∘
Final Answers
Therefore, we have:
- x=50∘
- y=80∘
Since QT is perpendicular to PR, we know that angle TQR is 40 degrees and angle T is 90 degrees (because of the perpendicularity).
Using the triangle angle sum property:
Angle T+Angle TQR+Angle QRT=180∘
Substituting the known values:
90∘+40∘+x=180∘
This simplifies to:
130∘+x=180∘
Now, isolate x:
x=180∘−130∘=50∘
In triangle SPR, we know angle SPR is 30 degrees and we just found angle QRT (which is x) to be 50 degrees.
Using the exterior angle theorem, we know that the exterior angle (angle PSQ) is equal to the sum of the two opposite interior angles (angle SPR and angle PRS):
Angle PSQ=Angle SPR+Angle PRS
Substituting the known values:
y=30∘+50∘
Thus:
y=80∘
Therefore, we have:
- x=50∘
- y=80∘
Topper's Solved these Questions
Similar Questions
In Fig. 6.37, if QT⊥PR,∠TQR=40∘and∠SPR=30∘, find x and y.
View SolutionIf Figure, if QT⊥PR,∠TQR=400 AND ∠SPR=300, find x and y. Figure
View SolutionIn Figure, if PQ∣∣RS, ∠MXQ=135∘and ∠MYR=40∘,find ∠XMY
View SolutionIn Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
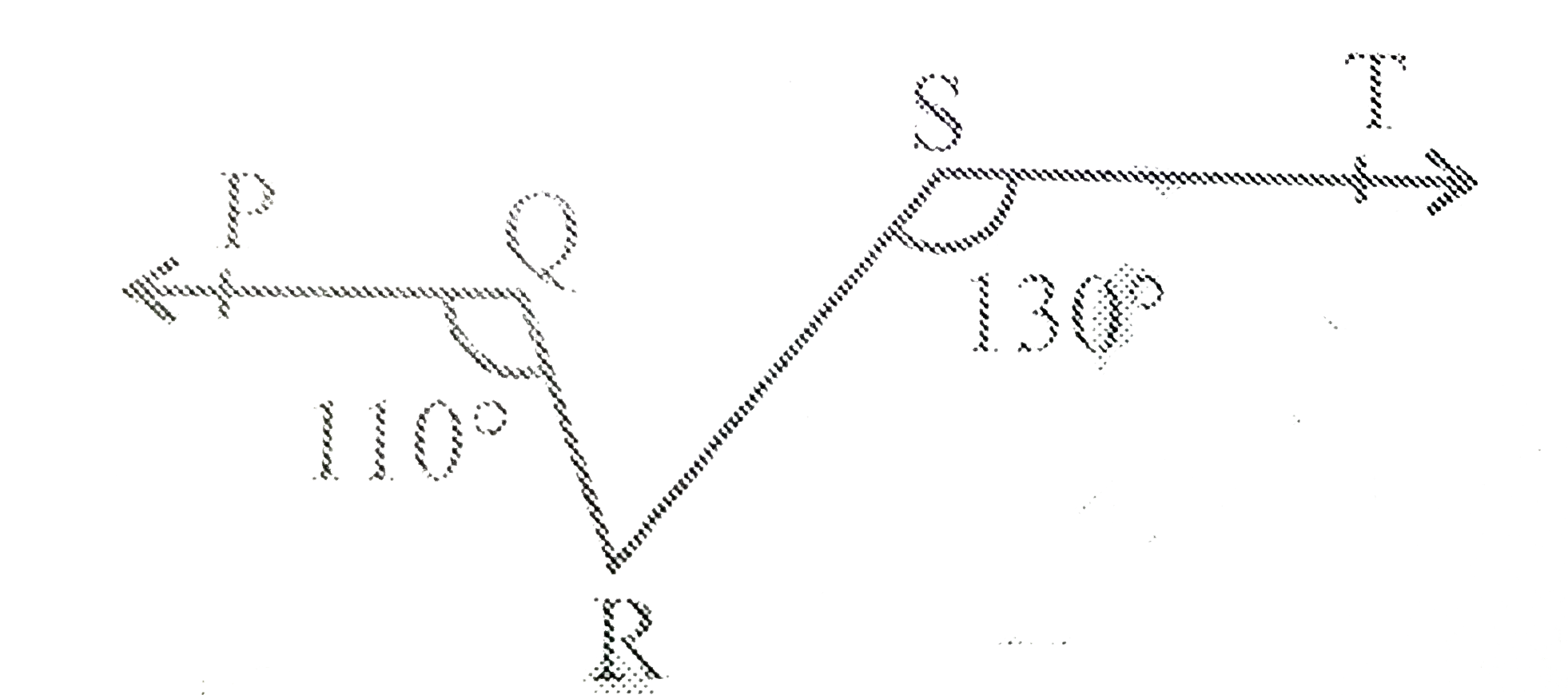
View SolutionIn Figure, if AB∣∣CD, ∠APQ=500AND ∠PRD=1270, find x and y
View SolutionIn Figure, if PQ⊥PS, PQSR, ∠SQR=280and ∠QRT=650, then find the values of x and y
View SolutionIn Fig. 6.24, if PQ∣∣RS,∠MXQ=135∘ and∠MYR=40∘,find ∠XMY.
View SolutionIn figure, PQ∣∣RS, ∠PAB=70∘and ∠ACS=100∘. Determine ∠ABC, ∠BAC and ∠CAQ
View SolutionIn Figure , find out ∠PXR, if PQ ∣∣ RS.
View SolutionIn figure, if PQ⊥PS,PQ∣∣SR,∠SQR=280 and ∠QRT=650, then find the values of x and y. Figure
View Solution
