Kamla has a triangular field with sides 240 m, 200 m, 360 m, where she grew wheat. In another triangular field with sides 240 m, 320 m, 400 m adjacent to the previous field, she wanted to grow potatoes and onions. She divided the field in two parts by joining the mid point of the longest side to the opposite vertex and grew potatoes in one part and onions in one part. How much area (in hectares) has been used for wheat,potatoes and onions? ( 1 hectare = 10000 m2 )
To solve the problem step by step, we will calculate the areas of the triangular fields where Kamla grew wheat, potatoes, and onions using Heron's formula.
Step 1: Calculate the area of the triangular field where Kamla grew wheat.
Given sides of the triangle:
- a=240m
- b=200m
- c=360m
Calculate the semi-perimeter (s):
s=a+b+c2=240+200+3602=8002=400m
Using Heron's formula to find the area (A):
A=√s(s−a)(s−b)(s−c)
Substituting the values:
A=√400(400−240)(400−200)(400−360)
=√400×160×200×40
Calculating the area:
=√400×160×200×40=16000√2m2
Convert area to hectares:
Area in hectares=16000√210000=1.6√2hectares
Step 2: Calculate the area of the triangular field where Kamla grew potatoes and onions.
Given sides of the triangle:
- a=240m
- b=320m
- c=400m
Calculate the semi-perimeter (s):
s=a+b+c2=240+320+4002=9602=480m
Using Heron's formula to find the area (A):
A=√s(s−a)(s−b)(s−c)
Substituting the values:
A=√480(480−240)(480−320)(480−400)
=√480×240×160×80
Calculating the area:
=√480×240×160×80=38400m2
Convert area to hectares:
Area in hectares=3840010000=3.84hectares
Step 3: Divide the area of the second triangular field into two equal parts for potatoes and onions.
Area for potatoes and onions:
Area for each=3.842=1.92hectares
Summary of Areas
- Area for wheat: 1.6√2hectares (approximately 2.26 hectares)
- Area for potatoes: 1.92hectares
- Area for onions: 1.92hectares
- a=240m
- b=200m
- c=360m
s=a+b+c2=240+200+3602=8002=400m
A=√s(s−a)(s−b)(s−c)
Substituting the values:
A=√400(400−240)(400−200)(400−360)
=√400×160×200×40
=√400×160×200×40=16000√2m2
Area in hectares=16000√210000=1.6√2hectares
- a=240m
- b=320m
- c=400m
s=a+b+c2=240+320+4002=9602=480m
A=√s(s−a)(s−b)(s−c)
Substituting the values:
A=√480(480−240)(480−320)(480−400)
=√480×240×160×80
=√480×240×160×80=38400m2
Area in hectares=3840010000=3.84hectares
Area for each=3.842=1.92hectares
- Area for wheat: 1.6√2hectares (approximately 2.26 hectares)
- Area for potatoes: 1.92hectares
- Area for onions: 1.92hectares
Topper's Solved these Questions
Similar Questions
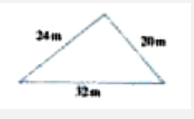
Find the cost of fencing a triangular garden with sides 20m,24m and 32m at the rate of 142 per metre.
View SolutionThe perimeter of a triangular field is 420 m and its sides are in the ratio 6 : 7 : 8. Find the area of the triangular field.
View SolutionFind the cost of laying grass in a triangular field of sides 50 m, 65 m and 65 m at the rate of rupee 7 per m2.
View SolutionThe area of a square field is 325 m2. Find the approximate length of one side of the field.
View SolutionFind the area of a square field if its each side is 1034 m long.
View SolutionThe perimeter of a triangular field is 240dm. If two of its sides are 78dm and 50dm, find the length of the perpendicular on the side of length 50dm from the opposite vertex.
View SolutionThe perimeter of a triangular field is 240dm. If two of its sides are 78dm and 50dm, find the length of the perpendicular on the side of length 50dm from the opposite vertex.
View SolutionA rectangular field is 48 m long and 20 m wide. How many right triangular flower beds, whose sides containing the right angle measure 12 m and 5 m can be laid in this field?
View SolutionFind the length of a side of a square, whose area is equal to the area of a rectangle with sides 240 m and 70 m.
View SolutionThrough a rectangular field of sides 90 m × 60 m , two roads are constructed which are parallel to the sides and cut each other at right angles through the centre of the field. If the width of the roads is 3 m, find the total area covered by the two roads.
View Solution
