Radha made a picture of an aeroplane with coloured paper as shown in Fig 12.15. Find the total area of the paper used.
To find the total area of the paper used to make the aeroplane picture, we will break down the shape into simpler geometric figures: triangles, a rectangle, and a trapezium. We will then calculate the area of each part and sum them up.
1. Identify the Shapes:
The aeroplane can be decomposed into:
- Triangle ABC
- Two right-angle triangles (CGF and DEB)
- Rectangle BCQP
- Trapezium PQRS
2. Calculate the Area of Triangle ABC:
- Given: AB = AC = 5 cm, BC = 1 cm
- Semi-perimeter (s) = (AB + AC + BC) / 2 = (5 + 5 + 1) / 2 = 5.5 cm
- Area (A) = √(s(s - AB)(s - AC)(s - BC))
- Area = √(5.5(5.5 - 5)(5.5 - 5)(5.5 - 1))
- Area = √(5.5 * 0.5 * 0.5 * 4.5) = √(6.1875) ≈ 2.48 cm²
3. Calculate the Area of Triangle CGF:
- Given: Base = 1.5 cm, Height = 6 cm
- Area = 1/2 * base * height = 1/2 * 1.5 * 6 = 4.5 cm²
- Since there are two identical triangles (CGF and DEB), total area = 2 * 4.5 = 9 cm²
4. Calculate the Area of Rectangle BCQP:
- Given: Length = 6.5 cm, Width = 1 cm
- Area = Length * Width = 6.5 * 1 = 6.5 cm²
5. Calculate the Area of Trapezium PQRS:
- Given: Top base (PQ) = 1 cm, Bottom base (RS) = 2 cm, Height = 1 cm
- Area = 1/2 * (Base1 + Base2) * Height = 1/2 * (1 + 2) * 1 = 1.5 cm²
6. Sum All Areas:
- Total Area = Area of Triangle ABC + Area of Triangle DEB + Area of Triangle CGF + Area of Rectangle BCQP + Area of Trapezium PQRS
- Total Area = 2.48 cm² + 9 cm² + 6.5 cm² + 1.5 cm² = 19.48 cm²
Final Answer:
The total area of the paper used is 19.48 cm².
The aeroplane can be decomposed into:
- Triangle ABC
- Two right-angle triangles (CGF and DEB)
- Rectangle BCQP
- Trapezium PQRS
- Given: AB = AC = 5 cm, BC = 1 cm
- Semi-perimeter (s) = (AB + AC + BC) / 2 = (5 + 5 + 1) / 2 = 5.5 cm
- Area (A) = √(s(s - AB)(s - AC)(s - BC))
- Area = √(5.5(5.5 - 5)(5.5 - 5)(5.5 - 1))
- Area = √(5.5 * 0.5 * 0.5 * 4.5) = √(6.1875) ≈ 2.48 cm²
- Given: Base = 1.5 cm, Height = 6 cm
- Area = 1/2 * base * height = 1/2 * 1.5 * 6 = 4.5 cm²
- Since there are two identical triangles (CGF and DEB), total area = 2 * 4.5 = 9 cm²
- Given: Length = 6.5 cm, Width = 1 cm
- Area = Length * Width = 6.5 * 1 = 6.5 cm²
- Given: Top base (PQ) = 1 cm, Bottom base (RS) = 2 cm, Height = 1 cm
- Area = 1/2 * (Base1 + Base2) * Height = 1/2 * (1 + 2) * 1 = 1.5 cm²
- Total Area = Area of Triangle ABC + Area of Triangle DEB + Area of Triangle CGF + Area of Rectangle BCQP + Area of Trapezium PQRS
- Total Area = 2.48 cm² + 9 cm² + 6.5 cm² + 1.5 cm² = 19.48 cm²
The total area of the paper used is 19.48 cm².
Topper's Solved these Questions
Similar Questions
Radha made a picture of an aeroplane with coloured paper as shown in figure. Find the total area of the paper used.
View SolutionFour equal circles are described about the four corners of a square so that each touches two of the others as shown in Fig. 15.38. Find the area of the shaded region, each side of the square measuring 14 cm. (FIGURE)
View SolutionA brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire is also used in making 5 diameters which divide the circle into 10 equal sectors as shown in Fig. 12.12. Find :
(i) the total length of the silver wire required.
(ii) the area of each sector of the brooch.
View SolutionA brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire also used in making 5 diameters which divide the circle into 10 equal sectors as shown in Fig. 15.16. Find: (i) the total length of the silver wire required (ii) the area of each sector of the brooch.
View SolutionA loop, carring a current i, lying in the plane of the paper, is in the field of a long straight wire with current i0 (inward) as shown in Fig. Find the torque acting on the loop.

View SolutionIn a circular table cover of radius 32 cm, a design (shade) is formed leaving an equilateral traingle ABC in the middle as shown in the adjacent figure.Find the area of the shaded region.
View SolutionFrom each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in Fig. 12.23. Find the area of the remaining portion of the square.
View SolutionFrom each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in Fig. 12.23. Find the area of the remaining portion of the square.
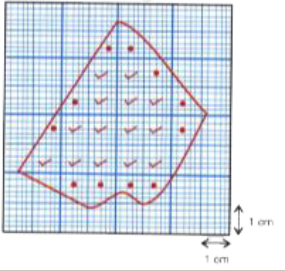
View SolutionThe boundary line of an irregular lamina, on a graph paper is shown in Fig. Find the approximate area of the lamina. In Fig. , the number of complete squares = 14
The number of squares more than half = 11
View SolutionAn aquarium is in the form of a cuboid whose external measures are 80cm×30cm×40cm. The base, side faces and back face are to be covered with a coloured paper. Find the area of the paper needed?
View Solution
