A firm manufactures two types of products, A and B, and sells them at a profil of 3 per unit to type B product and 5 perunit of type A product. Both product is processed on two machines M1and M2 One unit of type A requires one minute of processing time on M1 and two minutes of processing time on M2i whereas one unit of type B requires one minute of processing time on M1 and one minute on M Machines sells them at a profit of 5 per unit of type A and M, and M, are respectively available for at most 5 hours and 6 hours in a day. Find out how many units of each type of product the firn should produce a day in order to maximize the profit. Solve the problem graphically [CHSE 2000]
To solve the problem of maximizing the profit for the firm manufacturing products A and B, we need to follow these steps:
Step 1: Define the Variables
Let:
- x = number of units of product A produced
- y = number of units of product B produced
Step 2: Define the Objective Function
The profit from product A is 5 per unit and from product B is 3 per unit. Therefore, the objective function to maximize is:
Z=5x+3y
Step 3: Define the Constraints
We have two machines, M1 and M2, with the following constraints based on the processing times:
1. Machine M1:
- Each unit of A requires 1 minute and each unit of B requires 1 minute.
- Total available time for M1 = 5 hours = 300 minutes.
x+y≤300
2. Machine M2:
- Each unit of A requires 2 minutes and each unit of B requires 1 minute.
- Total available time for M2 = 6 hours = 360 minutes.
2x+y≤360
3. Non-negativity Constraints:
x≥0,y≥0
Step 4: Graph the Constraints
To graph the constraints, we will convert the inequalities into equations:
1. From x+y=300:
- If x=0, then y=300 (point A).
- If y=0, then x=300 (point B).
2. From 2x+y=360:
- If x=0, then y=360 (point C).
- If y=0, then x=180 (point D).
Now, we plot these lines on a graph and identify the feasible region formed by these constraints.
Step 5: Identify the Feasible Region
The feasible region is the area where all the constraints overlap. It is bounded by the lines we plotted and the axes.
Step 6: Find the Corner Points
The corner points of the feasible region can be found by solving the equations of the lines:
1. Intersection of x+y=300 and 2x+y=360:
x+y=300(1)2x+y=360(2)
Subtract (1) from (2):
2x+y−(x+y)=360−300x=60
Substitute x=60 into (1):
60+y=300y=240
So one corner point is (60,240).
2. Intersection of x+y=300 and x=0:
(0,300)
3. Intersection of 2x+y=360 and y=0:
2x=360x=180
So another corner point is (180,0).
4. Intersection of x=0 and y=0:
(0,0)
Step 7: Evaluate the Objective Function at Each Corner Point
Now we evaluate Z=5x+3y at each corner point:
1. (60,240):
Z=5(60)+3(240)=300+720=1020
2. (0,300):
Z=5(0)+3(300)=0+900=900
3. (180,0):
Z=5(180)+3(0)=900+0=900
4. (0,0):
Z=5(0)+3(0)=0
Step 8: Determine the Maximum Profit
The maximum profit occurs at the point (60,240) with a profit of 1020.
Conclusion
The firm should produce:
- 60 units of product A
- 240 units of product B
Let:
- x = number of units of product A produced
- y = number of units of product B produced
The profit from product A is 5 per unit and from product B is 3 per unit. Therefore, the objective function to maximize is:
Z=5x+3y
We have two machines, M1 and M2, with the following constraints based on the processing times:
- Each unit of A requires 1 minute and each unit of B requires 1 minute.
- Total available time for M1 = 5 hours = 300 minutes.
x+y≤300
- Each unit of A requires 2 minutes and each unit of B requires 1 minute.
- Total available time for M2 = 6 hours = 360 minutes.
2x+y≤360
x≥0,y≥0
To graph the constraints, we will convert the inequalities into equations:
- If x=0, then y=300 (point A).
- If y=0, then x=300 (point B).
- If x=0, then y=360 (point C).
- If y=0, then x=180 (point D).
The feasible region is the area where all the constraints overlap. It is bounded by the lines we plotted and the axes.
The corner points of the feasible region can be found by solving the equations of the lines:
1. Intersection of x+y=300 and 2x+y=360:
x+y=300(1)2x+y=360(2)
Subtract (1) from (2):
2x+y−(x+y)=360−300x=60
Substitute x=60 into (1):
60+y=300y=240
So one corner point is (60,240).
(0,300)
2x=360x=180
So another corner point is (180,0).
(0,0)
Now we evaluate Z=5x+3y at each corner point:
1. (60,240):
Z=5(60)+3(240)=300+720=1020
2. (0,300):
Z=5(0)+3(300)=0+900=900
3. (180,0):
Z=5(180)+3(0)=900+0=900
4. (0,0):
Z=5(0)+3(0)=0
The maximum profit occurs at the point (60,240) with a profit of 1020.
The firm should produce:
- 60 units of product A
- 240 units of product B
Topper's Solved these Questions
Similar Questions
Evaluate ∫cot2xdx
View SolutionFind the coordinates of a point on the parabola y=x2+7x+2 which is closest to the straight line y=3x−3.
View SolutionA manufacturer produces two products A and B. Both the products are processed on two different machines. The available capacity of first machine is 12 hours and that of second machine is 9 hours per day. Each unit of product A requires 3 hours on both machines and each unit of product B requires 2 hours on first machine and 1 hour on second machine. Each unit of product A is sold at Rs. 7 profit and that of B at a profit of Rs. 4. Find the production level per day for maximum profit graphically.
View SolutionA firm manufactures two types of products, A and B, and sells them at a profil of 3 per unit to type B product and 5 perunit of type A product. Both product is processed on two machines M1and M2 One unit of type A requires one minute of processing time on M1 and two minutes of processing time on M2i whereas one unit of type B requires one minute of processing time on M1 and one minute on M Machines sells them at a profit of 5 per unit of type A and M, and M, are respectively available for at most 5 hours and 6 hours in a day. Find out how many units of each type of product the firn should produce a day in order to maximize the profit. Solve the problem graphically [CHSE 2000]
View SolutionA company manufacturers two types of products P and Q. The product P requires 5 minutes each for cutting and 10 minutes each for assembling. The product Q requires 8 minutes each for cutting and 8 minutes each for assembling. There are 3 hours 20 minutes availabe for cutting and 4 hours for assembling. The profit is 50 paise each for type P and 60 paise each for type Q. How many products of each type should the company manufacture in order to maximize the profit?
View SolutionA firm manufactures two types of products, A and B, and sells them at a profit of Rs.5 per unit of type A and Rs.3 per unit of type B.Each product is processed on two machines, M1andM2. One unit of type A requires one minute of processing time on M1 and one minute on M2. Machines M1andM2. are respectively available for at most 5 hours and 6 hours in a day. Find out how many units of each type of product the fiem should produce a day in order to maximize the profit. Solve the problem graphically.
View Solutionएक कम्पनी दो प्रकार के उत्पाद A तथा B बनाती है तथा A को 5 रुपये प्रति इकाई तथा B को 3 रुपये प्रति इकाई पर बेचती हैं । प्रत्येक उत्पाद दो मशीनों M1 तथा M2 से बनता हैं । A के 1 इकाई को बनाने में 1 मिनट मशीन M1 तथा 2 मिनट मशीन M2 पर लगते हैं जबकि B के 1 इकाई को बनाने में 1 मिनट मशीन M1 तथा 1 मिनट मशीन M2 पर लगते हैं । मशीन M1 व M2 क्रमशः 5 घण्टे तथा 6 घण्टे एक दिन में काम करती हैं तो 1 दिन में अधिकतम लाभ पाने के लिए उत्पाद A तथा B की कितनी इकाइयाँ बन सकती हैं ? इसे आलेखीय विधि व्दारा हल करो ।
View Solutionएक कंपनी A और B दो प्रकार के खिलौनों का उत्पादन करती है। A प्रकार के प्रति खिलौना के निर्माण में 5 मिनट काटने और 10 मिनट जोड़ने में लगते हैं। B प्रकार के प्रति खिलौना के लिए 8 मिनट काटने और 8 मिनट जोड़ने में लगते हैं। काटने के लिए कुल समय 3 घंटे जोड़ने के लिए 4 घंटे प्रतिदिन उपलब्ध हैं। A प्रकार के प्रति खिलौना पर 50 रू0 और B प्रकार के प्रति खिलौना पर 60 रू0 का लाभ होता है। ज्ञात करें कि अधिकतम लाभ के लिए प्रत्येक प्रकार के कितने-कितने खिलौने कंपनी को प्रतिदिन निर्माण करना चाहिए।
View SolutionA company manufactures two types of chemicals A and B. Each chemical requires the types of raw materials P and Q. The table below shows number of units of P and Q required to manufacture one unit of A and one unit of B and the total availability of P and Q.
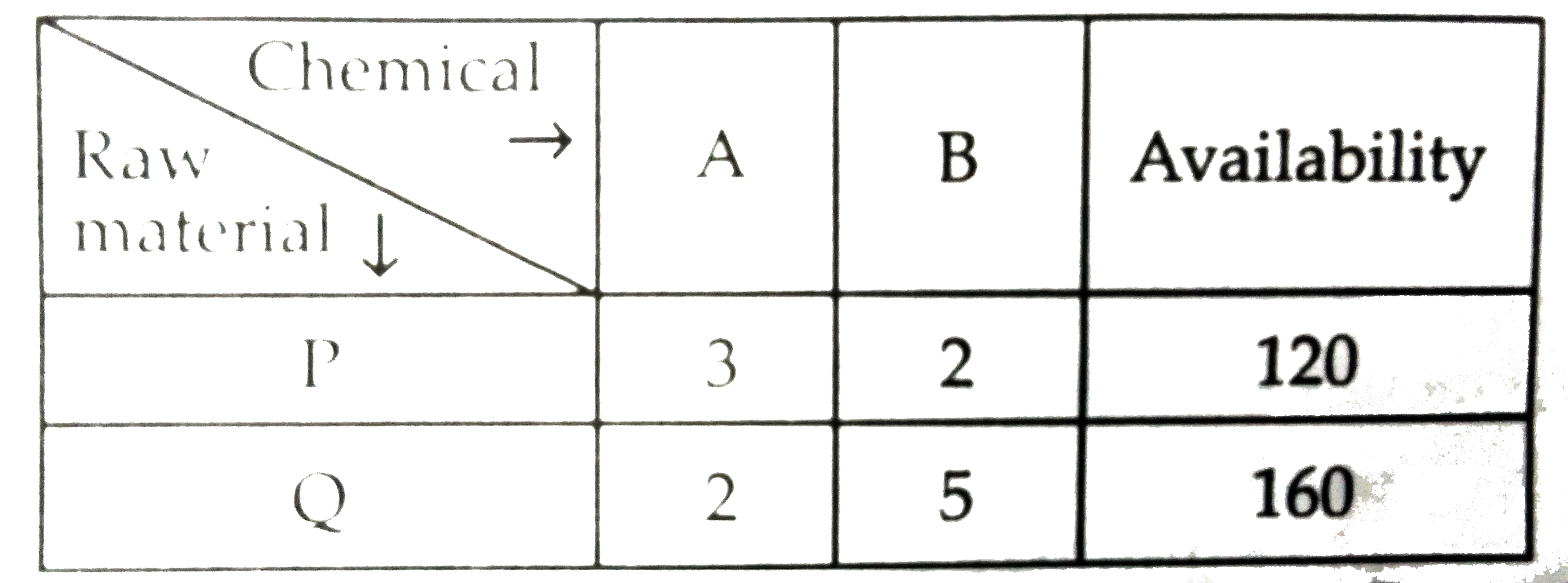
The company gets profit of Rs. 350 and RS.400 by selling one unit of A and one unit of B respectively. If the entire production of A and B is sold, then formulate the problem as LPP.
View Solutionएक फर्म A और B दो प्रकार के उत्पाद बनाती है और उन्हें क्रमशः प्रति इकाई ₹6 तथा ₹ 3 के लाभ पर बेचती है। प्रत्येक उत्पाद दो मशीनों M1 और M2 से गुजरता है। A उत्पाद को M1 और M2 पर क्रमश: 1 मिनट और 2 मिनट लगते हैं जबकि B उत्पाद को M1 और M2 पर क्रमश: 1 मिनट और 1 मिनट लगते हैं। मशीन M1 और M2 प्रति दिवस क्रमशः 5 घण्टे तथा 6 घण्टे के लिए उपलब्ध है। अधिकतम लाभ के लिए फर्म को प्रतिदिन दोनों प्रकारों की कितनी संख्या उत्पादित करना चाहिए। ग्राफीय विधि से हल कीजिए।
View SolutionA firm manufactures two types of products A and B and sells tehm at a profit of rs 5 per unit of type A and Rs 3 per unit to type B. One unti of type A requires one minute of process ign time on M1 and two minutes of processing time on M2 find out how many units of each type of procuct the firm should producve a day in order to maximize the profit solve the problem graphically
View SolutionA manufacturer produces two products A and B. Both the products are processed on two different machines. The available capacity of first machine is 12 hours and that of second machine is 9 hours per day. Each unit of product A requires 3 hours on both machines and each unit of product B requires 2 hours on first machine and 1 hour on second machine. Each unit of product A is sold at Rs. 7 profit and that of B at a profit of Rs. 4. Find the production level per day for maximum profit graphically.
View SolutionA firm manufactures two products A and B. Each product is processed on two machines M1andM2 . Product A requires 4 minutes of processing time on M1 and 8 min. on M2 ; product B requires 4 minutes on M1 and 4 min. on M2 . The machine M1 is available for not more than 8 hrs 20 min. while machine M2 is available for 10 hrs. during any working day. The products A and B are sold at a profit of Rs. 3 and Rs. 4 respectively. Formulate the problem as a linear programming problem and find how many products of each type should be produced by the firm each day in order to get maximum profit.
View SolutionA manufacturer produces two products A and B. Both the products are processed on two different machines. The available capacity of first machine is 12 hours and that of second machine is 9 hours per day. Each unit of product A requires 3 hours on both machines and each unit of product B requires 2 hours on first machine and 1 hour on second machine. Each unit of product A is sold at ₹ 7 profit and that of B at a profit oft ₹ 4. Find the production level per day for maximum profit graphically.
View SolutionA firm manufactures two types of products A and B and sells them at a profit of Rs. 5 per unit of type A and Rs. 3 per unit of type B . Each product is processed on two machines M_1 and M_2 . One unit of type A requires one minute of processing time, on, M_1 and two minutes of processing time on M_2 whereas one unit of type B requires one minute of processing time on M_1 and one minute of M_2 . Machines M_1 and M_2 are respectively available for at most 5 hours and 6 hours a day. Find how many, units of each type of product should the firm produce a day, in order, to maximise the profit. Solve thè problem graphically.
View Solution
