If the probability of a defective bolt is 0.1, find the i. mean and ii. standard deviation for the distribution of bolts in a total of 400 bolts.
To solve the problem, we need to find the mean and standard deviation of the distribution of defective bolts among a total of 400 bolts, given that the probability of a defective bolt is 0.1.
1. Identify the parameters:
- Total number of bolts (n) = 400
- Probability of a defective bolt (p) = 0.1
- Probability of a non-defective bolt (q) = 1 - p = 1 - 0.1 = 0.9
2. Calculate the Mean (μ):
- The formula for the mean (μ) in a binomial distribution is given by:
μ=n×p
- Substituting the values:
μ=400×0.1=40
3. Calculate the Variance (σ²):
- The formula for the variance (σ²) in a binomial distribution is given by:
σ2=n×p×q
- Substituting the values:
σ2=400×0.1×0.9=400×0.09=36
4. Calculate the Standard Deviation (σ):
- The standard deviation (σ) is the square root of the variance:
σ=√σ2=√36=6
Final Answers:
- Mean (μ) = 40
- Standard Deviation (σ) = 6
- Total number of bolts (n) = 400
- Probability of a defective bolt (p) = 0.1
- Probability of a non-defective bolt (q) = 1 - p = 1 - 0.1 = 0.9
- The formula for the mean (μ) in a binomial distribution is given by:
μ=n×p
- Substituting the values:
μ=400×0.1=40
- The formula for the variance (σ²) in a binomial distribution is given by:
σ2=n×p×q
- Substituting the values:
σ2=400×0.1×0.9=400×0.09=36
- The standard deviation (σ) is the square root of the variance:
σ=√σ2=√36=6
- Mean (μ) = 40
- Standard Deviation (σ) = 6
Topper's Solved these Questions
Similar Questions
Find the variance and standard deviation for the following distribution.

View SolutionFind the variance and standard deviation of the following distribution

View SolutionFind the mean and the standard deviation from the following:
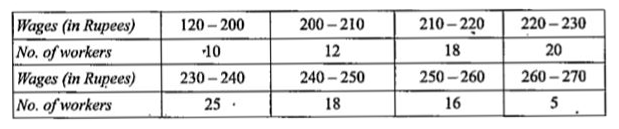
View SolutionFind the mean and standard deviation from the following data :

View SolutionFind the mean and standard deviation of the following frequency distribution :
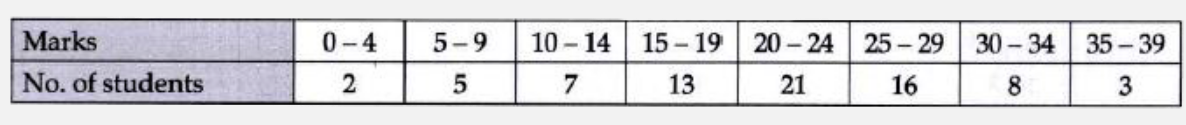
View SolutionFind the mean and standard deviation using short cut method

View SolutionFind the standard deviation of the following distribution :
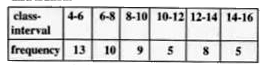
View SolutionFind out the standard deviation from the following distribution table:
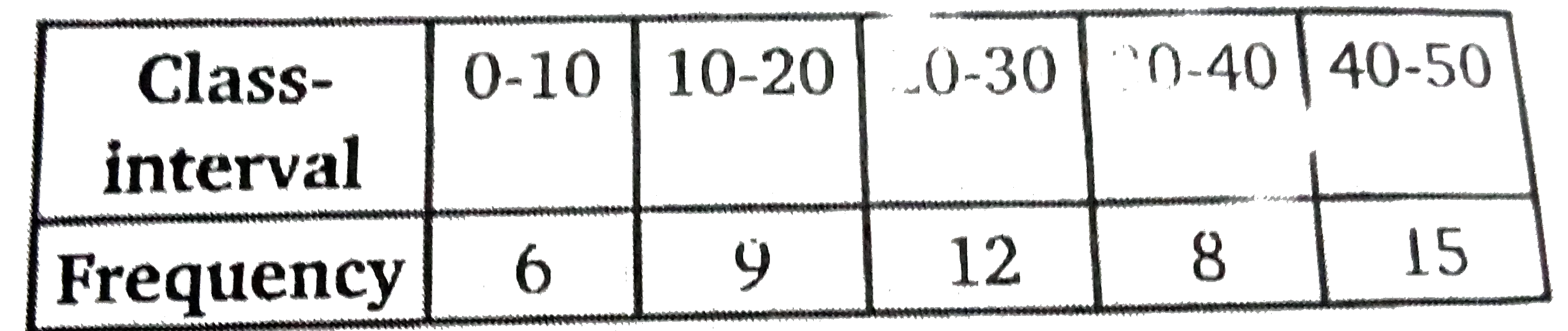
View SolutionIn a binomial distribution the mean is 12 and the standard deviation is √3. Then the distribution is
View SolutionIn a group of 200 items, if the probability of getting a defective item is 0.2, write the mean of the distribution.
View Solution