Find the variance and standard deviation for the following data: 4,6,10,12,14,18,20
To find the variance and standard deviation for the given data set: 4, 6, 10, 12, 14, 18, 20, we will follow these steps:
Step 1: Find the Mean
The mean (average) is calculated by adding all the data points and dividing by the number of data points.
Mean=Sum of all data pointsNumber of data points
Calculating the sum:
4+6+10+12+14+18+20=84
The number of data points is 7.
Mean=847=12
Step 2: Calculate the Deviations from the Mean
Next, we find the deviation of each data point from the mean and then square each deviation.
4−12=−8⇒(−8)2=646−12=−6⇒(−6)2=3610−12=−2⇒(−2)2=412−12=0⇒(0)2=014−12=2⇒(2)2=418−12=6⇒(6)2=3620−12=8⇒(8)2=64
Step 3: Sum of Squared Deviations
Now, we sum all the squared deviations:
64+36+4+0+4+36+64=208
Step 4: Calculate the Variance
The variance is calculated by dividing the sum of squared deviations by the number of data points.
Variance=Sum of squared deviationsNumber of data points=2087≈29.71
Step 5: Calculate the Standard Deviation
The standard deviation is the square root of the variance.
Standard Deviation=√Variance=√29.71≈5.45
Final Answers
- Variance: ≈29.71
- Standard Deviation: ≈5.45
The mean (average) is calculated by adding all the data points and dividing by the number of data points.
Next, we find the deviation of each data point from the mean and then square each deviation.
Now, we sum all the squared deviations:
The variance is calculated by dividing the sum of squared deviations by the number of data points.
The standard deviation is the square root of the variance.
- Variance: ≈29.71
- Standard Deviation: ≈5.45
Topper's Solved these Questions
Similar Questions
Find the variance and standard deviation of the following data:

View SolutionFind the variance and standard deviation from the following data:

View SolutionFind the mean, variance and standard deviation for the following data: 6,7,10,12,13,4,8,12
View SolutionFind the mean and standard deviation from the following data :

View SolutionFind the variance and standard deviation of the following data :
(i) 6,7,10,12,13,4,8,12
(ii) 5,12,3,18,6,8,2,10
(iii) 350,361, 370, 373, 376, 379, 385, 387, 394, 395
View SolutionFind the standard deviation for the following data

View SolutionFind the standard deviation for the following data
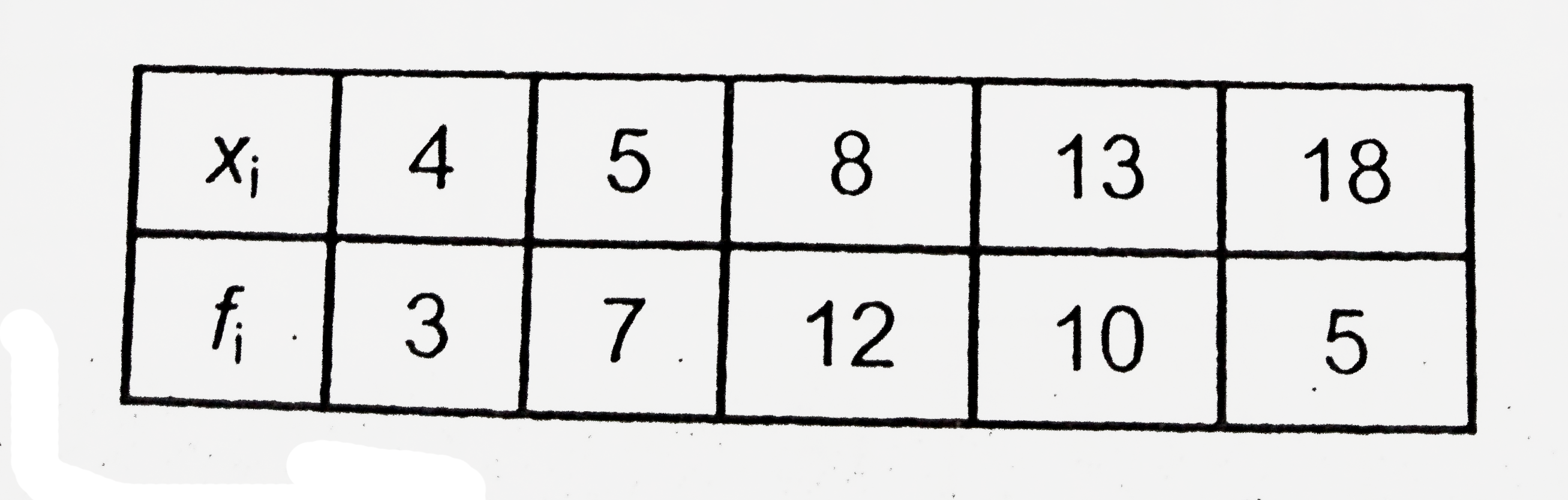
View SolutionFind the mean variance and standard deviation for the following data: 2,4,5,6,8,17
View SolutionFind the standard deviation from the following data:
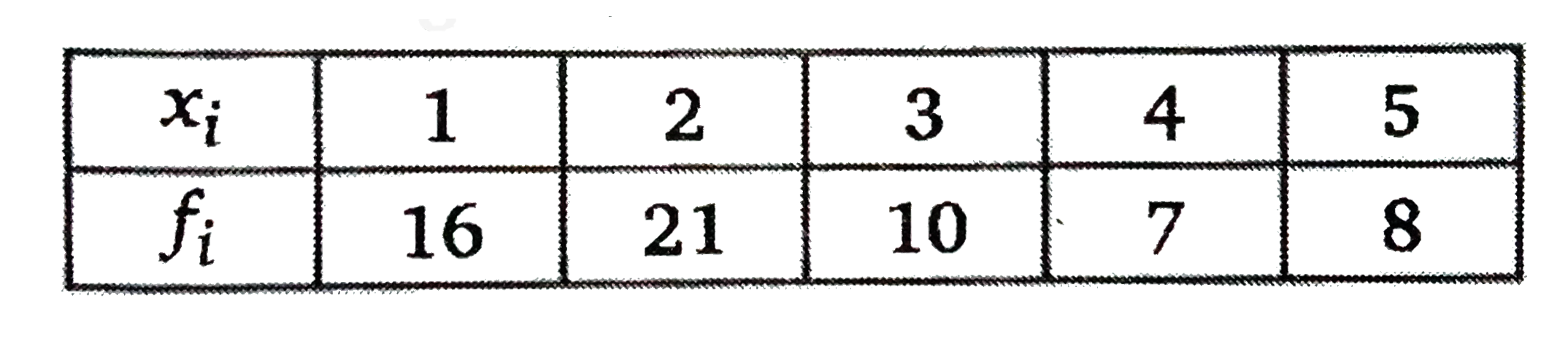
View SolutionFind the variance and standard deviation of the following data 5,12,3,18,6,8,2,10
View Solution
