In Fig. state if PQ∣∣EF
To determine if line segment PQ is parallel to line segment EF, we will use the Basic Proportionality Theorem (also known as Thales' theorem). According to this theorem, if a line is drawn parallel to one side of a triangle, it divides the other two sides in the same ratio.
1. Identify the given lengths:
- EP = 3 cm
- PD = 3.9 cm
- FQ = 2.4 cm
- QD = 3.6 cm
2. Set up the ratios according to the Basic Proportionality Theorem:
- We need to check if the ratio EP/PD is equal to the ratio FQ/QD.
- This means we need to calculate:
EPPD=33.9
and
FQQD=2.43.6
3. Calculate the first ratio:
- Simplifying 33.9:
33.9=3÷33.9÷3=11.3≈0.769
4. Calculate the second ratio:
- Simplifying 2.43.6:
2.43.6=2.4÷2.43.6÷2.4=11.5≈0.667
5. Compare the two ratios:
- We have:
EPPD≈0.769andFQQD≈0.667
- Since 0.769≠0.667, we conclude that:
EPPD≠FQQD
6. Conclusion:
- Since the ratios are not equal, by the Basic Proportionality Theorem, we can conclude that PQ is not parallel to EF.
- EP = 3 cm
- PD = 3.9 cm
- FQ = 2.4 cm
- QD = 3.6 cm
- We need to check if the ratio EP/PD is equal to the ratio FQ/QD.
- This means we need to calculate:
EPPD=33.9
and
FQQD=2.43.6
- Simplifying 33.9:
33.9=3÷33.9÷3=11.3≈0.769
- Simplifying 2.43.6:
2.43.6=2.4÷2.43.6÷2.4=11.5≈0.667
- We have:
EPPD≈0.769andFQQD≈0.667
- Since 0.769≠0.667, we conclude that:
EPPD≠FQQD
- Since the ratios are not equal, by the Basic Proportionality Theorem, we can conclude that PQ is not parallel to EF.
Topper's Solved these Questions
Similar Questions
In Fig. (i) and (ii), PQ∣∣BC . Find QC in (i) and AQ in (ii) (FIGURE)
View SolutionIn Fig. , the side QR of △ PQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR=12∠QPR.

View SolutionIn Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
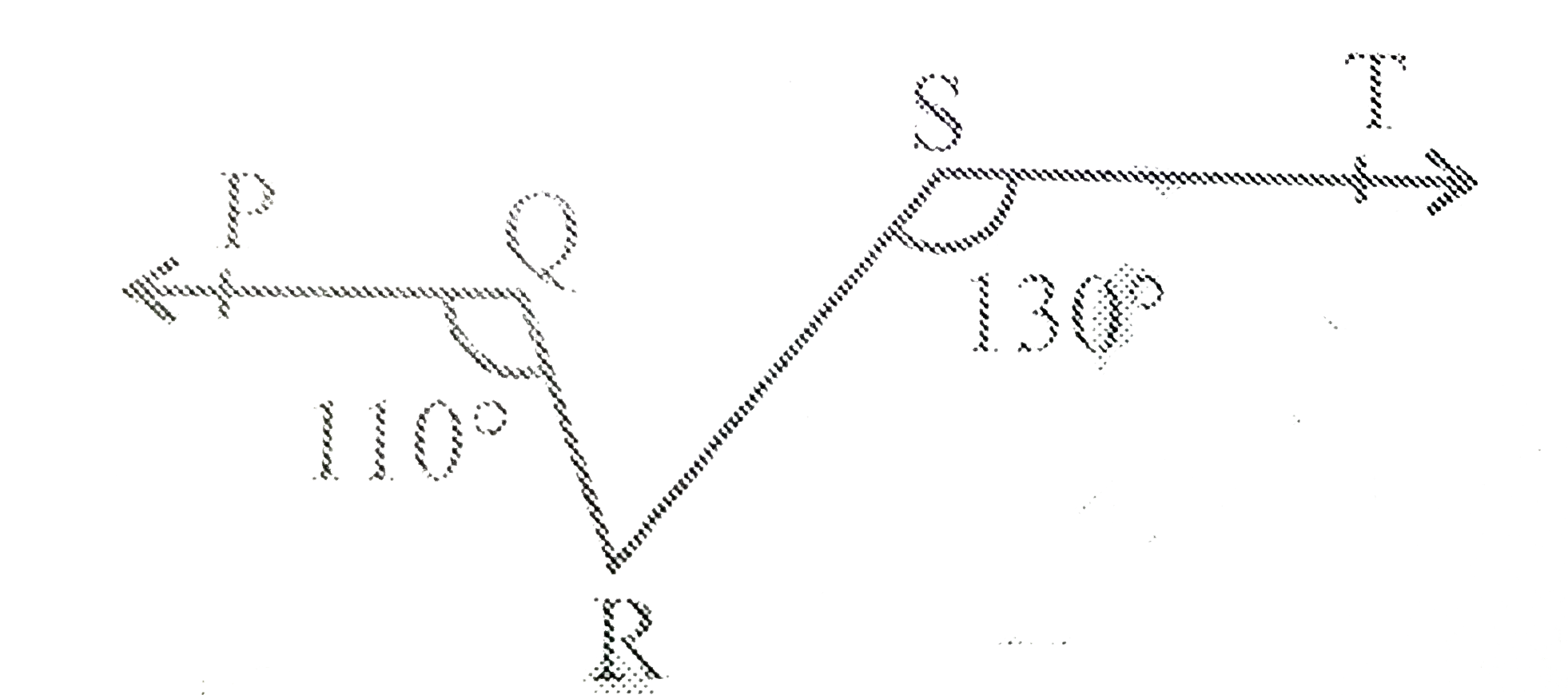
View SolutionIn Fig. 4.90, if PS∣∣QR , prove that POS~ROQ . (FIGURE)
View SolutionIn Fig.99, ABCD and EF is a transversal intersecting AB and CD at P and Q respectively. The measure of ∠DPQ is (a) (b)(c)(d)(e)100(f)0(g)(h)(i) (j) (b) (k)(l)(m)(n)80(o)0(p)(q)(r) (s) (c) (d)(e)(f)(g)110(h)0(i)(j)(k) (l) (d) (m)(n)(o)(p)70(q)0(r)(s)(t) (u)
View SolutionIn Fig. 6.43, if PQ⊥PS,PQ∣∣SR,∠SQR=28∘ and ∠QRT=65∘, then find the values of x and y.

View SolutionIn Fig. PQ is tangent at a point R of the circle with centre O . If ∠TRQ=30o , find ∠PRS . (FIGURE)
View SolutionIn Fig. if PQ∣∣BC and PR∣∣CD . Prove that (i) ARAD=AQAB (ii) QBAQ=DRAR .
View SolutionIn Figure ABCD and AEFD are two parallelograms. Prove that: PE=FQ
View SolutionIn Fig. 4.246, if PBCF and DPEF , then ADDE= (FIGURE) 34 (b) 13 (c) 14 (d) 23
View Solution
