State which pairs of triangles in Figure are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form:
To determine which pairs of triangles in the given figures are similar, we will follow these steps:
1. Identify the Triangles:
We have two triangles: Triangle DEF and Triangle PQR.
2. Calculate the Angles of Triangle DEF:
- Given angles:
- Angle D = 80°
- Angle E = 70°
- To find Angle F:
Angle F=180°−(Angle D+Angle E)=180°−(80°+70°)=180°−150°=30°
- So, Angle F = 30°.
3. Calculate the Angles of Triangle PQR:
- Given angles:
- Angle Q = 80°
- Angle R = 30°
- To find Angle P:
Angle P=180°−(Angle Q+Angle R)=180°−(80°+30°)=180°−110°=70°
- So, Angle P = 70°.
4. List the Angles of Both Triangles:
- Triangle DEF:
- Angle D = 80°
- Angle E = 70°
- Angle F = 30°
- Triangle PQR:
- Angle P = 70°
- Angle Q = 80°
- Angle R = 30°
5. Compare the Angles:
- We see that:
- Angle D = Angle P = 70°
- Angle E = Angle Q = 80°
- Angle F = Angle R = 30°
6. Apply the Similarity Criterion:
- Since all corresponding angles of Triangle DEF and Triangle PQR are equal, we can use the Angle-Angle-Angle (AAA) similarity criterion.
7. State the Similarity:
- Therefore, we conclude that:
△DEF∼△PQR(by AAA criterion)
Final Answer:
The pairs of similar triangles are:
△DEF∼△PQR(by AAA criterion)
We have two triangles: Triangle DEF and Triangle PQR.
- Given angles:
- Angle D = 80°
- Angle E = 70°
- To find Angle F:
Angle F=180°−(Angle D+Angle E)=180°−(80°+70°)=180°−150°=30°
- So, Angle F = 30°.
- Given angles:
- Angle Q = 80°
- Angle R = 30°
- To find Angle P:
Angle P=180°−(Angle Q+Angle R)=180°−(80°+30°)=180°−110°=70°
- So, Angle P = 70°.
- Triangle DEF:
- Angle D = 80°
- Angle E = 70°
- Angle F = 30°
- Triangle PQR:
- Angle P = 70°
- Angle Q = 80°
- Angle R = 30°
- We see that:
- Angle D = Angle P = 70°
- Angle E = Angle Q = 80°
- Angle F = Angle R = 30°
- Since all corresponding angles of Triangle DEF and Triangle PQR are equal, we can use the Angle-Angle-Angle (AAA) similarity criterion.
- Therefore, we conclude that:
△DEF∼△PQR(by AAA criterion)
The pairs of similar triangles are:
△DEF∼△PQR(by AAA criterion)
Topper's Solved these Questions
Similar Questions
State, true or false :
All isosceles triangles are similar.
View SolutionState, true or false :
All equiangular triangles are similar.
View SolutionThe triangles shown in adjoining figures are similar. Find the values of a and b.
View SolutionIn which pair of species both species do have the similar geometry ? .
View SolutionWhich of pair is not having similar types of roots ?
View SolutionState, true or false :
Two isosceles-right triangles are similar.
View Solution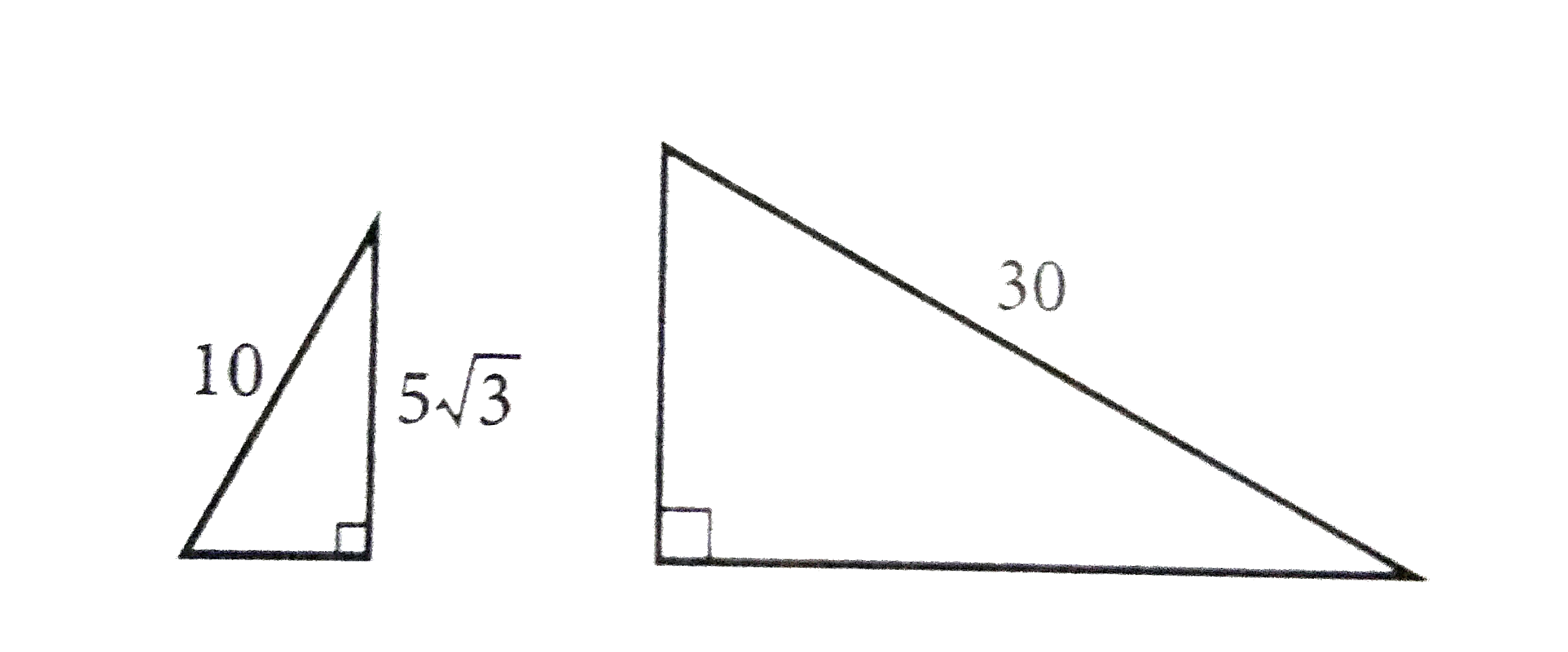
If the right triangle in the figure shown are similar triangle, what is the length of the sorteer leg of the larger triangle?
View SolutionWhich of the following pairs does not have similar dimensions ?
View SolutionWhich pair of elements of atomic numbers given below will have similar chemical properties
View Solution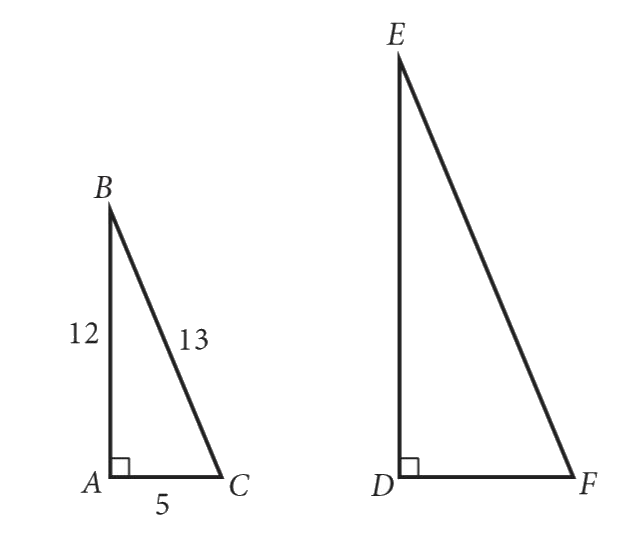
In the figure above, triangle ABC is similar to triangle DEF . What is the value of cos(E) ?
View Solution
