Question

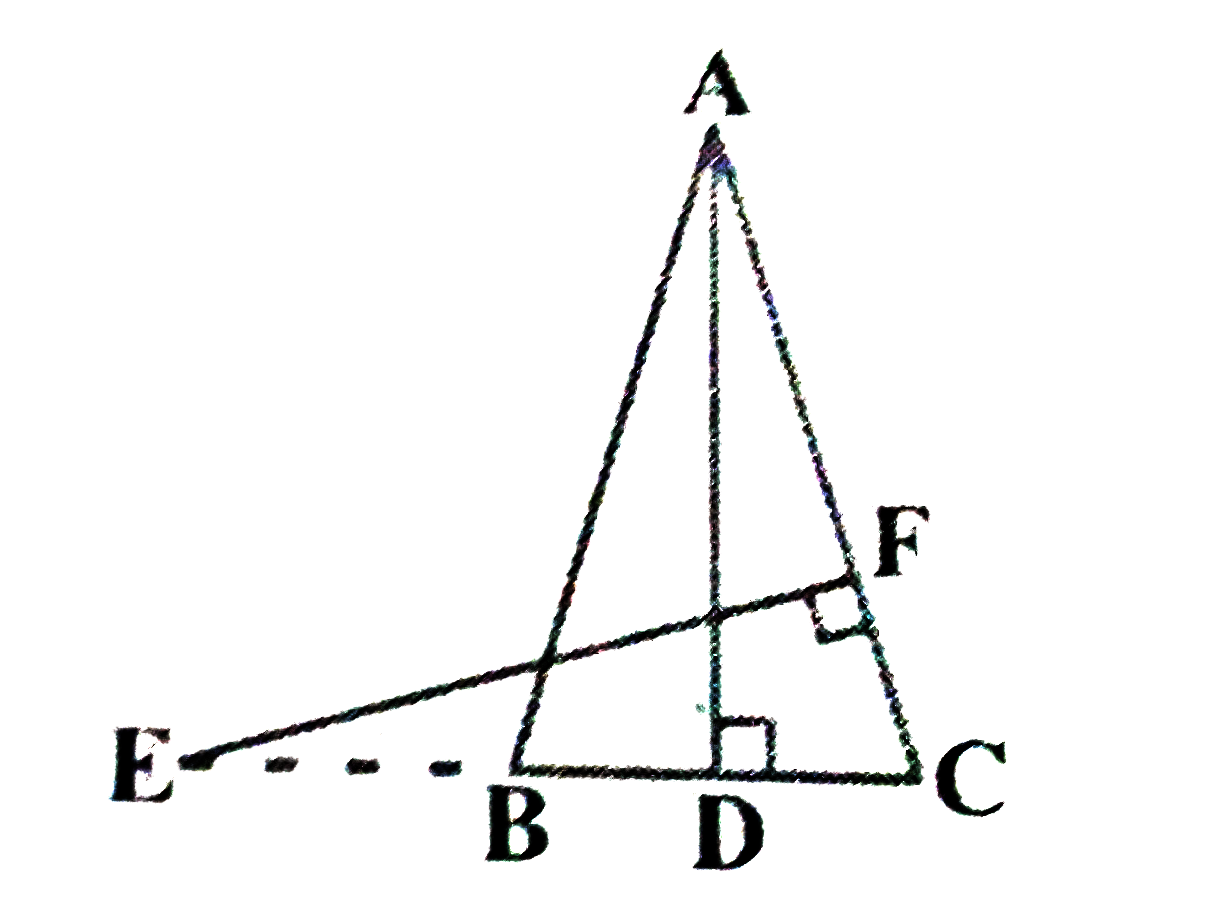
In Fig.
4.124, $E$
is a point
on side $CB$
produced of
an isosceles triangle $ABC$
with $AB=AC$
. If $AD\perp BC$
and $EF\perp AC$
, prove
that (i) △ABD∼△ECF
(ii) $AB\times EF=AD\times EC$
. (FIGURE)

Explanation

To solve the problem step by step, we will prove the two parts as required.

Step 1: Identify the Given Information
We have an isosceles triangle \( ABC \) where \( AB = AC \). Point \( D \) is such that \( AD \perp BC \) and point \( E \) is on the extended line of \( CB \). Line \( EF \) is such that \( EF \perp AC \).

Step 2: Establish Angles
Since \( AB = AC \), by the properties of isosceles triangles, we know that:
\( \angle C = \angle B \)
Also, since \( AD \perp BC \), we have:
\( \angle ADB = 90^\circ \)
And since \( EF \perp AC \), we have:
\( \angle ECF = 90^\circ \)

Step 3: Use Angle-Angle (AA) Criterion for Similarity
Now we will compare triangles \( ABD \) and \( ECF \):
1. We already established that \( \angle ADB = 90^\circ \) and \( \angle ECF = 90^\circ \).
2. We have \( \angle B = \angle C \) (from the isosceles triangle property).

Thus, we can conclude that:
\( \triangle ABD \sim \triangle ECF \quad \text{(by AA criterion)} \)

Step 4: Establish Proportionality of Sides
Since the triangles are similar, the corresponding sides are proportional:
\( \frac{AB}{EC} = \frac{AD}{EF} \)

Step 5: Cross-Multiply to Prove the Second Part
From the proportionality established, we can cross-multiply:
\( AB \cdot EF = AD \cdot EC \)
This is what we needed to prove for the second part.

Conclusion
We have successfully shown that:
(i) \( \triangle ABD \sim \triangle ECF \)
(ii) \( AB \cdot EF = AD \cdot EC \)