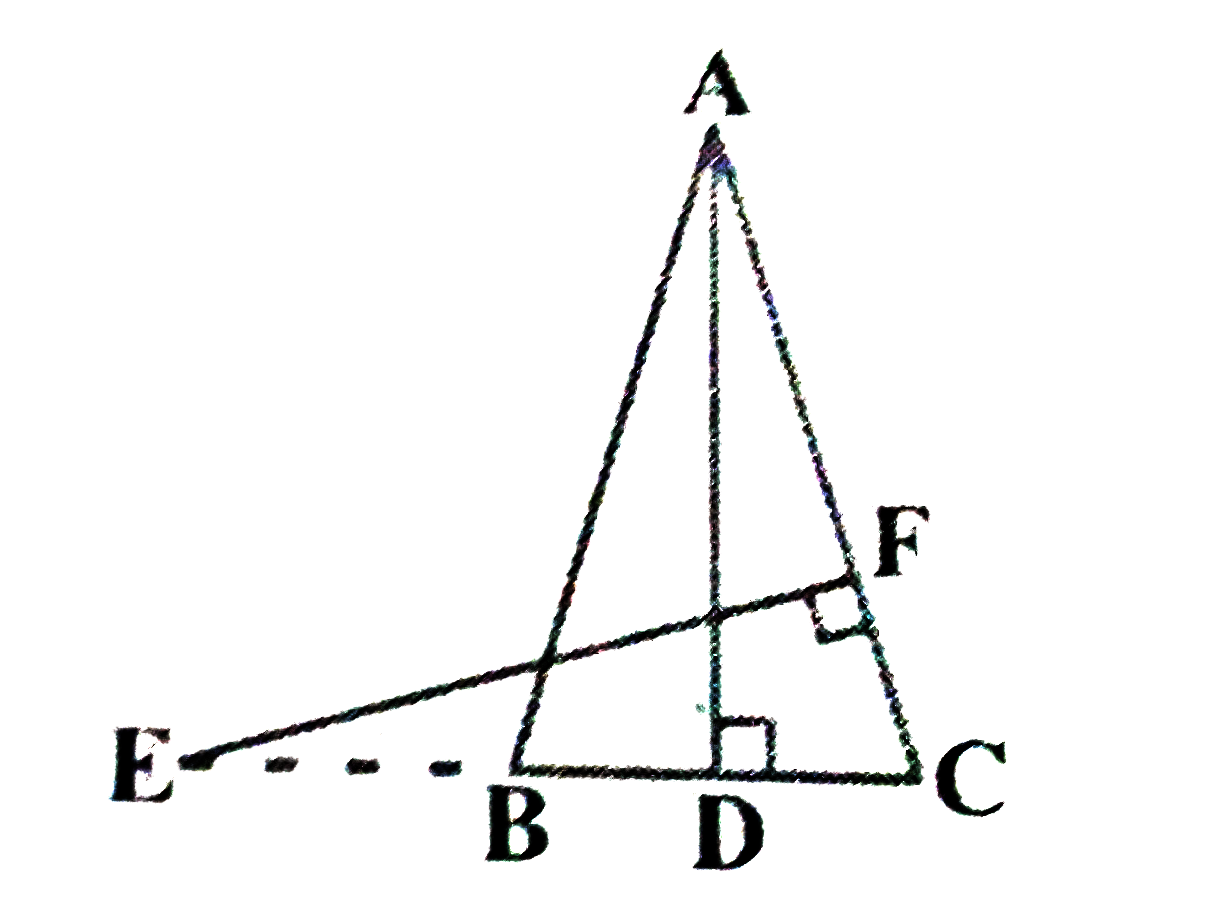
In figureE is a point on side CB produced of an isosceles △ABC with AB=AC. If AD⊥BCandEF⊥AC, prove thatΔABD~ΔECF.
If two angles of one triangle are respectively equal to two angles of another triangle, then the two triangles are similar.
This is referred to as AA similarity criterion for two triangles.
In ΔABD and ΔECF
∠ADB = ∠EFC = 90º [∵AD⊥BC and EF⊥AC]
∠ABD=∠ECF [∵ In ΔABC, AB=AC which signifies ∠ABC=∠ACB as angles opposite to equal sides are equal]
Thus we have ΔABD~ΔECF (AA criterion)
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 6.2
10 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLES
14 Videos
- TRIANGLESBOOK - NCERT ENGLISHCHAPTER - TRIANGLESEXERCISE - Exercise 6.5
17 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.3
9 Videos
Similar Questions
In Fig. 4.124, E is a point on side CB produced of an isosceles triangle ABC with AB=AC . If AD⊥BC and EF⊥AC , prove that (i) △ABD∼△ECF (ii) AB×EF=AD×EC . (FIGURE)
View SolutionIf D is any point on the base BC produced, of an isosceles triangle ABC, prove that AD>AB .
View SolutionIf D is any point on the base BC produced, of an isosceles triangle ABC , prove that AD>AB.
View SolutionIn Figure, AD and BE are respectively altitudes of an isosceles triangle ABC with AC=BC. Prove that AE=BD
View SolutionAD and BE are respectively altitudes of an isosceles triangle ABC with AC=BC . Prove that AE=BD.
View SolutionD is a point on the side BC of a triangle ABC such that ∠ADC=∠BAC. Show that CA2=CB.CD.
View SolutionD and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD=AE . Prove that B,C,D,E are concylic.
View SolutionIn an isosceles triangle ABC with AB=AC, D and E are points on BCsuch that BE=CD(see Fig. 7.29). Show that AD=AE
View SolutionD, E and F are the mid-points of the sides AB, BC and CA of an isosceles triangle ABC in which AB = BC. Prove that ΔDEF is also isosceles.
View SolutionThe incircle of an isoceles triangle ABC, with AB=AC, touches the sides AB,BC and CA at D,E and F respecrively. Prove that E bisects BC.
View Solution
