In Figure, given that △ABC~△PQR and ABCD~ PQRS . Determine the values of x, y, z in each case.
To solve the problem step-by-step, we will first address the similarity of the triangles and then the quadrilaterals.
Step 1: Understanding Triangle Similarity
Given that triangle ABC is similar to triangle PQR, we can use the properties of similar triangles. The sides of similar triangles are in proportion.
Step 2: Setting Up Proportions for Triangle ABC and Triangle PQR
From the similarity of triangles, we can write the following proportions:
ABPQ=BCQR=ACPR
We are given:
- AB=12
- PQ=9
- BC=7
- QR=x
- AC=10
- PR=y
Step 3: Solving for x and y
Using the proportions:
1. From ABPQ=BCQR:
129=7x
Cross-multiplying gives:
12x=9×7
12x=63⟹x=6312=5.25
2. From ABPQ=ACPR:
129=10y
Cross-multiplying gives:
12y=9×10
12y=90⟹y=9012=7.5
Step 4: Understanding Quadrilateral Similarity
Now, we consider the quadrilaterals ABCD and PQRS, which are also similar. The sides of similar quadrilaterals are in proportion.
Step 5: Setting Up Proportions for Quadrilateral ABCD and Quadrilateral PQRS
From the similarity of quadrilaterals, we can write:
ABPQ=BCQR=CDRS=ADPS
We are given:
- AB=16
- PQ=x
- BC=50
- QR=y
- CD=503
- RS=z
- AD=20
- PS=7
Step 6: Solving for x, y, and z
Using the proportions:
1. From ABPQ=ADPS:
16x=207
Cross-multiplying gives:
16×7=20x
112=20x⟹x=11220=5.6
2. From BCQR=ADPS:
50y=207
Cross-multiplying gives:
50×7=20y
350=20y⟹y=35020=17.5
3. From CDRS=ADPS:
503z=207
Cross-multiplying gives:
503×7=20z
3503=20z⟹z=35060=5.83
Final Values
- x=5.25
- y=7.5
- x=5.6
- y=17.5
- z=5.83
Given that triangle ABC is similar to triangle PQR, we can use the properties of similar triangles. The sides of similar triangles are in proportion.
From the similarity of triangles, we can write the following proportions:
ABPQ=BCQR=ACPR
We are given:
- AB=12
- PQ=9
- BC=7
- QR=x
- AC=10
- PR=y
Using the proportions:
1. From ABPQ=BCQR:
129=7x
Cross-multiplying gives:
12x=9×7
12x=63⟹x=6312=5.25
129=10y
Cross-multiplying gives:
12y=9×10
12y=90⟹y=9012=7.5
Now, we consider the quadrilaterals ABCD and PQRS, which are also similar. The sides of similar quadrilaterals are in proportion.
From the similarity of quadrilaterals, we can write:
ABPQ=BCQR=CDRS=ADPS
We are given:
- AB=16
- PQ=x
- BC=50
- QR=y
- CD=503
- RS=z
- AD=20
- PS=7
Using the proportions:
1. From ABPQ=ADPS:
16x=207
Cross-multiplying gives:
16×7=20x
112=20x⟹x=11220=5.6
50y=207
Cross-multiplying gives:
50×7=20y
350=20y⟹y=35020=17.5
503z=207
Cross-multiplying gives:
503×7=20z
3503=20z⟹z=35060=5.83
- x=5.25
- y=7.5
- x=5.6
- y=17.5
- z=5.83
Topper's Solved these Questions
Similar Questions
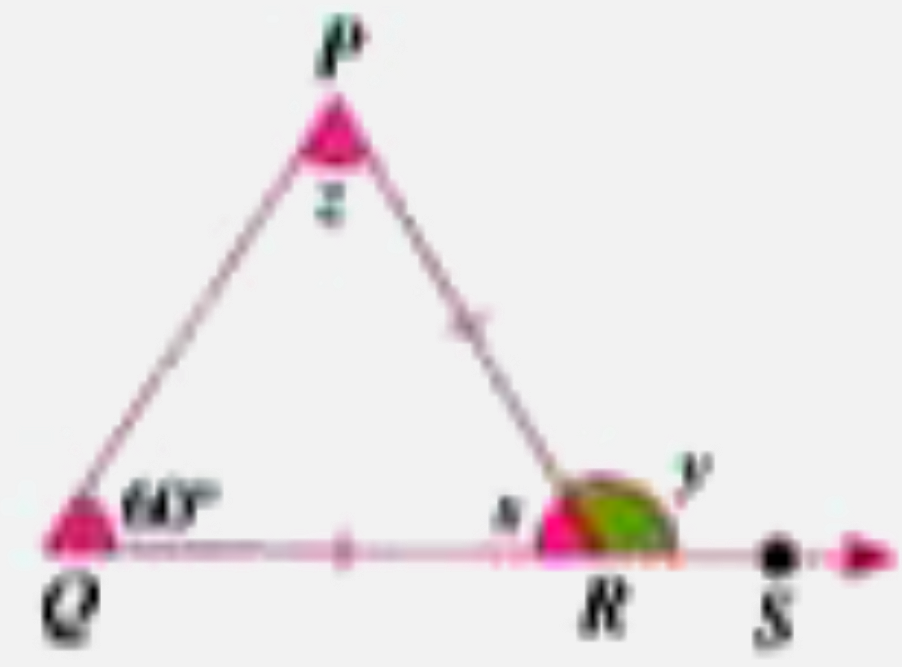
Find the values of x,y and z in the given figure.
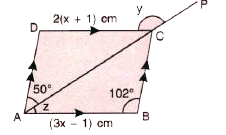
View SolutionIn the given figure, ABCD is a parallelogram. Find the values of x, y and z
View SolutionIn Figure, if PQ⊥PS, PQSR, ∠SQR=280and ∠QRT=650, then find the values of x and y
View SolutionIn the given figure, l || m and p || q. Find the value of x,y,z,t.
View SolutionIn Figure, OP∣∣RS. Determine ∠PQR
View SolutionIn Figure, PQ||AB and PR ||BC . If ∠QPR=1020 , determine ∠ABC. Give reasons.
View SolutionIn Figure, AP||BQ ||CR. Prove that ar (AQC)= ar (PBR)
View SolutionIn Figure, AD=DB and ∠B is a right angle. Determine. (a) sinθ (b) cosθ (c) tanθ (d) sin2θ+cos2θ
View SolutionIn figure, PQ∣∣RS, ∠PAB=70∘and ∠ACS=100∘. Determine ∠ABC, ∠BAC and ∠CAQ
View SolutionIn each of the figures given below, an altitude is drawn to the hypotenuse by a right-angled triangle. The length of different line-segments are marked in each figure. Determine x, y, z in each case.
View Solution
