What is the maximum value of 1cosec θ?
To find the maximum value of 1cscθ, we can follow these steps:
Step 1: Understand the relationship between cosecant and sine
The cosecant function is defined as the reciprocal of the sine function:
cscθ=1sinθ
Step 2: Rewrite the expression
We can rewrite 1cscθ using the relationship from Step 1:
1cscθ=sinθ
Step 3: Determine the maximum value of sine
The sine function, sinθ, has a maximum value of 1. This occurs at specific angles, such as θ=π2+2nπ for any integer n.
Step 4: Conclude the maximum value
Thus, the maximum value of 1cscθ is:
Maximum value=1
Final Answer
The maximum value of 1cscθ is 1.
---
The cosecant function is defined as the reciprocal of the sine function:
cscθ=1sinθ
We can rewrite 1cscθ using the relationship from Step 1:
1cscθ=sinθ
The sine function, sinθ, has a maximum value of 1. This occurs at specific angles, such as θ=π2+2nπ for any integer n.
Thus, the maximum value of 1cscθ is:
Maximum value=1
The maximum value of 1cscθ is 1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
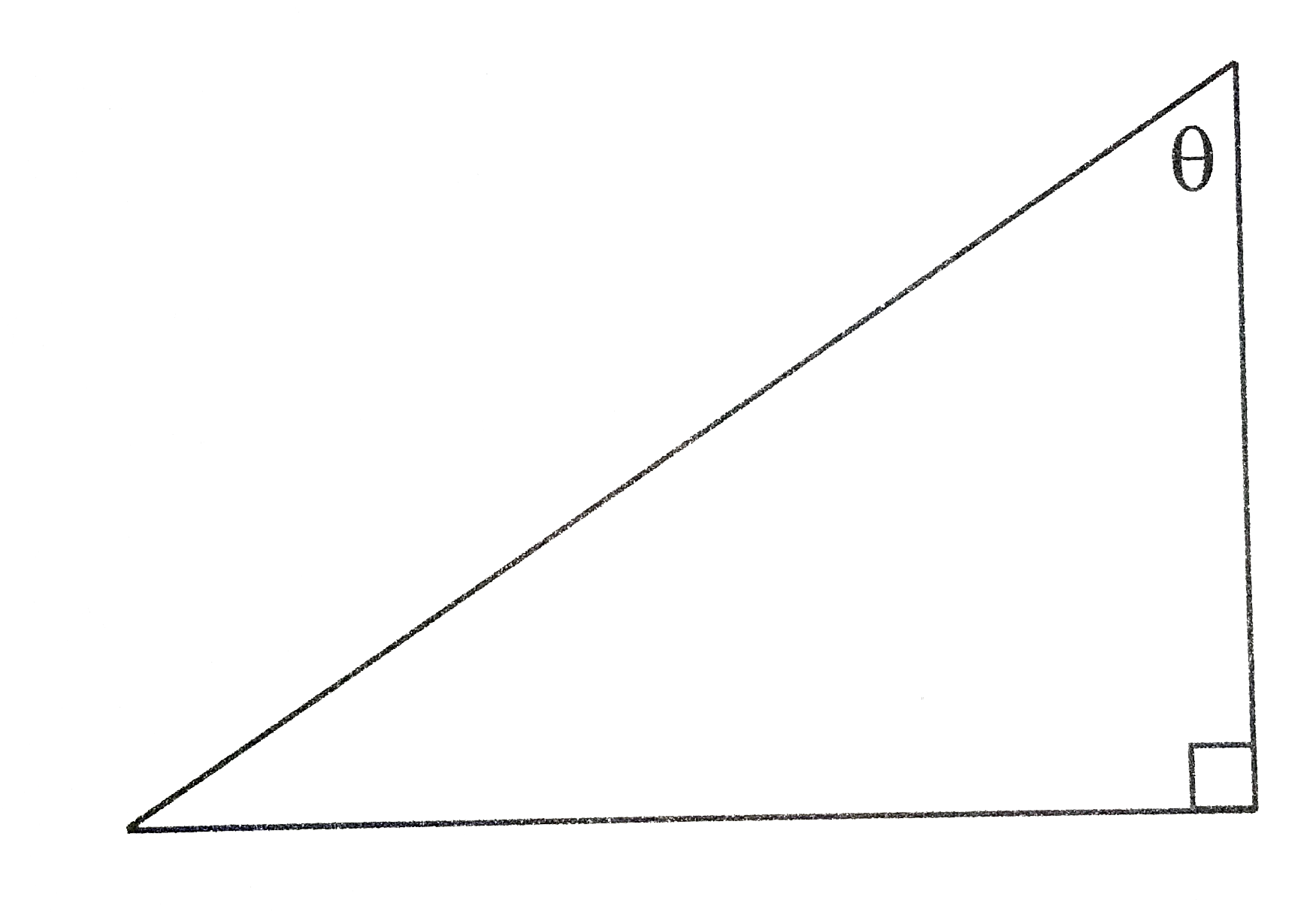
In the figure above, IF cosθ=35, what is the value of cos(90−θ)?A35B34C45D54
What is the minimum value of (sin2θ+cos4θ)?
View SolutionWhat is the value of (1−cos2θ)cosec2θ ?
View Solution→a,→band→c are unit vecrtors such that ∣∣→a+→b+3→c∣∣=4 Angle between →aand→bisθ1 , between →band→cisθ2 and between →aand→b varies [π/6,2π/3] . Then the maximum value of cosθ1+3cosθ2 is
View Solution→a,→band→c are unit vecrtors such that ∣∣→a+→b+3→c∣∣=4 Angle between →aand→bisθ1 , between →band→cisθ2 and between →aand→b varies [π/6,2π/3] . Then the maximum value of cosθ1+3cosθ2 is
View SolutionWhat is the value of 6tan2θ−6cos2θ ?
View SolutionStatement I The maximum value of sinθ+cosθ is 2.
Statement II The maximum value of sinθ is 1 and that of cosθ is also 1.
View SolutionFor θ∈(0,π2), the maximum value of sin(θ+π6)+cos(θ+π6) is attained at θ=
View Solution