100 surnames were randomly picked up from a local telephone directly and the frequency distribution of the number of letters in the English alphabets in the surnames was obtained as follows: Number of letters: 1-4 4-7 7-10 10-13 13-16 16-19 Number surnames 6 30 40 16 4 4 Determine the median number of letters in the surnames. Find the mean number of letters in the surnames. Also, find the modal size of the surnames.
To solve the problem, we need to determine the median, mean, and mode of the number of letters in the surnames based on the given frequency distribution. Let's break it down step by step.
Step 1: Create a Frequency Distribution Table
We are given the following data:
| Number of Letters | Frequency (fi) |
|-------------------|----------------|
| 1 - 4 | 6 |
| 4 - 7 | 30 |
| 7 - 10 | 40 |
| 10 - 13 | 16 |
| 13 - 16 | 4 |
| 16 - 19 | 4 |
Now, we will calculate the cumulative frequency (cf).
| Number of Letters | Frequency (fi) | Cumulative Frequency (cf) |
|-------------------|----------------|----------------------------|
| 1 - 4 | 6 | 6 |
| 4 - 7 | 30 | 36 |
| 7 - 10 | 40 | 76 |
| 10 - 13 | 16 | 92 |
| 13 - 16 | 4 | 96 |
| 16 - 19 | 4 | 100 |
Step 2: Calculate the Median
To find the median, we use the formula:
Median=L+(n/2−CFf)×h
Where:
- L = lower boundary of the median class
- n = total number of observations
- CF = cumulative frequency of the class preceding the median class
- f = frequency of the median class
- h = class width
Finding the Median Class:
- Total number of observations n=100
- n/2=50
- The median class is the first class where the cumulative frequency is greater than or equal to 50, which is 7 - 10 (cf = 76).
Values:
- L=7
- CF=36 (cumulative frequency of the class preceding the median class)
- f=40 (frequency of the median class)
- h=3 (class width, calculated as 10−7)
Substituting the values:
Median=7+(50−3640)×3
=7+(1440)×3
=7+1.05=8.05
Step 3: Calculate the Mean
To calculate the mean, we first need to find the midpoints (xi) of each class interval.
| Number of Letters | Frequency (fi) | Midpoint (xi) | fi * xi |
|-------------------|----------------|----------------|---------|
| 1 - 4 | 6 | 2.5 | 15 |
| 4 - 7 | 30 | 5.5 | 165 |
| 7 - 10 | 40 | 8.5 | 340 |
| 10 - 13 | 16 | 11.5 | 184 |
| 13 - 16 | 4 | 14.5 | 58 |
| 16 - 19 | 4 | 17.5 | 70 |
Calculate ∑fi and ∑(fi⋅xi):
- ∑fi=100
- ∑(fi⋅xi)=15+165+340+184+58+70=832
Mean formula:
Mean=∑(fi⋅xi)∑fi
=832100=8.32
Step 4: Calculate the Mode
The mode is the class with the highest frequency. From the table, the highest frequency is 40, which corresponds to the class 7 - 10.
Using the mode formula:
Mode=L+(f1−f0(2f1−f0−f2))×h
Where:
- L=7 (lower limit of the modal class)
- f1=40 (frequency of the modal class)
- f0=30 (frequency of the class preceding the modal class)
- f2=16 (frequency of the class succeeding the modal class)
- h=3
Substituting the values:
Mode=7+(40−30(2⋅40−30−16))×3
=7+(1080−30−16)×3
=7+(1034)×3
=7+0.882=7.88
Final Results
- Median: 8.05
- Mean: 8.32
- Mode: 7.88
|-------------------|----------------|
| 1 - 4 | 6 |
| 4 - 7 | 30 |
| 7 - 10 | 40 |
| 10 - 13 | 16 |
| 13 - 16 | 4 |
| 16 - 19 | 4 |
|-------------------|----------------|----------------------------|
| 1 - 4 | 6 | 6 |
| 4 - 7 | 30 | 36 |
| 7 - 10 | 40 | 76 |
| 10 - 13 | 16 | 92 |
| 13 - 16 | 4 | 96 |
| 16 - 19 | 4 | 100 |
- L = lower boundary of the median class
- n = total number of observations
- CF = cumulative frequency of the class preceding the median class
- f = frequency of the median class
- h = class width
- Total number of observations n=100
- n/2=50
- The median class is the first class where the cumulative frequency is greater than or equal to 50, which is 7 - 10 (cf = 76).
- L=7
- CF=36 (cumulative frequency of the class preceding the median class)
- f=40 (frequency of the median class)
- h=3 (class width, calculated as 10−7)
Median=7+(50−3640)×3
=7+(1440)×3
=7+1.05=8.05
|-------------------|----------------|----------------|---------|
| 1 - 4 | 6 | 2.5 | 15 |
| 4 - 7 | 30 | 5.5 | 165 |
| 7 - 10 | 40 | 8.5 | 340 |
| 10 - 13 | 16 | 11.5 | 184 |
| 13 - 16 | 4 | 14.5 | 58 |
| 16 - 19 | 4 | 17.5 | 70 |
- ∑fi=100
- ∑(fi⋅xi)=15+165+340+184+58+70=832
Mean=∑(fi⋅xi)∑fi
=832100=8.32
Mode=L+(f1−f0(2f1−f0−f2))×h
- L=7 (lower limit of the modal class)
- f1=40 (frequency of the modal class)
- f0=30 (frequency of the class preceding the modal class)
- f2=16 (frequency of the class succeeding the modal class)
- h=3
Mode=7+(40−30(2⋅40−30−16))×3
=7+(1080−30−16)×3
=7+(1034)×3
=7+0.882=7.88
- Mean: 8.32
- Mode: 7.88
Topper's Solved these Questions
Similar Questions
100 surnames were randomly picked up from a local telephone directory and a frequency distribution of the number of letter in English alphabet in the surnames was found as follows:
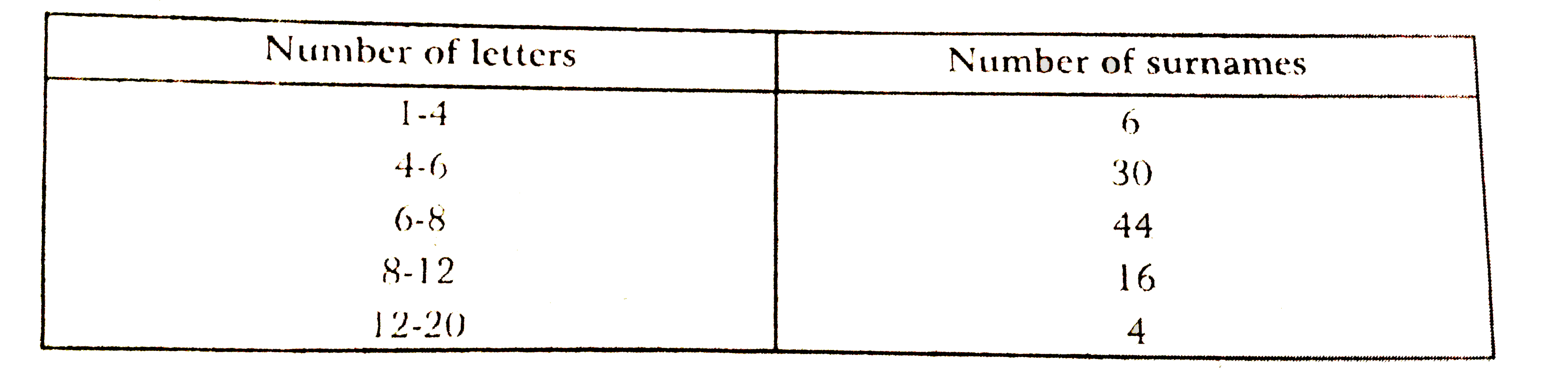
(i) Draw a histogram to depict the given information.
(ii) Write the class interval in which the maximum number of surnames lie.
View SolutionFind the number of terms in the sequence 4,7,10,13…….,82.
View SolutionFind the total number of permutations of the letters of the word INSTITUTE.
View SolutionFind the number of permutations of the letters of the word ALLAHABAD.
View SolutionTwo letters are drawn from the english alphabets.
Find the probability that both letters are vowels.
View SolutionHow many 4-letter code can be formed using the first 10 letters of the English alphabet, if no letter can be repeated?
View SolutionFind the number of arrangements of the letters of the EXAM
View SolutionWrite the set of the letter of the word 'ALLAGABAD'. Also find the number of subsets of this set.
View SolutionWrite the set of the letter of the word 'ALLAHABAD'. Also find the number of subsets of this set.
View SolutionFind the number of permutations of the letters of the word PRE-UNIVERSITY.
View Solution