Radha made a picture of an aeroplane with coloured paper as shown in figure. Find the total area of the paper used.
To find the total area of the paper used to make the aeroplane, we will break down the aeroplane into different geometric shapes and calculate the area of each part separately. The parts include a triangle, a rectangle, a trapezium, and two equal triangles.
Step 1: Calculate the Area of the Triangle (Part 1)
1. Identify the dimensions: The triangle has a base (A) of 5 cm and a height (h) of 5 cm.
2. Use Heron's formula:
- First, we need to find the semi-perimeter (S):
S=A+B+C2=5+5+12=112=5.5 cm
- Now apply Heron's formula:
Area=√S⋅(S−A)⋅(S−B)⋅(S−C)=√5.5⋅(5.5−5)⋅(5.5−5)⋅(5.5−1)
=√5.5⋅0.5⋅0.5⋅4.5=√2.48≈2.48 cm2
Step 2: Calculate the Area of the Rectangle (Part 2)
1. Identify the dimensions: Length (L) = 6.5 cm, Breadth (B) = 1 cm.
2. Calculate the area:
Area=L×B=6.5×1=6.5 cm2
Step 3: Calculate the Area of the Trapezium (Part 3)
1. Identify the dimensions: The lengths of the parallel sides are 2 cm and 1 cm, and the height (h) is 1 cm.
2. Calculate the area:
Area=12×(a+b)×h=12×(2+1)×1=32=1.5 cm2
Step 4: Calculate the Area of the Equal Triangles (Parts 4 and 5)
1. Identify the dimensions: Base = 1.5 cm, Height = 6 cm.
2. Calculate the area of one triangle:
Area=12×Base×Height=12×1.5×6=4.5 cm2
3. Since there are two equal triangles:
Total Area of triangles=2×4.5=9 cm2
Step 5: Calculate the Total Area
1. Sum all the areas:
Total Area=Area of Triangle+Area of Rectangle+Area of Trapezium+Area of Triangles
=2.48+6.5+1.5+9=19.48 cm2
Final Answer
The total area of the paper used to make the aeroplane is 19.48 cm².
---
1. Identify the dimensions: The triangle has a base (A) of 5 cm and a height (h) of 5 cm.
2. Use Heron's formula:
- First, we need to find the semi-perimeter (S):
S=A+B+C2=5+5+12=112=5.5 cm
- Now apply Heron's formula:
Area=√S⋅(S−A)⋅(S−B)⋅(S−C)=√5.5⋅(5.5−5)⋅(5.5−5)⋅(5.5−1)
=√5.5⋅0.5⋅0.5⋅4.5=√2.48≈2.48 cm2
1. Identify the dimensions: Length (L) = 6.5 cm, Breadth (B) = 1 cm.
2. Calculate the area:
Area=L×B=6.5×1=6.5 cm2
1. Identify the dimensions: The lengths of the parallel sides are 2 cm and 1 cm, and the height (h) is 1 cm.
2. Calculate the area:
Area=12×(a+b)×h=12×(2+1)×1=32=1.5 cm2
1. Identify the dimensions: Base = 1.5 cm, Height = 6 cm.
2. Calculate the area of one triangle:
Area=12×Base×Height=12×1.5×6=4.5 cm2
3. Since there are two equal triangles:
Total Area of triangles=2×4.5=9 cm2
1. Sum all the areas:
Total Area=Area of Triangle+Area of Rectangle+Area of Trapezium+Area of Triangles
=2.48+6.5+1.5+9=19.48 cm2
The total area of the paper used to make the aeroplane is 19.48 cm².
Topper's Solved these Questions
Similar Questions
Radha made a picture of an aeroplane with coloured paper as shown in Fig 12.15. Find the total area of the paper used.
View SolutionAn aquarium is in the form of a cuboid whose external measures are 80cm×30cm×40cm. The base, side faces and back face are to be covered with a coloured paper. Find the area of the paper needed?
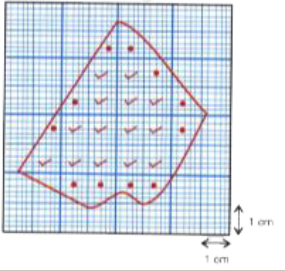
View SolutionThe boundary line of an irregular lamina, on a graph paper is shown in Fig. Find the approximate area of the lamina. In Fig. , the number of complete squares = 14
The number of squares more than half = 11
View SolutionFrom a piece of paper in the shape of a regular hexagon, sectors of circles with the centre at the vertices are removed as shown in the figure. Find the fraction of the vertices are removed as shown in the figure. Find the fraction of the piece of paper left. Also find the perimeter of the piece of paper left in terms of a side of the hexagon

View SolutionFind the area of the blades of the magnetic compass as shown in figure
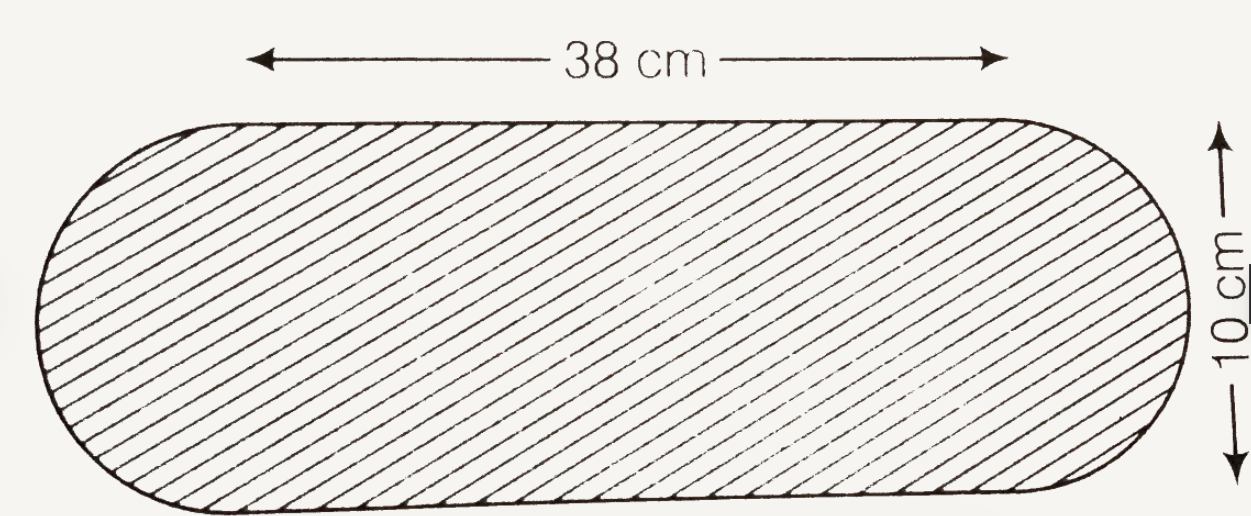
View SolutionFind the area of the flower bed (with semi-circular ends) shown in figure.
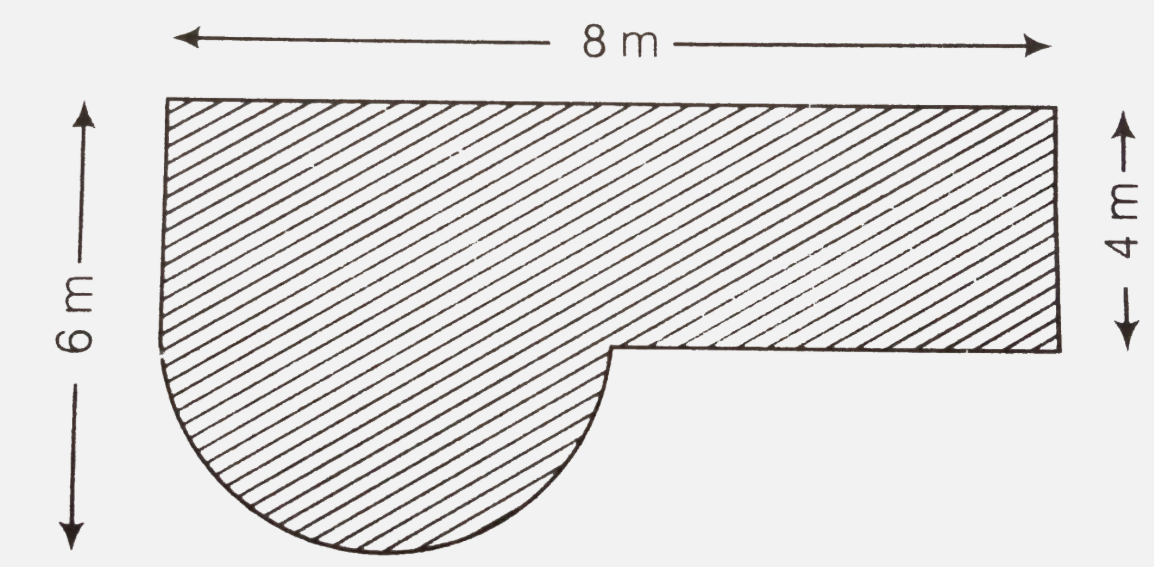
View SolutionFind the area of the shaded field shown in figure.
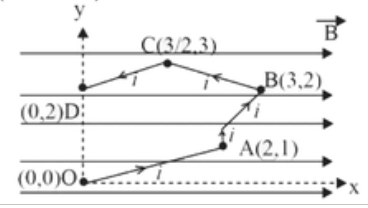
View SolutionA uniform magnetic field →B of magnitude 0.2 T exists in the plane of paper from left to right. A conducting wire, bent in an irregular manner, and carrying a current i , equal to 5A is placed in the plane of paper as shown in figure. Force acting on the wire (in Newton) is
View SolutionThere is a magnetic field acting in a plane perpendicular to this sheet of paper, downward into the paper as shown in the figure. Paticles in acuum move in the plane of the paper from left to right. The path indicated by the arrow could be travelled by

View SolutionA capacitor is made of a flat plate of area A and a second plate having s stair-like structure as shown in figure. The width of each stair is a and the height is d. Both plates have the same width perpendicular to plane of paper. Find the capacitance of the assembly.

View Solution
