Calculate the mean deviation about the mean for the following frequency distribution Class interval 0−4,4−8,8−12,12−16,16−20 Frequency 4,6,8,5,2
To calculate the mean deviation about the mean for the given frequency distribution, we will follow these steps:
Step 1: Organize the Data
We will create a table to organize the class intervals and their corresponding frequencies.
| Class Interval | Frequency (f_i) |
|----------------|-----------------|
| 0 - 4 | 4 |
| 4 - 8 | 6 |
| 8 - 12 | 8 |
| 12 - 16 | 5 |
| 16 - 20 | 2 |
Step 2: Calculate Mid Values (x_i)
Next, we will calculate the mid values for each class interval. The mid value is calculated as the average of the lower and upper bounds of each class interval.
- For 0 - 4: (0 + 4) / 2 = 2
- For 4 - 8: (4 + 8) / 2 = 6
- For 8 - 12: (8 + 12) / 2 = 10
- For 12 - 16: (12 + 16) / 2 = 14
- For 16 - 20: (16 + 20) / 2 = 18
| Class Interval | Frequency (f_i) | Mid Value (x_i) |
|----------------|-----------------|------------------|
| 0 - 4 | 4 | 2 |
| 4 - 8 | 6 | 6 |
| 8 - 12 | 8 | 10 |
| 12 - 16 | 5 | 14 |
| 16 - 20 | 2 | 18 |
Step 3: Calculate f_i * x_i
Now, we will calculate the product of frequency and mid value for each class.
- For 0 - 4: 4 * 2 = 8
- For 4 - 8: 6 * 6 = 36
- For 8 - 12: 8 * 10 = 80
- For 12 - 16: 5 * 14 = 70
- For 16 - 20: 2 * 18 = 36
| Class Interval | Frequency (f_i) | Mid Value (x_i) | f_i * x_i |
|----------------|-----------------|------------------|-----------|
| 0 - 4 | 4 | 2 | 8 |
| 4 - 8 | 6 | 6 | 36 |
| 8 - 12 | 8 | 10 | 80 |
| 12 - 16 | 5 | 14 | 70 |
| 16 - 20 | 2 | 18 | 36 |
Step 4: Calculate Summations
Now, we will calculate the summation of frequencies and the summation of f_i * x_i.
- Σfi=4+6+8+5+2=25
- Σ(fi∗xi)=8+36+80+70+36=230
Step 5: Calculate Mean (x̄)
Now we can calculate the mean (x̄) using the formula:
¯x=Σ(fi∗xi)Σfi=23025=9.2
Step 6: Calculate Deviation from Mean
Next, we will calculate the deviation of each mid value from the mean (x̄):
- For 0 - 4: 2−9.2=−7.2
- For 4 - 8: 6−9.2=−3.2
- For 8 - 12: 10−9.2=0.8
- For 12 - 16: 14−9.2=4.8
- For 16 - 20: 18−9.2=8.8
Step 7: Calculate f_i * (x_i - x̄)
Now we will calculate the product of frequency and the deviation from the mean:
- For 0 - 4: 4∗(−7.2)=−28.8
- For 4 - 8: 6∗(−3.2)=−19.2
- For 8 - 12: 8∗0.8=6.4
- For 12 - 16: 5∗4.8=24
- For 16 - 20: 2∗8.8=17.6
Step 8: Calculate Summation of f_i * (x_i - x̄)
Now we will calculate the summation of f_i * (x_i - x̄):
Σ(fi∗(xi−xˉ))=−28.8−19.2+6.4+24+17.6=0
Step 9: Calculate Mean Deviation
Finally, we can calculate the mean deviation using the formula:
Mean Deviation=Σ(fi∗|xi−xˉ|)Σfi=9625=3.84
Final Answer
The mean deviation about the mean for the given frequency distribution is 3.84.
---
We will create a table to organize the class intervals and their corresponding frequencies.
|----------------|-----------------|
| 0 - 4 | 4 |
| 4 - 8 | 6 |
| 8 - 12 | 8 |
| 12 - 16 | 5 |
| 16 - 20 | 2 |
Next, we will calculate the mid values for each class interval. The mid value is calculated as the average of the lower and upper bounds of each class interval.
- For 4 - 8: (4 + 8) / 2 = 6
- For 8 - 12: (8 + 12) / 2 = 10
- For 12 - 16: (12 + 16) / 2 = 14
- For 16 - 20: (16 + 20) / 2 = 18
|----------------|-----------------|------------------|
| 0 - 4 | 4 | 2 |
| 4 - 8 | 6 | 6 |
| 8 - 12 | 8 | 10 |
| 12 - 16 | 5 | 14 |
| 16 - 20 | 2 | 18 |
Now, we will calculate the product of frequency and mid value for each class.
- For 4 - 8: 6 * 6 = 36
- For 8 - 12: 8 * 10 = 80
- For 12 - 16: 5 * 14 = 70
- For 16 - 20: 2 * 18 = 36
|----------------|-----------------|------------------|-----------|
| 0 - 4 | 4 | 2 | 8 |
| 4 - 8 | 6 | 6 | 36 |
| 8 - 12 | 8 | 10 | 80 |
| 12 - 16 | 5 | 14 | 70 |
| 16 - 20 | 2 | 18 | 36 |
Now, we will calculate the summation of frequencies and the summation of f_i * x_i.
- Σ(fi∗xi)=8+36+80+70+36=230
Now we can calculate the mean (x̄) using the formula:
Next, we will calculate the deviation of each mid value from the mean (x̄):
- For 4 - 8: 6−9.2=−3.2
- For 8 - 12: 10−9.2=0.8
- For 12 - 16: 14−9.2=4.8
- For 16 - 20: 18−9.2=8.8
Now we will calculate the product of frequency and the deviation from the mean:
- For 4 - 8: 6∗(−3.2)=−19.2
- For 8 - 12: 8∗0.8=6.4
- For 12 - 16: 5∗4.8=24
- For 16 - 20: 2∗8.8=17.6
Now we will calculate the summation of f_i * (x_i - x̄):
Finally, we can calculate the mean deviation using the formula:
The mean deviation about the mean for the given frequency distribution is 3.84.
Topper's Solved these Questions
Similar Questions
Calculate the mean deviation from the mean for the following frequency distributions.
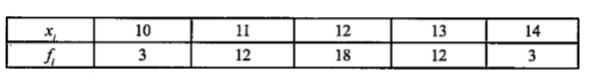 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
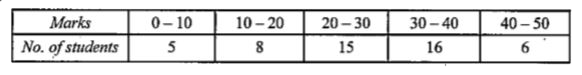 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
 View Solution
View SolutionCalculate the mean deviation from the mean for the following frequency distributions.
 View Solution
View SolutionFind the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
View SolutionFind the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
View SolutionFind the mean of the following frequency distributions: Class interval: 0-6 6-12 12-18 18-24 24-30 Frequency: 6 8 10 9 7
View SolutionFind the mean of the following frequency distributions: Class interval: 0-8 8-16 16-24 24-32 32-40 Frequency: 6 7 10 8 9
View SolutionFind the mean of the following frequency distributions: Class interval: 0-6 6-12 12-18 18-24 24-30 Frequency: 7 5 10 12 6
View Solution

