In a group of 400 people, 160 are smokers and non-vegetarian, 100 are smokers and vegetarian and the remaining are non-smokers and vegetarian. The probabilities of getting a special chest disease are 35%,,20% and 10% respectively. A person is chosen from the group at random and is found to be suffering from the disease. What is the probability that the selected person is a smoker and non-vegetarian?
To solve the problem step by step, we will use Bayes' theorem to find the probability that a randomly selected person suffering from the disease is a smoker and non-vegetarian.
Step 1: Define the Events
Let:
- E1: The event that a person is a smoker and non-vegetarian.
- E2: The event that a person is a smoker and vegetarian.
- E3: The event that a person is a non-smoker and vegetarian.
- A: The event that a person suffers from the disease.
Step 2: Determine the Number of People in Each Category
From the problem:
- Total number of people = 400
- Number of smokers and non-vegetarians (E1) = 160
- Number of smokers and vegetarians (E2) = 100
- Number of non-smokers and vegetarians (E3) = Total - (E1+E2) = 400−(160+100)=140
Step 3: Calculate the Probabilities of Each Event
- P(E1)=160400=0.4
- P(E2)=100400=0.25
- P(E3)=140400=0.35
Step 4: Determine the Probabilities of Disease Given Each Event
From the problem:
- Probability of getting the disease if a person is a smoker and non-vegetarian: P(A|E1)=0.35
- Probability of getting the disease if a person is a smoker and vegetarian: P(A|E2)=0.20
- Probability of getting the disease if a person is a non-smoker and vegetarian: P(A|E3)=0.10
Step 5: Apply Bayes' Theorem
We want to find P(E1|A), which is the probability that a person is a smoker and non-vegetarian given that they have the disease. According to Bayes' theorem:
P(E1|A)=P(E1)⋅P(A|E1)P(E1)⋅P(A|E1)+P(E2)⋅P(A|E2)+P(E3)⋅P(A|E3)
Step 6: Substitute the Values
Substituting the values we calculated:
P(E1|A)=(160400)⋅(35100)(160400)⋅(35100)+(100400)⋅(20100)+(140400)⋅(10100)
Step 7: Calculate the Numerator and Denominator
Numerator:
160400⋅35100=560040000
Denominator:
160400⋅35100+100400⋅20100+140400⋅10100
Calculating each term:
1. 560040000
2. 100400⋅20100=200040000
3. 140400⋅10100=140040000
Now, summing these:
5600+2000+140040000=900040000
Step 8: Final Calculation
Now substituting back into Bayes' theorem:
P(E1|A)=560040000900040000=56009000=2845
Conclusion
The probability that the selected person is a smoker and non-vegetarian given that they are suffering from the disease is 2845.
Let:
- E1: The event that a person is a smoker and non-vegetarian.
- E2: The event that a person is a smoker and vegetarian.
- E3: The event that a person is a non-smoker and vegetarian.
- A: The event that a person suffers from the disease.
From the problem:
- Total number of people = 400
- Number of smokers and non-vegetarians (E1) = 160
- Number of smokers and vegetarians (E2) = 100
- Number of non-smokers and vegetarians (E3) = Total - (E1+E2) = 400−(160+100)=140
- P(E1)=160400=0.4
- P(E2)=100400=0.25
- P(E3)=140400=0.35
From the problem:
- Probability of getting the disease if a person is a smoker and non-vegetarian: P(A|E1)=0.35
- Probability of getting the disease if a person is a smoker and vegetarian: P(A|E2)=0.20
- Probability of getting the disease if a person is a non-smoker and vegetarian: P(A|E3)=0.10
We want to find P(E1|A), which is the probability that a person is a smoker and non-vegetarian given that they have the disease. According to Bayes' theorem:
Substituting the values we calculated:
Numerator:
160400⋅35100=560040000
160400⋅35100+100400⋅20100+140400⋅10100
Calculating each term:
1. 560040000
2. 100400⋅20100=200040000
3. 140400⋅10100=140040000
5600+2000+140040000=900040000
Now substituting back into Bayes' theorem:
The probability that the selected person is a smoker and non-vegetarian given that they are suffering from the disease is 2845.
Topper's Solved these Questions
Similar Questions
From the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
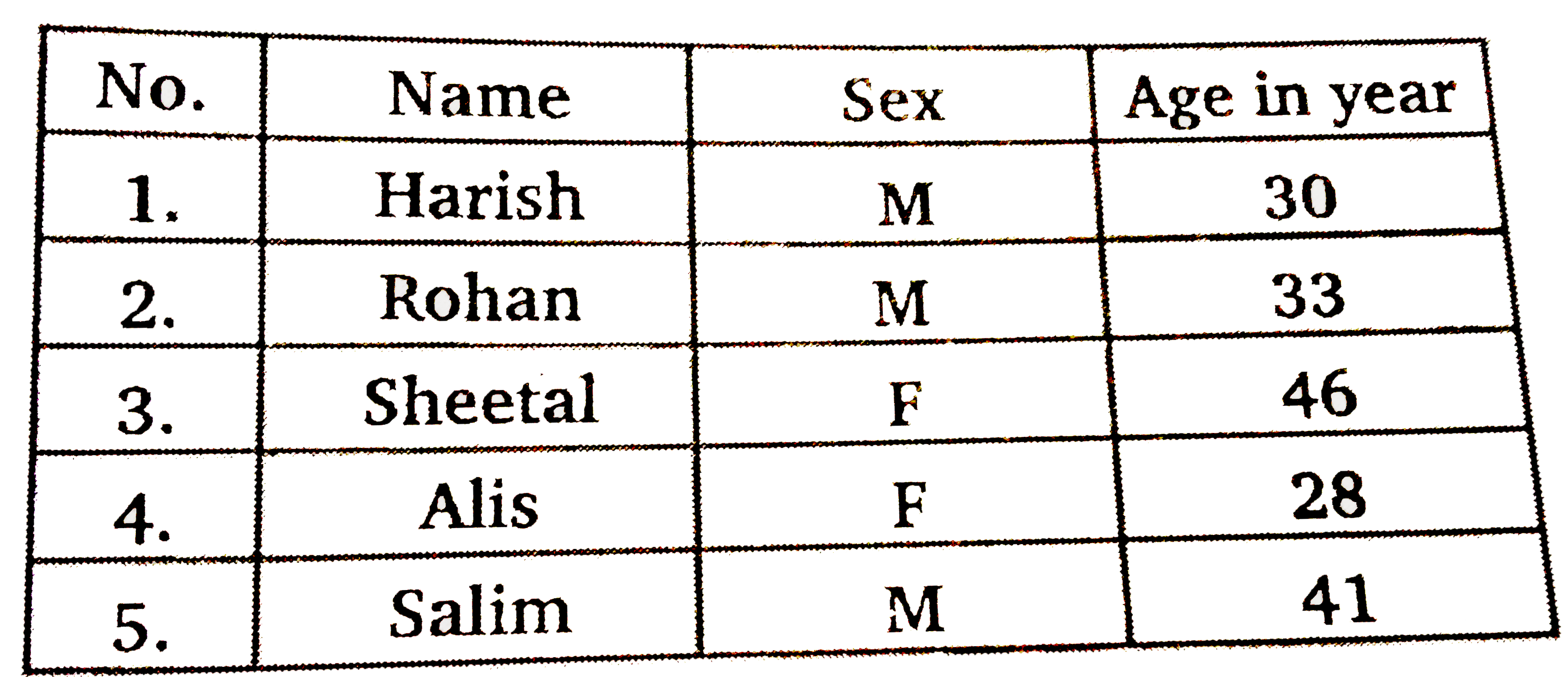
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View SolutionIn a factory which manufactures bolts, machines A. B and C manufacture respectively 25%, 35% and 40% of the bolts. Of their outputs, 5, 4 and 2 percent are respectively defective bolts. A bolt is drawn at random from the product and is found to be defective. What is the probability that it is manufactured by the machine B?
View Solution
Knowledge Check
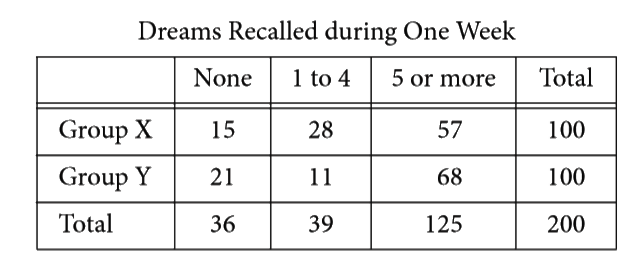
The data in the table above were produced by a sleep researcher studying the number of dreams people recall when asked to record their dreams for one week. Group X consisted of 100 people who observed early bedtimes, and Group Y consisted of 100 people who observed later bedtimes. If a person is chosen at random from those who recalled at least 1 dream, what is the probability that the person belonged to Group Y ?A68100B79100C79164D164200
By examining the. chest X-ray, the probability that TB is detected when a person is actually suffering is 0.99. The probability of an healthy person diagnosed to have TB is 0.001. In a certain city, 1 in 1000 people suffers from TB. A person is selected at random and is diagnosed to have TB. What is the probability that he actually has TB?
View SolutionOf the students in a college, it is known that 60% reside in a hostel and 40% do not reside in hostel. Previous year results report that 30% of students residing in hostel attain A grade and 20% of one’s not residing in hostel attain A grade in their annual examination. At the end of the year, one students is chosen at random from the college and he has an A grade. What is the probability that the selected student is a hosteller?
View SolutionFrom the employees of a company 5 persons are elected to represent them n the managing committee of the company. Particulars of the five persons are as follows: S. No. Person Age (in years) 1 2 3 4 5 Male Male Female Female Male 30 33 46 28 41 A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:S.No. Name Sex Age in years1. Harish M 302. Rohan M 333. Sheetal F 464. Alis F 285. Salim M 41A person is selected at random from this group to act as a spokesperson. What is the probability that the spokesperson will be either male or over 35 years?
View SolutionOut of a group of 30 honest people, 20 always speak the truth. Two persons are selected at random from the group. Find the probability distribution of the number of selected persons who speak the truth. Also find the mean of the distribution
View SolutionOf all the students in a school, it is known that 30% have 100% attendance and 70% students are irregular. Previous year results report that 70% of all students who have 100% attendance attain A grade and 10% irregular students attain A grade in their annual examination. At the end of the year, one student is chosen at random from the school and he was found to have an A grade. What is the probability that the student has 100% attendance ? Is regularity required in school ? Justify your answer.
View SolutionSuppose that 6% of the people with blood group O are left handed and 10% of those with other blood groups are left handed, 30 % of the people have blood groups O. If a left handed person os selected at random, what is the probability that he/she will have blood group O?
View Solution
