In Fig.70, if ABCD, then the value of x and y are x=24, y=48 (b) x=34, y=68 x=24, y=68 (d) x=34, y=48
To solve the problem, we need to find the values of x and y given that AB is parallel to CD and we have certain angle measures. Let's go through the solution step by step.
Step 1: Identify the Given Angles
We are given the following angles:
- ∠NXM=78∘
- ∠XMD=25∘
- ∠ANE=121∘
Step 2: Use the Property of Vertically Opposite Angles
Since AB is parallel to CD and EF is a transversal, the vertically opposite angles are equal. Therefore, we can state:
∠MNB=∠ANE=121∘
Step 3: Apply the Supplementary Angles Property
The angles ∠MNB and ∠NMD are supplementary because they are on the same side of the transversal EF. Thus, we can write:
∠MNB+∠NMD=180∘
Substituting the known value:
121∘+∠NMD=180∘
This implies:
∠NMD=180∘−121∘=59∘
Step 4: Express ∠NMD in Terms of x
We know that:
∠NMD=x+25∘
Setting this equal to our previous result:
x+25∘=59∘
Now, solve for x:
x=59∘−25∘=34∘
Step 5: Use the Angle Sum Property of Triangles
Now we can find y using the triangle MXN. The sum of the angles in a triangle is 180∘:
x+y+78∘=180∘
Substituting the value of x:
34∘+y+78∘=180∘
Now, combine the known angles:
112∘+y=180∘
Solving for y:
y=180∘−112∘=68∘
Final Values
Thus, the values of x and y are:
x=34∘,y=68∘
Conclusion
The correct option is:
(b) x=34,y=68
---
We are given the following angles:
- ∠NXM=78∘
- ∠XMD=25∘
- ∠ANE=121∘
Since AB is parallel to CD and EF is a transversal, the vertically opposite angles are equal. Therefore, we can state:
∠MNB=∠ANE=121∘
The angles ∠MNB and ∠NMD are supplementary because they are on the same side of the transversal EF. Thus, we can write:
∠MNB+∠NMD=180∘
Substituting the known value:
121∘+∠NMD=180∘
This implies:
∠NMD=180∘−121∘=59∘
We know that:
∠NMD=x+25∘
Setting this equal to our previous result:
x+25∘=59∘
Now, solve for x:
x=59∘−25∘=34∘
Now we can find y using the triangle MXN. The sum of the angles in a triangle is 180∘:
x+y+78∘=180∘
Substituting the value of x:
34∘+y+78∘=180∘
Now, combine the known angles:
112∘+y=180∘
Solving for y:
y=180∘−112∘=68∘
Thus, the values of x and y are:
x=34∘,y=68∘
The correct option is:
(b) x=34,y=68
Topper's Solved these Questions
Similar Questions
In Fig.69, if ABCD, then the value of x and y are x=106, y=307 (b) x=307, y=106 x=107, y=306 (d) x=105, y=308
View SolutionIn the given figure, if AB∣∣CE, then the values of x and y are
(a) x=26, y=144
(b) x=36, y=154
(c) x=154, y=36
(d) x=144, y=26
View SolutionIn the given figure, if AB∣∣CD, the values of x and y are
(a) x=21, y=28
(b) x=21, y=38
(c) x=38, y=21
(d) x=22, y=38
View SolutionIn Fig.68, the value of x and y are x=120, y=150 (b) x=110, y=160 x=150, y=120 (d) x=110, y=160
View SolutionIn Fig.71, if ABCD, then the values of x, y and z are x=56, y=47, z=77 (b) x=47, y=56, z=77 x=77, y=56, z=47 (d) x=56, y=77, z=47
View SolutionIn Fig.74, if ABCD, ∠CAB=490, ∠CBD=270 and ∠BCD=1120, then the values of x and y are x=41, y=90, (b) x=41, y=63, x=63, y=41, (d) x=90, y=41,
View SolutionIn Fig.67, the value of x and y are x=130, y=120 (b) x=120, y=130 x=120, y=120 (d) x=130, y=130
View SolutionIn Fig.109, If ABCD then the value of x is (a) 34 (b) 124 (c) 24 (d) 158
View SolutionIn the given figure, the value of x and y are
(a) x=20, y=130
(b) x=40, y=140
(c) x=20, y=140
(d) x=15, y=140
View SolutionIn Fig.72, if AB∣∣CD and AE∣∣BD, then the value of x
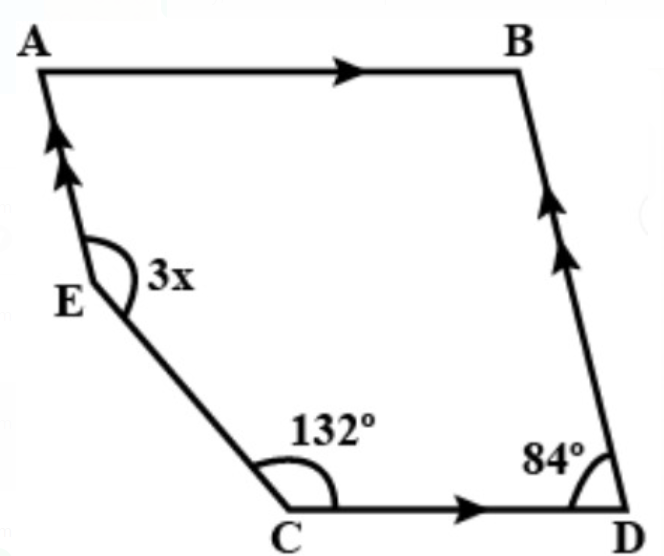 is
(a) 38 (b) 48
(b) 58 (d) 68
is
(a) 38 (b) 48
(b) 58 (d) 68
View Solution
