Question

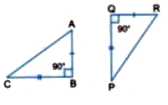
In each of the following
pairs of triangles the measure of some parts are indicated along. Side. By
the application of SAS congruence condition, state which are congruent. State
the result in symbolic form.

Explanation

To determine the congruence of the given pairs of triangles using the SAS (Side-Angle-Side) congruence condition, we will analyze each triangle pair step by step.

1. Identify the Triangles:
We have two pairs of triangles to analyze:
- Triangle ABC and Triangle DEF
- Triangle ABC and Triangle PQR

2. Examine Triangle ABC and Triangle DEF:
- Given:
- Side AB = Side DE
- Angle A ≠ Angle D
- Side AC = Side DF
- Analysis:
- We have two sides (AB and AC) that are equal to two sides (DE and DF) of the other triangle.
- However, the angle A is not equal to angle D.
- Conclusion:
- Since one angle is not equal, the triangles ABC and DEF are not congruent.

3. Examine Triangle ABC and Triangle PQR:
- Given:
- Side BC = Side PQ
- Angle C = Angle P
- Side AC = Side PR
- Analysis:
- We have two sides (BC and AC) that are equal to two sides (PQ and PR) of the other triangle.
- The angle C is equal to angle P.
- Conclusion:
- Since both sides and the included angle are equal, the triangles ABC and PQR are congruent.

4. State the Results in Symbolic Form:
- For the first pair:
- Triangle ABC ≠ Triangle DEF
- For the second pair:
- Triangle ABC ≅ Triangle PQR

Final Results:
- Triangle ABC is not congruent to Triangle DEF (ABC ≠ DEF).
- Triangle ABC is congruent to Triangle PQR (ABC ≅ PQR).