Question

In Fig.38, ${\displaystyle AX}$
bisects${\displaystyle \angle BAC}$
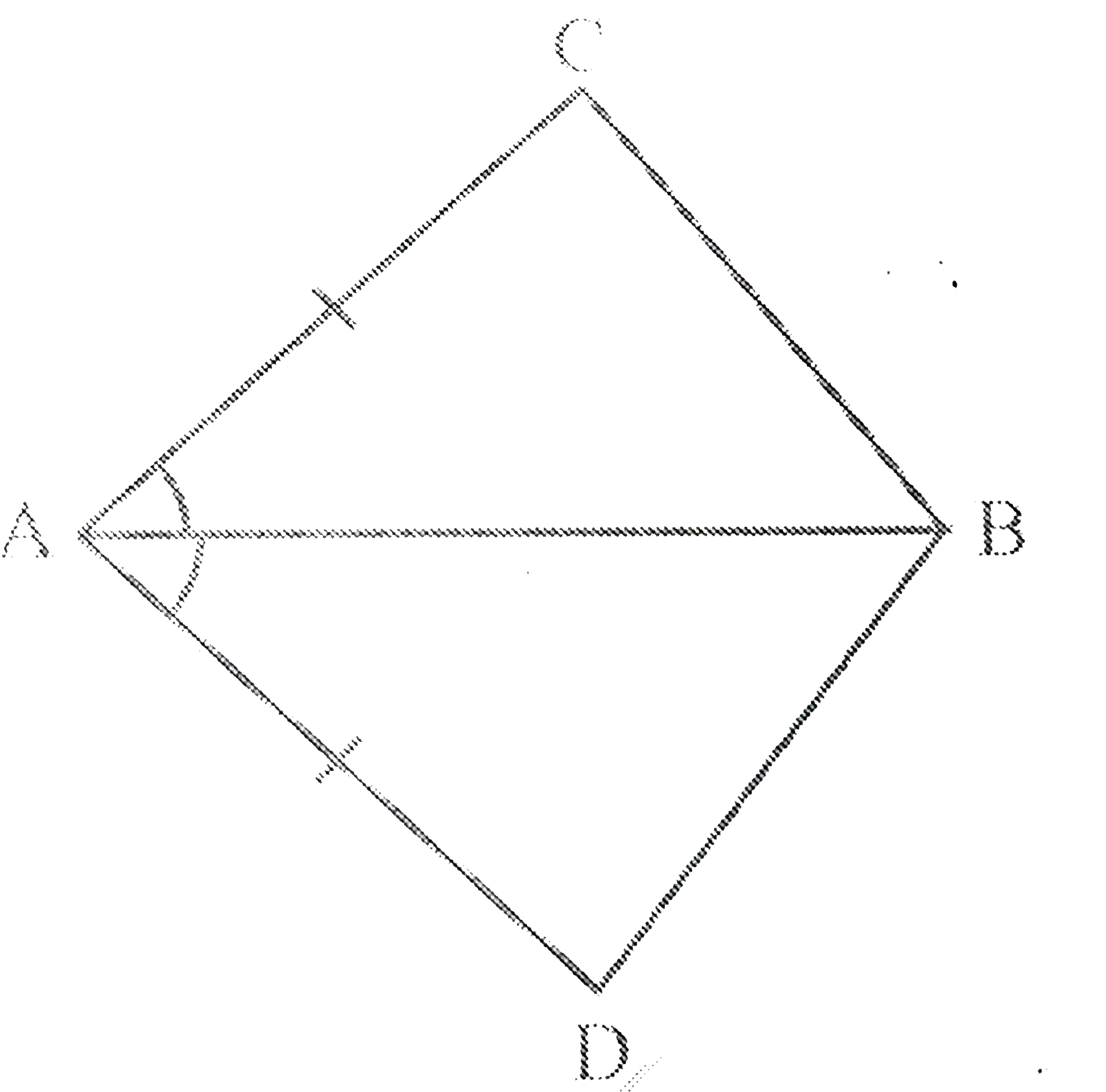
as well as${\displaystyle \angle BDC\stackrel{.}{}}$
State the three facts
needed to ensure that${\displaystyle \Delta ABD\cong \Delta ACD}$

Explanation

To prove that triangle ABD is congruent to triangle ACD, we need to establish three facts based on the information given in the question. Here’s the step-by-step solution:

Step 1: Identify the Angles
Since AX bisects angle BAC, we can denote:
- Let angle BAD = angle 1
- Let angle CAD = angle 2

From the property of angle bisectors, we have:
- angle 1 = angle 2

Step 2: Identify the Other Angles
Similarly, since AX also bisects angle BDC, we can denote:
- Let angle BDA = angle 3
- Let angle CDA = angle 4

From the property of angle bisectors, we have:
- angle 3 = angle 4

Step 3: Identify the Common Side
The side AD is common to both triangles ABD and ACD. Therefore, we can state:
- AD = AD (common side)

Conclusion
Now, we can summarize the three facts needed for the congruence of triangles ABD and ACD:
1. angle BAD (angle 1) = angle CAD (angle 2) (from the bisector of angle BAC)
2. angle BDA (angle 3) = angle CDA (angle 4) (from the bisector of angle BDC)
3. AD = AD (common side)

Based on these three facts, we can conclude that triangle ABD is congruent to triangle ACD by the Angle-Side-Angle (ASA) congruence criterion.

---