State the number of lines of symmetry for the following figures: (i) A rhombus (ii) A square
To determine the number of lines of symmetry for the given figures, we will analyze each figure step by step.
Step 1: Understand the concept of lines of symmetry
A line of symmetry in a figure is a line that divides the figure into two identical halves that are mirror images of each other.
Step 2: Analyze the rhombus
1. Identify the rhombus: A rhombus is a quadrilateral with all four sides of equal length.
2. Draw the diagonals: A rhombus has two diagonals. Let's label the rhombus as ABCD.
- Diagonal AC and diagonal BD.
3. Determine the lines of symmetry:
- When we draw diagonal AC, it divides the rhombus into two identical triangles (ABC and ADC).
- When we draw diagonal BD, it also divides the rhombus into two identical triangles (ABD and BCD).
4. Count the lines of symmetry: Therefore, the rhombus has 2 lines of symmetry.
Step 3: Analyze the square
1. Identify the square: A square is a quadrilateral with all sides equal and all angles equal (90 degrees).
2. Draw the lines of symmetry:
- The square has 4 lines of symmetry:
- 2 lines through the midpoints of opposite sides (vertical and horizontal).
- 2 lines through the diagonals (from corner to corner).
3. Count the lines of symmetry: Therefore, the square has 4 lines of symmetry.
Final Answer
- The number of lines of symmetry for a rhombus is 2.
- The number of lines of symmetry for a square is 4.
---
A line of symmetry in a figure is a line that divides the figure into two identical halves that are mirror images of each other.
1. Identify the rhombus: A rhombus is a quadrilateral with all four sides of equal length.
2. Draw the diagonals: A rhombus has two diagonals. Let's label the rhombus as ABCD.
- Diagonal AC and diagonal BD.
3. Determine the lines of symmetry:
- When we draw diagonal AC, it divides the rhombus into two identical triangles (ABC and ADC).
- When we draw diagonal BD, it also divides the rhombus into two identical triangles (ABD and BCD).
4. Count the lines of symmetry: Therefore, the rhombus has 2 lines of symmetry.
1. Identify the square: A square is a quadrilateral with all sides equal and all angles equal (90 degrees).
2. Draw the lines of symmetry:
- The square has 4 lines of symmetry:
- 2 lines through the midpoints of opposite sides (vertical and horizontal).
- 2 lines through the diagonals (from corner to corner).
3. Count the lines of symmetry: Therefore, the square has 4 lines of symmetry.
- The number of lines of symmetry for a rhombus is 2.
- The number of lines of symmetry for a square is 4.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The number of lines of symmetry of a circle.
A1B2C10DInfinite manyThe number of lines of symmetry of a circle is
A0B1C4DunlimitedThe number of lines of symmetry of a rectangle is
A0B1C2D4
Draw the lines of symmetry for the following shapes:
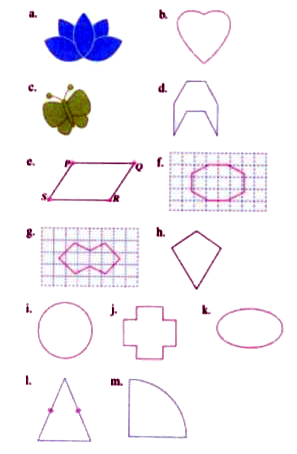 .
.
View SolutionState the number of lines of symmetry for the following figures: (i) A circle (ii) A semi-circle
View SolutionState the number of lines of symmetry for the following figures: (i) A parallelogram (ii) A quadrilateral
View SolutionThe number of lines (s) of symmetry of a line segment is
View SolutionThe number of lines of symmetry of a scalene triangles is
View Solution

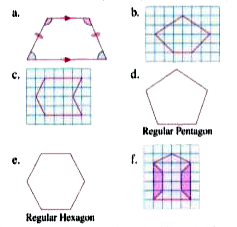 .
. .
.