In a parallelogram, two adjacent angles are supplementary.
To prove that in a parallelogram, two adjacent angles are supplementary, we can follow these steps:
Step 1: Define the Parallelogram
Let’s denote the parallelogram as ABCD, where A, B, C, and D are the vertices of the parallelogram.
Step 2: Identify Adjacent Angles
Adjacent angles in a parallelogram are pairs of angles that share a common side. For parallelogram ABCD, the adjacent angles are:
- Angle A and Angle B
- Angle B and Angle C
- Angle C and Angle D
- Angle D and Angle A
Step 3: Recall the Properties of a Parallelogram
In a parallelogram, opposite sides are equal and parallel. Therefore, we have:
- Side AB is parallel to side CD
- Side AD is parallel to side BC
Step 4: Use the Transversal Property
Since AB is parallel to CD, and AD acts as a transversal, we can apply the property of angles formed by a transversal cutting through parallel lines. According to this property, the sum of the interior angles on the same side of the transversal is equal to 180 degrees.
Step 5: Apply the Property to Angles A and B
Using the property mentioned above, we can say:
- Angle A + Angle B = 180 degrees
This shows that Angle A and Angle B are supplementary.
Step 6: Repeat for Other Pairs of Adjacent Angles
Similarly, we can prove for the other pairs:
- Angle B + Angle C = 180 degrees (using BC as a transversal)
- Angle C + Angle D = 180 degrees (using CD as a transversal)
- Angle D + Angle A = 180 degrees (using AD as a transversal)
Conclusion
Thus, we have shown that in a parallelogram, any two adjacent angles are supplementary, meaning their sum is 180 degrees.
---
Let’s denote the parallelogram as ABCD, where A, B, C, and D are the vertices of the parallelogram.
Adjacent angles in a parallelogram are pairs of angles that share a common side. For parallelogram ABCD, the adjacent angles are:
- Angle A and Angle B
- Angle B and Angle C
- Angle C and Angle D
- Angle D and Angle A
In a parallelogram, opposite sides are equal and parallel. Therefore, we have:
- Side AB is parallel to side CD
- Side AD is parallel to side BC
Since AB is parallel to CD, and AD acts as a transversal, we can apply the property of angles formed by a transversal cutting through parallel lines. According to this property, the sum of the interior angles on the same side of the transversal is equal to 180 degrees.
Using the property mentioned above, we can say:
- Angle A + Angle B = 180 degrees
This shows that Angle A and Angle B are supplementary.
Similarly, we can prove for the other pairs:
- Angle B + Angle C = 180 degrees (using BC as a transversal)
- Angle C + Angle D = 180 degrees (using CD as a transversal)
- Angle D + Angle A = 180 degrees (using AD as a transversal)
Thus, we have shown that in a parallelogram, any two adjacent angles are supplementary, meaning their sum is 180 degrees.
Topper's Solved these Questions
Similar Questions
Consider the following statements: When two straight lines intersect:
(i)adjacent angles are complementary
(ii)adjacent angles are supplementary
(iii)opposite angles are equal
(iv)opposite angles are supplementary
Of those statements which is correct
View SolutionIn a parallelogram the sum of any two adjacent angles is 1800.
View SolutionA field is in the shape of a parallelogram. The two adjacent sides are in the ratio 4:5. A fence 216 m long is placed along the boundary of the field. Find the sides of the parallelogram.
View SolutionDefine Adjacent angles.
View SolutionFill in the blanks:(i) If two angles are complementary, then the sum of their measures is _______.(ii) If two angles are supplementary, then the sum of their measures is ______.(iii) Two angles forming a linear pair are _______________.(iv) If two adjacent angles are supplementary, they form a ___________.(v) If two lines intersect at a point, then the vertically opposite angles are always_____________.(vi) If two lines intersect at a point, and if one pair of vertically opposite angles areacute angles, then the other pair of vertically opposite angles are __________.
View SolutionIn parallelogram ABCD, the bisectors of adjacent angles A and D intersect each other at point P. prove that ∠APD=90∘.
View SolutionIf an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram.
View SolutionIf an angle of a parallelogram is two-third of its adjacent angle, find the smallest angle of the parallelogram.
View SolutionIf an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram.
View Solution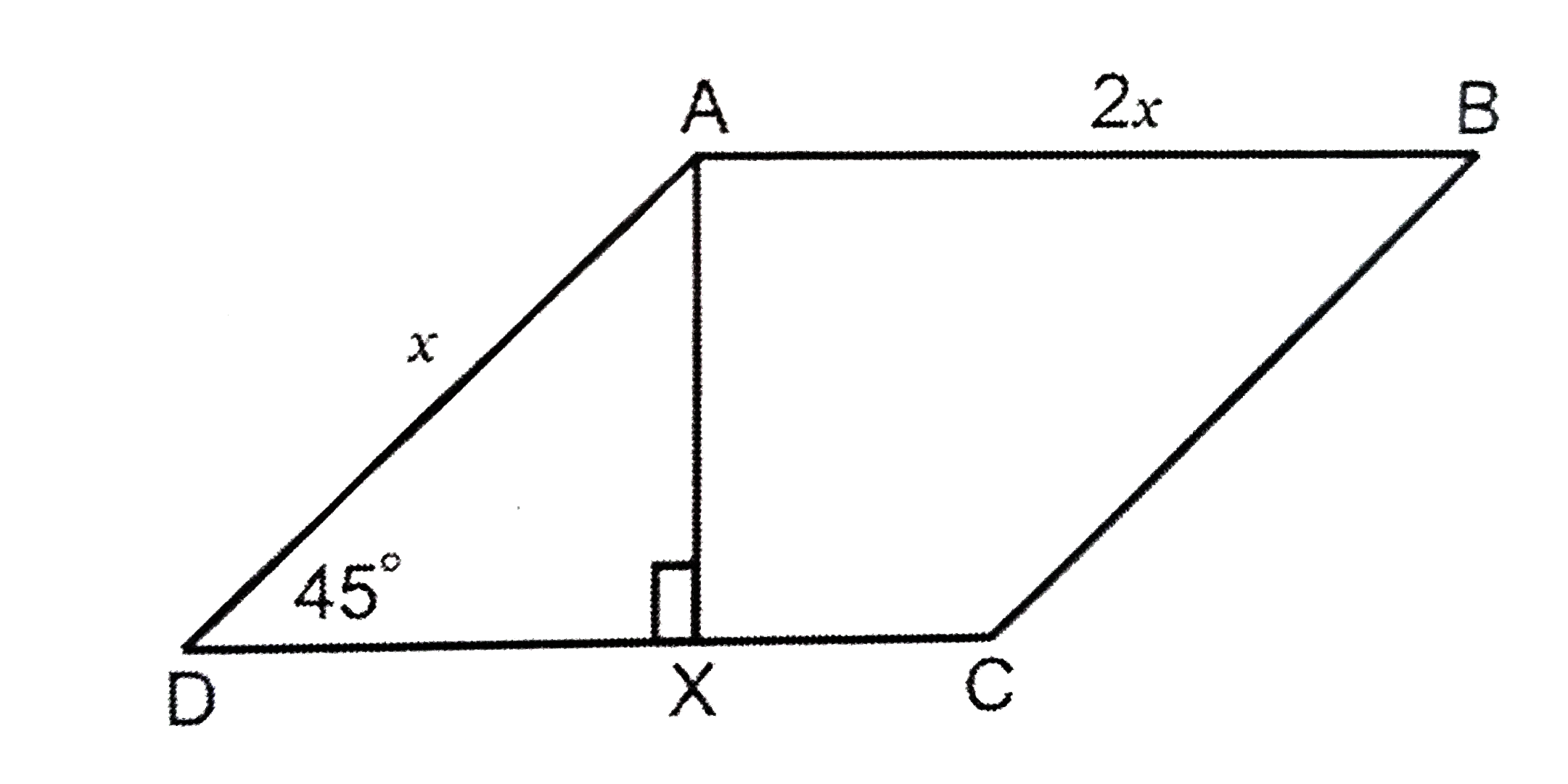
In a parallelogram, the ratio of the two adjacent sides is 1 : 2. If the area of the parallelogram is 20 square units and the angle beween the two sides is 45∘, what is the area, to the nearest integer, of the rectangle having the sides equal to that of the parallelogram?
View Solution
