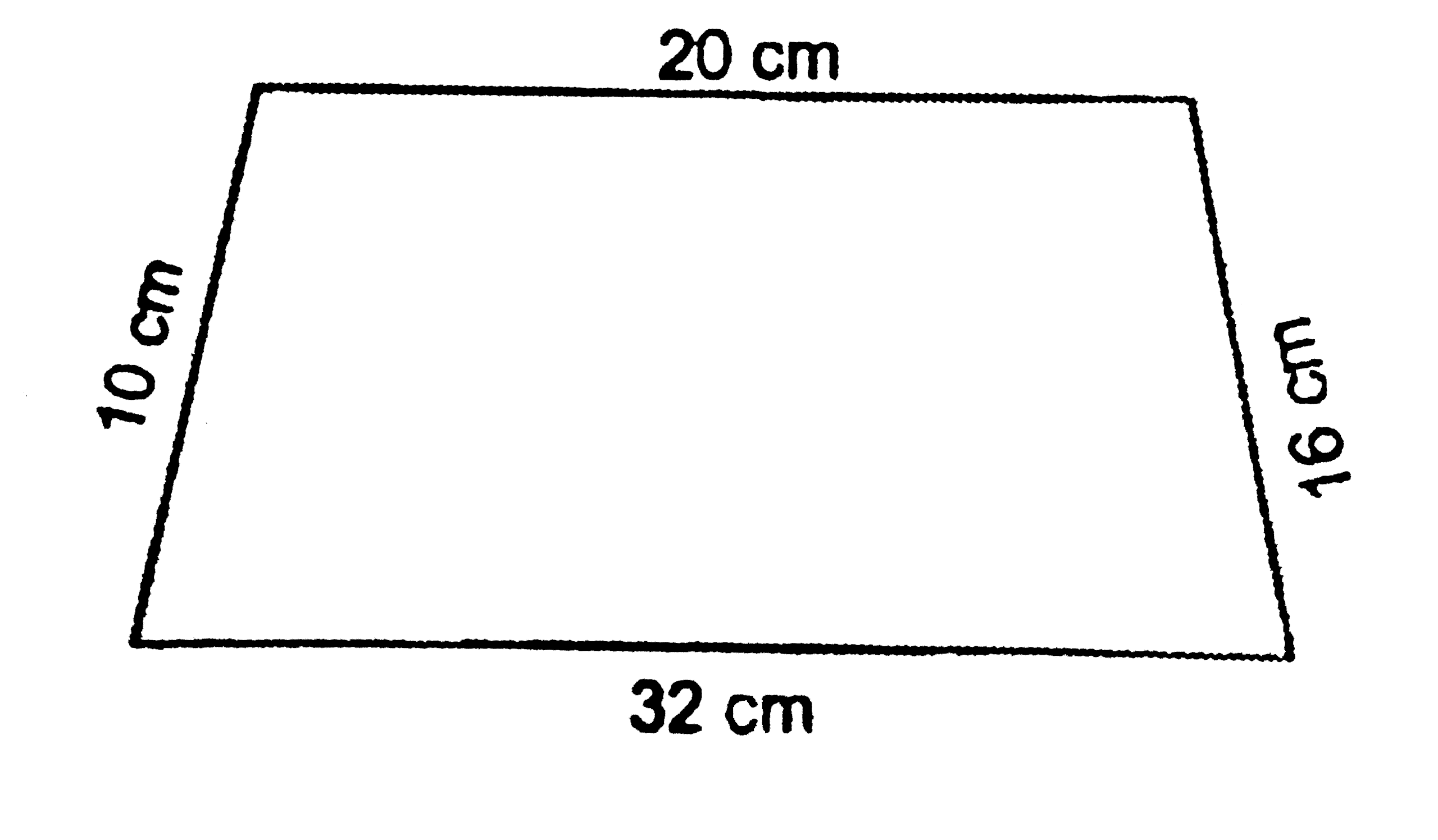
In Figure, a parallelogram is drawn in a trapezium, the area of the parallelogram is 80 cm2, find the area of the trapezium.
To find the area of the trapezium given that the area of the parallelogram is 80cm2, we can follow these steps:
1. Identify the Given Information:
- Area of the parallelogram =80cm2.
- Let the base of the parallelogram =a=10cm.
- Let the height of the parallelogram (which is also the height of the trapezium) =df.
2. Use the Area Formula for the Parallelogram:
- The area of a parallelogram is given by the formula:
Area=Base×Height
- Substituting the known values:
80=10×df
3. Calculate the Height df:
- Rearranging the equation to find df:
df=8010=8cm
4. Identify the Parallel Sides of the Trapezium:
- Let the lengths of the parallel sides of the trapezium be AB=22cm and CD=10cm.
5. Use the Area Formula for the Trapezium:
- The area of a trapezium is given by the formula:
Area=12×(Base1+Base2)×Height
- Substituting the values:
Area=12×(22+10)×8
6. Calculate the Area of the Trapezium:
- First, calculate the sum of the bases:
22+10=32
- Now substitute back into the area formula:
Area=12×32×8
- Calculate:
Area=16×8=128cm2
7. Final Answer:
- Therefore, the area of the trapezium is 128cm2.
- Area of the parallelogram =80cm2.
- Let the base of the parallelogram =a=10cm.
- Let the height of the parallelogram (which is also the height of the trapezium) =df.
- The area of a parallelogram is given by the formula:
Area=Base×Height
- Substituting the known values:
80=10×df
- Rearranging the equation to find df:
df=8010=8cm
- Let the lengths of the parallel sides of the trapezium be AB=22cm and CD=10cm.
- The area of a trapezium is given by the formula:
Area=12×(Base1+Base2)×Height
- Substituting the values:
Area=12×(22+10)×8
- First, calculate the sum of the bases:
22+10=32
- Now substitute back into the area formula:
Area=12×32×8
- Calculate:
Area=16×8=128cm2
- Therefore, the area of the trapezium is 128cm2.
Topper's Solved these Questions
- LINEAR EQUATION IN ONE VARIABLEBOOK - RD SHARMA ENGLISHCHAPTER - LINEAR EQUATION IN ONE VARIABLEEXERCISE - All Questions
110 Videos
- MENSURATION-II (VOLUMES AND SURFACE AREAS OF A CUBOID AND A CUBE)BOOK - RD SHARMA ENGLISHCHAPTER - MENSURATION-II (VOLUMES AND SURFACE AREAS OF A CUBOID AND A CUBE)EXERCISE - All Questions
134 Videos
Similar Questions
Knowledge Check
The base of a parallelogram is one - third of it’s height, the area of this parallelogram is 147cm2 , find its height .
A=24 cm .B=21 cm .C=22 cm .D=23 cm .
In the figure, the area of parallelogram ABCD is
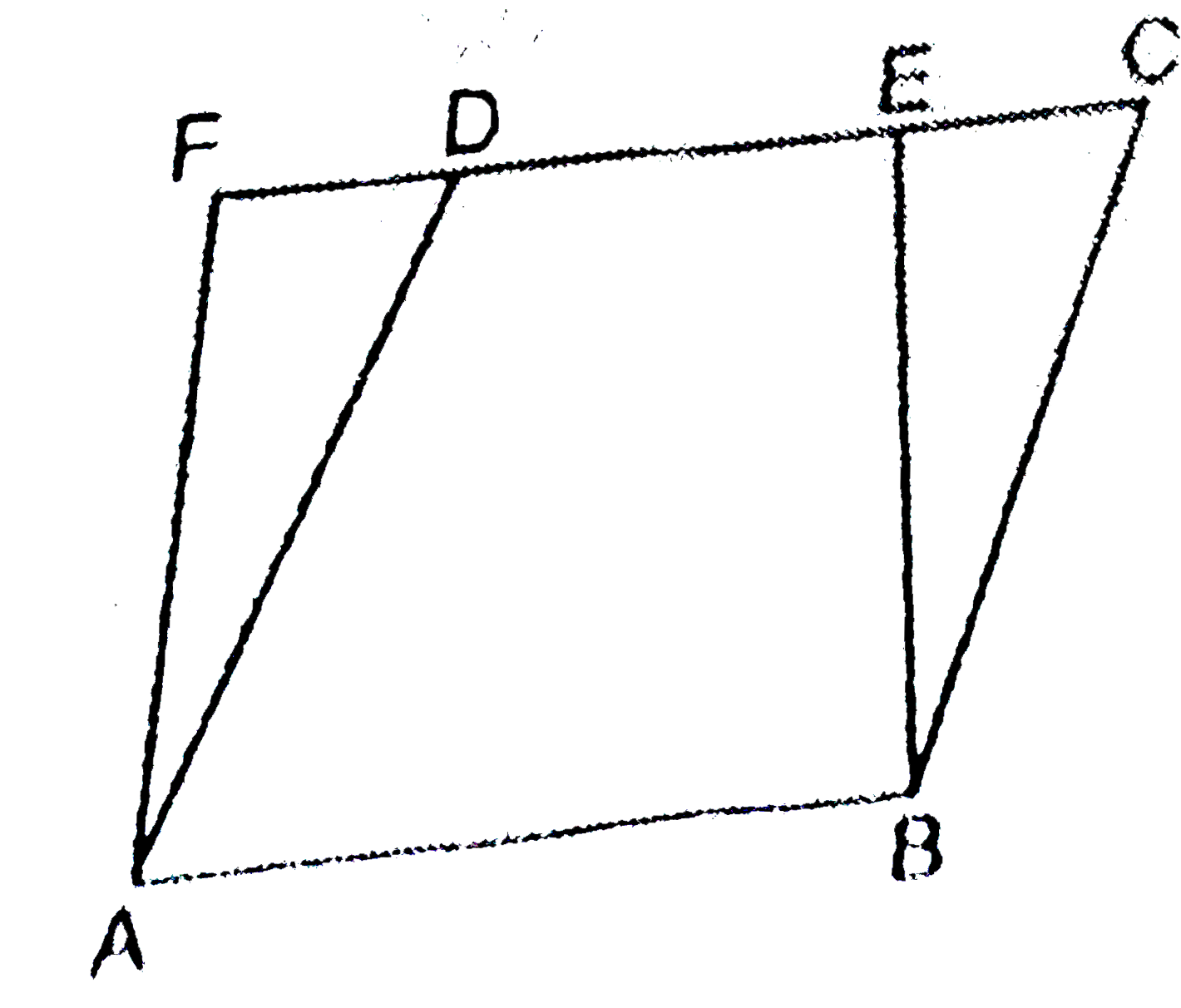
View SolutionIn the given figure, if the area of parallelogram ABCD is 40cm2, find the area of parallelogram ABEF
View SolutionThe diagonals of a parallelogram are 2ˆi and 2ˆj. What is the area of the parallelogram
View SolutionThe base of a parallelogram is 3 times of its corresponding height. If the area of the parallelogram is 48cm2, then find the base and the corresponding height of the parallelogram.
View SolutionThe height of a parallelogram is three-eighths of its base. If the area of the parallelogram is 96cm2 , find its height and base .
View SolutionThe parallel sides of a trapezium are 25cm and 13cm; its nonparallel sides are equal, each being 10cm, find the area of the trapezium.
View SolutionThe area of a parallelogram is y cm2 and its height is h cm. The base of another parallelogram is x cm more than the base of the first parallelogram and its area is twice the area of the first. Find, in terms of y, h and x, the expression for the height of the second parallelogram.
View Solution