The metre is defined as the distance travelled by ligh in 1299,792,458 second. Why didn't people choose some easier number such as 1300.000.000 second?
To understand why the meter is defined as the distance light travels in 1299,792,458 seconds instead of a simpler fraction like 1300,000,000 seconds, we need to consider the actual speed of light and how it relates to the definition of a meter.
1. Understanding the Speed of Light:
The speed of light in a vacuum is approximately 299,792,458 meters per second. This value is a precise measurement and is fundamental in physics.
2. Defining the Meter:
The meter is defined as the distance light travels in 1c seconds, where c is the speed of light. Therefore, using the precise value of c, the meter is defined as the distance light travels in 1299,792,458 seconds.
3. Using a Simpler Number:
If we were to use 1300,000,000 seconds, we would need to calculate the distance light travels in that time using the speed of light.
4. Calculating Distance with 1300,000,000 Seconds:
- Distance s can be calculated using the formula:
s=v×t
- Here, v is the speed of light c=299,792,458 m/s and t=1300,000,000 seconds.
- Substituting the values:
s=299,792,458×1300,000,000
5. Performing the Calculation:
- Calculate s:
s≈0.999308 meters
- This means that using 1300,000,000 seconds would yield a distance slightly less than 1 meter.
6. Conclusion:
The reason for using 1299,792,458 seconds is that it provides a precise definition of the meter that corresponds exactly to the distance light travels in that time. Using 1300,000,000 seconds would not yield a full meter, leading to inaccuracies in measurements.
The speed of light in a vacuum is approximately 299,792,458 meters per second. This value is a precise measurement and is fundamental in physics.
The meter is defined as the distance light travels in 1c seconds, where c is the speed of light. Therefore, using the precise value of c, the meter is defined as the distance light travels in 1299,792,458 seconds.
If we were to use 1300,000,000 seconds, we would need to calculate the distance light travels in that time using the speed of light.
- Distance s can be calculated using the formula:
s=v×t
- Here, v is the speed of light c=299,792,458 m/s and t=1300,000,000 seconds.
- Substituting the values:
s=299,792,458×1300,000,000
- Calculate s:
s≈0.999308 meters
- This means that using 1300,000,000 seconds would yield a distance slightly less than 1 meter.
The reason for using 1299,792,458 seconds is that it provides a precise definition of the meter that corresponds exactly to the distance light travels in that time. Using 1300,000,000 seconds would not yield a full meter, leading to inaccuracies in measurements.
Topper's Solved these Questions
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Exercises
19 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER
11 Videos
- KINETIC THEORY OF GASESBOOK - HC VERMA ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - All Questions
106 Videos
Similar Questions
A freely falling body traveled xm in nth second distance travelled in n−1th second is
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
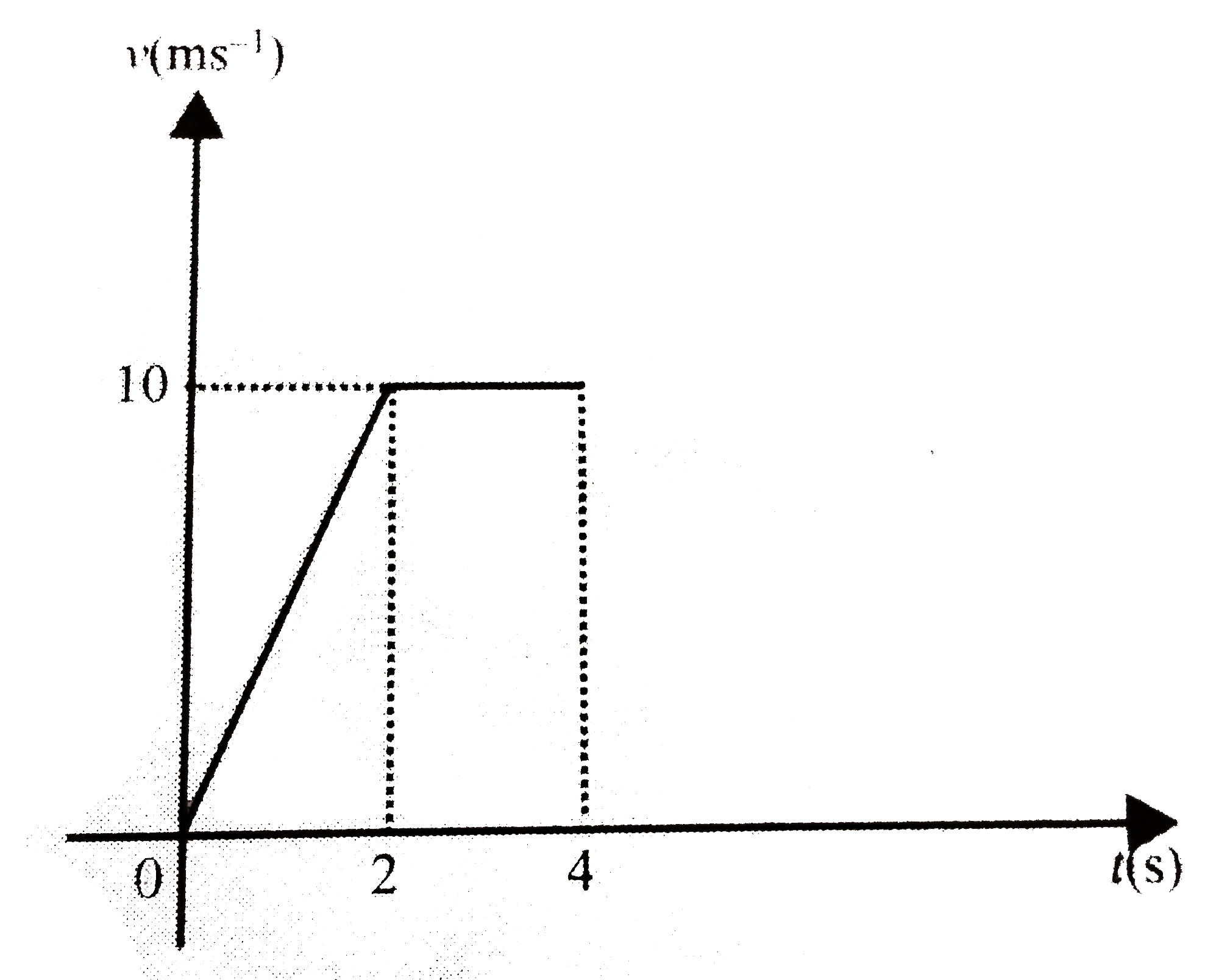
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionA particle is moving in a straight line with initial velocity u and uniform acceleration f. If the sum of the distances travelled in tthand(t+1)th seconds is 100cm, then its velocity after t seconds, in cm/s, is.
View SolutionA car travels 25 m in 1 second. How much distance will it cover in 2 minutes ?
View SolutionA body is thrown up with a velocity 29.23ms−1 distance travelled in last second of upward motion is
View SolutionThe distance travelled by a particle in nth second is Sn=u+a2(2n−1) where u is the velocity and a is the acceleration. The equation is
View SolutionAssertion : In the equation, s=u+at−12a where, s is the distance travelled by uniformly accelerated body in tth second.
Reason : The above equation is dimensionally incorrect.
View SolutionA body is projected with a velocity 50ms−1. Distance travelled in 6th second is [g=10ms−2]
View SolutionConsider a particle intially moving with a velocity of 5 m s−1 starts decelerating at a constant rate of 2 m s−2.
a. Determine the time at which the particle becomes stationary.
b. Find the distance travelled in the second second.
c. Find the distance travelled in the third second.
View SolutionA body is projected with a velocity 60ms−1 vertically upwards the distance travelled in last second of its motion is [g=10ms−1]
View Solution
