A article moves in a circle of radius 20 cm with linear speed of 10 m/s. Find the angular velocity
To solve the problem of finding the angular velocity of an article moving in a circle, we can follow these steps:
Step 1: Identify the given data
- Linear speed (v) = 10 m/s
- Radius (r) = 20 cm
Step 2: Convert the radius from centimeters to meters
Since we want to use SI units, we need to convert the radius from centimeters to meters.
- Conversion: 1 cm = 0.01 m
- Thus, 20 cm = 20 × 0.01 m = 0.2 m
Step 3: Use the formula for angular velocity
The relationship between linear speed (v), radius (r), and angular velocity (ω) is given by the formula:
v=r⋅ω
From this formula, we can express angular velocity (ω) as:
ω=vr
Step 4: Substitute the values into the formula
Now, we can substitute the known values into the formula:
- v=10m/s
- r=0.2m
So,
ω=10m/s0.2m
Step 5: Calculate the angular velocity
Now we perform the division:
ω=100.2=50radians/second
Final Answer
The angular velocity (ω) of the article is 50 radians/second.
---
- Linear speed (v) = 10 m/s
- Radius (r) = 20 cm
Since we want to use SI units, we need to convert the radius from centimeters to meters.
- Conversion: 1 cm = 0.01 m
- Thus, 20 cm = 20 × 0.01 m = 0.2 m
The relationship between linear speed (v), radius (r), and angular velocity (ω) is given by the formula:
v=r⋅ω
From this formula, we can express angular velocity (ω) as:
ω=vr
Now, we can substitute the known values into the formula:
- v=10m/s
- r=0.2m
ω=10m/s0.2m
Now we perform the division:
ω=100.2=50radians/second
The angular velocity (ω) of the article is 50 radians/second.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - worked out Examples13videos
CIRCULAR MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Objective -116videos
CIRCULAR MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -211videos
CENTRE OF MASS, LINEAR MOMENTUM, COLLISION
- BOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions90videos
FLUID MECHANICS
Similar Questions
A particle moves in a circle of radius 4 m with a linear velocity of 20ms−1. Find the angular velocity.
View Solution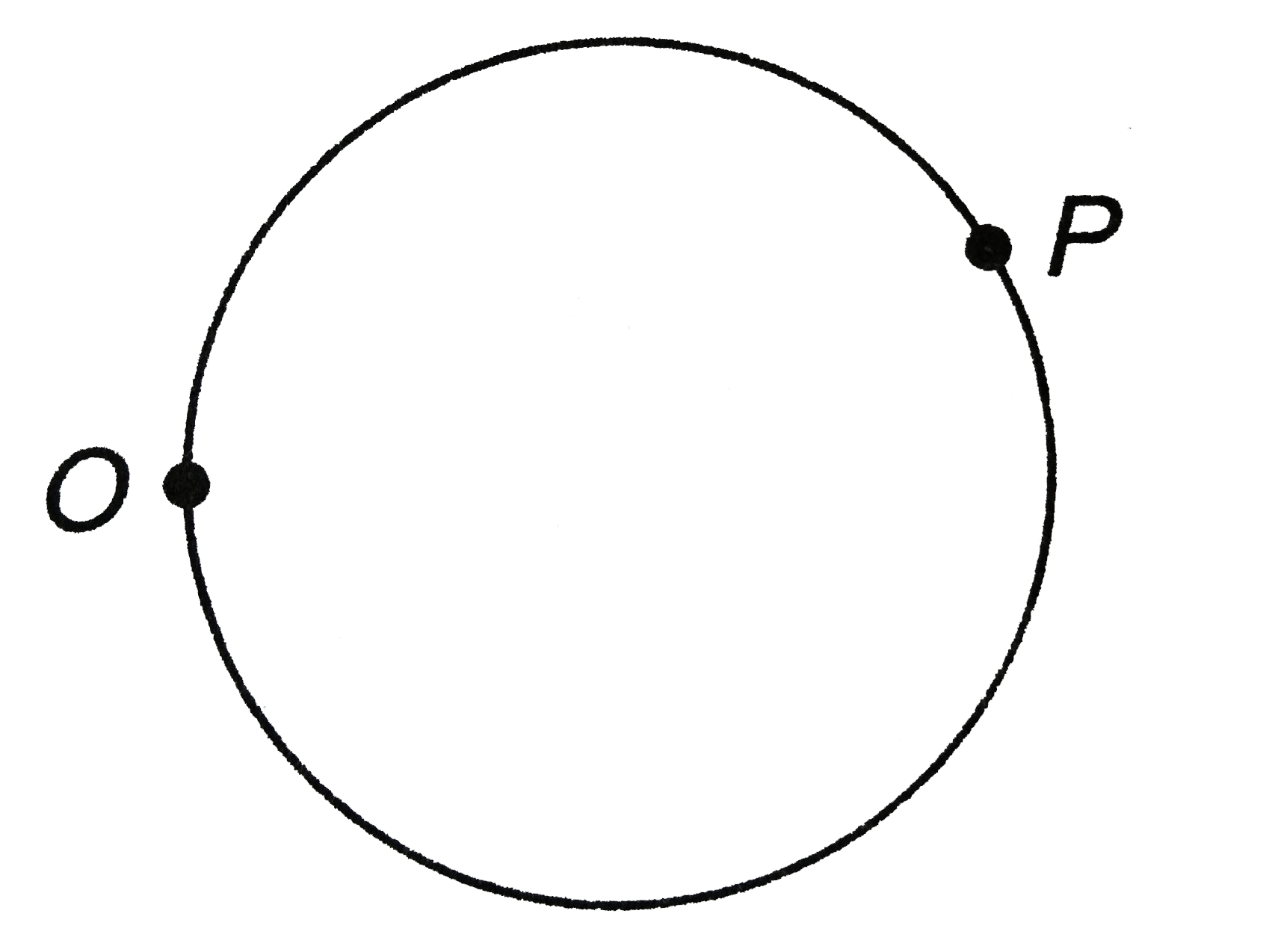
Particle P shown in figure is moving in a circle of radius R=10 cm with linear speed v=2m/s Find the angular speed of particle about point O.View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View SolutionA particle is moving in a circle of radius 4cm with constant speed of 1cm/s. Find
(a) time period of the particle.
(b) average speed, average velocity and average acceleration in a time interval from t=0 to t=T4. Here, T is the time period of the particle. Give only their magnitudes.View SolutionA particle moves in a circle of radius R=2122m with constant speed 1m/s. Find,
(a) magnitude of average velocity and
(b) magnitude of average acceleration in 2 s.View SolutionA particle moves in a circle of radius 20 cm. Its linear speed is given by v=2t, where t is in second and v in metre/ second. Find the radial and tangential acceleration at t=3s.
View SolutionA particle moves in a circle of radius 20cm. Its linear speed is given by v=2t where t is in seconds and v in ms−1. Then
View SolutionA particle moves in a circle of radius 20 cm. Its linear speed is given by v=(3t2+5t) where t is in second and v is in m/s. Find the resultant acceleration at t = 1s.
View SolutionA particle moves in a circle of radius 30cm. Its linear speed is given by v=2t, where t in second and v in m/s. Find out its radial and tangential acceleration at t=3s.
View Solution

