A smooth sphere of radius R is made to translate line with a constant acceleration a=g. A particle kept on the top of the sphere is released from there at zero velocity with respect to the sphere. Find the speed of the particle with respect to the sphere as a function of angle θ as it slides down.
The correct Answer is:A, B, C
To solve the problem, we need to determine the speed of a particle sliding down a smooth sphere that is translating with a constant acceleration equal to g. The particle is released from the top of the sphere at zero velocity with respect to the sphere. We will use the work-energy theorem to find the speed of the particle as a function of the angle θ.
1. Identify Forces Acting on the Particle:
- The gravitational force mg acts downward.
- A pseudo force Fpseudo=ma acts horizontally in the opposite direction of the sphere's acceleration. Since a=g, this force becomes mg.
2. Set Up the Coordinate System:
- Let the center of the sphere be at the origin. When the particle is at an angle θ from the vertical, its position can be described in terms of the radius R of the sphere:
- Horizontal position: x=Rsinθ
- Vertical position: y=R(1−cosθ)
3. Calculate the Work Done:
- The work done by gravity as the particle moves down is:
Wgravity=mg⋅h=mg⋅(R(1−cosθ))
- The work done by the pseudo force as the particle moves horizontally is:
Wpseudo=Fpseudo⋅d=mg⋅(Rsinθ)
4. Apply the Work-Energy Theorem:
- According to the work-energy theorem:
Wgravity+Wpseudo=ΔKE
- The change in kinetic energy is given by:
ΔKE=12mV2−0=12mV2
- Therefore, we can write:
mg(R(1−cosθ))+mg(Rsinθ)=12mV2
5. Simplify the Equation:
- Cancel m from both sides:
g(R(1−cosθ)+g(Rsinθ))=12V2
- This simplifies to:
gR(1−cosθ+sinθ)=12V2
6. Solve for V2:
- Rearranging gives:
V2=2gR(1−cosθ+sinθ)
7. Find V:
- Taking the square root:
V=√2gR(1−cosθ+sinθ)
Final Answer:
The speed of the particle with respect to the sphere as a function of angle θ is:
V=√2gR(1−cosθ+sinθ)
- The gravitational force mg acts downward.
- A pseudo force Fpseudo=ma acts horizontally in the opposite direction of the sphere's acceleration. Since a=g, this force becomes mg.
- Let the center of the sphere be at the origin. When the particle is at an angle θ from the vertical, its position can be described in terms of the radius R of the sphere:
- Horizontal position: x=Rsinθ
- Vertical position: y=R(1−cosθ)
- The work done by gravity as the particle moves down is:
Wgravity=mg⋅h=mg⋅(R(1−cosθ))
- The work done by the pseudo force as the particle moves horizontally is:
Wpseudo=Fpseudo⋅d=mg⋅(Rsinθ)
- According to the work-energy theorem:
Wgravity+Wpseudo=ΔKE
- The change in kinetic energy is given by:
ΔKE=12mV2−0=12mV2
- Therefore, we can write:
mg(R(1−cosθ))+mg(Rsinθ)=12mV2
- Cancel m from both sides:
g(R(1−cosθ)+g(Rsinθ))=12V2
- This simplifies to:
gR(1−cosθ+sinθ)=12V2
- Rearranging gives:
V2=2gR(1−cosθ+sinθ)
- Taking the square root:
V=√2gR(1−cosθ+sinθ)
The speed of the particle with respect to the sphere as a function of angle θ is:
V=√2gR(1−cosθ+sinθ)
Topper's Solved these Questions
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -2
8 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
Similar Questions
A smooth sphere of radius R is made to translate oin a straight line with a constant acceleration a. A particle kept on the top of the sphere is released rom there at zero velocity with respect to the sphere. Find the speed of the particle with respect to the sphere as a function of the angle θ it slides.
View SolutionA smooth chute is made in a dielectric sphere of radius R and uniform volume charge density. ρ. A charge particle of mass m and charge -q is placed at the centre of the sphere. Find the time period of motion of the particle?

View Solution
Knowledge Check
A particle rests on the top of a hemishpere of radius R. Find the smallest horizontal velocity that must be imparted to the particle if it is yo leave the hemisphere without sliding down it.
A√gRB√2gRC√3gRD√5gR
A particle of mass m is kept on a fixed, smooth sphere of radius R at a position, where the radius through the particle makes an angle of 30 ∘ with the vertical. The particle is released from this position. (a) What is the force exerted by the sphere on the particle just after the release? (b) Find the distance traveled by the particle before it leaves contact with the sphere.
View SolutionA particle of mass m starts to slide down from the top of the fixed smooth sphere. What is the tangential acceleration when it break off the sphere ?
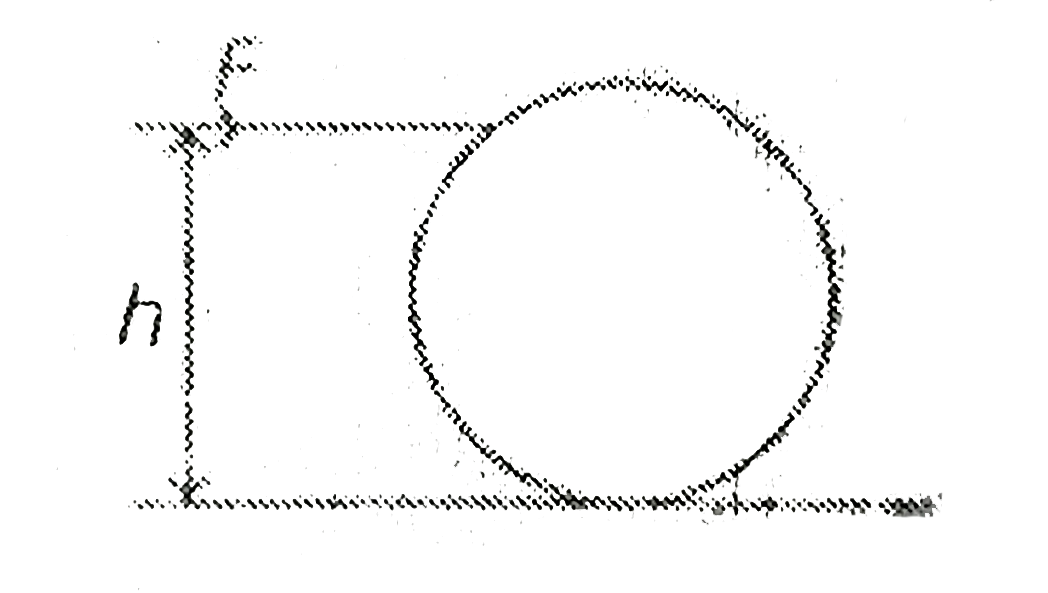
View SolutionA solid sphere of radius R is resting on a smooth horizontal surface. A constant force F is applied at a height h from the bottom. Choose the correct alternative.
View SolutionA particle is projected with velocity u horizontally from the top of a smooth sphere of radius a so that it slides down the outside of the sphere. If the particle leaves the sphere when it has fallen a height a4 , the value of u is
View SolutionA sphere is released on a smooth inclined plane from the top. When it moves down, its angular momentum is
View SolutionA ball is kept at a height h above the surface of a heavy transparent sphere made of a material of refractive index The radius of the sphere is R. At t = 0, the ball is dropped to fall normally on the sphere. Find the speed of the image formed as a function of time for t<√2hg. Consider only the image by a single refraction.
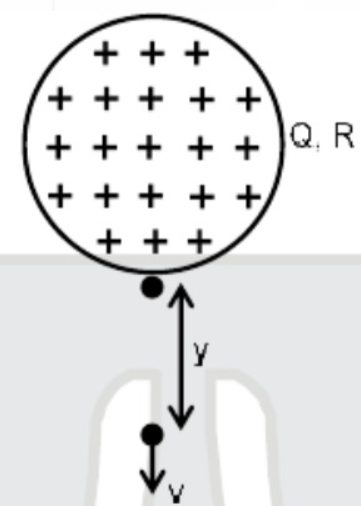
View SolutionA uniformly charged sphere of charge Q and radius R is placed at some height from ground surface and sphere is fixed. Now a charged particle of mass m and charge q is released form rest just below to sphere what will be speed of particle after travelling Y-direction
View Solution
