A kid of mass M stands at the edge of a platform of radius R which can be freely rotated about its axis. The moment of inertia of the platform is I . The system is at rest when a friend throws as ball of mass m and the kid catches it. If the velocity of the ball is v horizontally along the tangent to the edge of the platform when it was caught by the kid find the angular speed of the platform after the event.
The correct Answer is:B
To solve the problem, we will apply the principle of conservation of angular momentum. Here are the steps to find the angular speed of the platform after the kid catches the ball:
Step 1: Understand the System
Initially, the system consists of:
- A kid of mass M standing at the edge of a platform of radius R.
- The platform has a moment of inertia I.
- The system is at rest, meaning the initial angular momentum is zero.
Step 2: Identify the Initial Angular Momentum
Before the kid catches the ball, the ball of mass m is moving with a velocity v tangentially at the edge of the platform. The angular momentum Li of the ball about the center of the platform is given by:
Li=m⋅v⋅R
where R is the radius of the platform.
Step 3: Identify the Final Angular Momentum
After the kid catches the ball, the system (platform + kid + ball) rotates with an angular speed ω. The total moment of inertia If of the system after the event is:
If=I+M⋅R2+m⋅R2
where M⋅R2 is the moment of inertia of the kid and m⋅R2 is the moment of inertia of the ball (both at distance R from the axis).
Step 4: Apply Conservation of Angular Momentum
According to the conservation of angular momentum:
Li=Lf
where Lf is the final angular momentum of the system. Thus, we have:
m⋅v⋅R=(I+M⋅R2+m⋅R2)⋅ω
Step 5: Solve for Angular Speed ω
Rearranging the equation to solve for ω:
ω=m⋅v⋅RI+M⋅R2+m⋅R2
Final Answer
The angular speed of the platform after the event is:
ω=m⋅v⋅RI+(M+m)⋅R2
Initially, the system consists of:
- A kid of mass M standing at the edge of a platform of radius R.
- The platform has a moment of inertia I.
- The system is at rest, meaning the initial angular momentum is zero.
Before the kid catches the ball, the ball of mass m is moving with a velocity v tangentially at the edge of the platform. The angular momentum Li of the ball about the center of the platform is given by:
Li=m⋅v⋅R
where R is the radius of the platform.
After the kid catches the ball, the system (platform + kid + ball) rotates with an angular speed ω. The total moment of inertia If of the system after the event is:
If=I+M⋅R2+m⋅R2
where M⋅R2 is the moment of inertia of the kid and m⋅R2 is the moment of inertia of the ball (both at distance R from the axis).
According to the conservation of angular momentum:
Li=Lf
where Lf is the final angular momentum of the system. Thus, we have:
m⋅v⋅R=(I+M⋅R2+m⋅R2)⋅ω
Rearranging the equation to solve for ω:
ω=m⋅v⋅RI+M⋅R2+m⋅R2
The angular speed of the platform after the event is:
ω=m⋅v⋅RI+(M+m)⋅R2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -2
14 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
Similar Questions
A child of mass m is standing, on the periphery of a circular platform of radius R, which can rotate about its central axis. The moment of inertia of the platform is I. Child jumps off from the platform with a velocity u tangentially relative to the platform. Find the angular speed of the platform after the child jumps off.
View SolutionA sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
View Solution
Knowledge Check
The moment of inertia of a hoop of radius R and mass M, about any tangent
A[3mR2]/2B[mR2]/2CMR2D[MR2]/4Find the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
A25MR2B65MR2C45MR2D75MR2
Suppose in the previous problem, the child stays at rest on the platform and one of his friend throws a ball of mas m1 towards him with a velocity of v from a direction tangential to the platform and the child on the platform catches the ball. Find the angular velocity of the platform after he catches the ball.
View SolutionA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is

View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be

View SolutionThe moment of inertia of a cube of mass m and side a about one of its edges is equal to
View SolutionFind the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
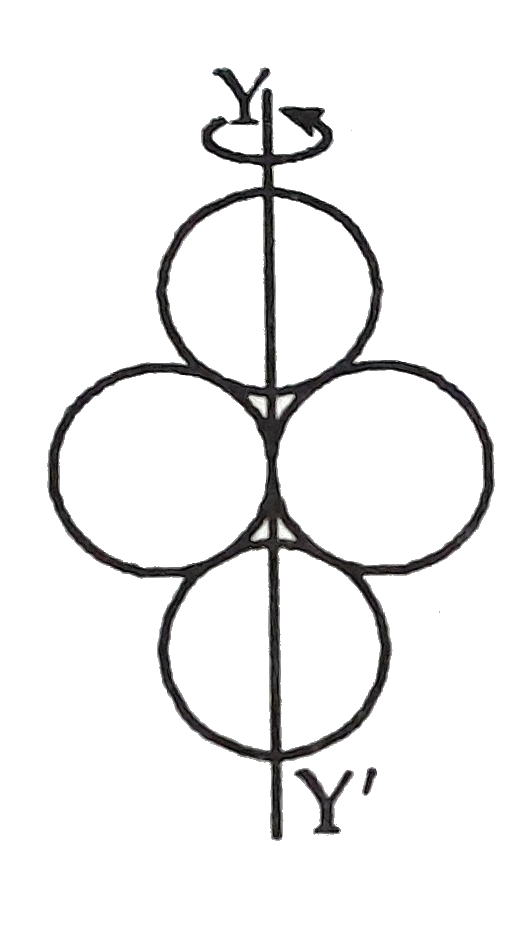
View SolutionFour rings each of mass M and radius R are arranged as shown in the figure. The moment of inertia of the system about YY' will be
View Solution
