By a surface of a liquid we mean
a geometrical plane like x=0
all molecules exposed to the atmosphere
a layer of thicknesss of the order of 10−8 m
a layer of thickneess of the order of 10−4m
To answer the question "By a surface of a liquid we mean," we need to analyze the given options and understand the concept of the surface of a liquid in relation to surface tension.
1. Understanding Surface Tension:
- Surface tension is a property of liquids that causes the surface to behave like a stretched elastic membrane. It arises due to the cohesive forces between liquid molecules.
2. Molecular Depth of Surface Tension:
- The effective range of surface tension is typically around 10 to 15 molecular layers deep from the surface of the liquid. This means that the properties of the liquid at the surface differ from those in the bulk.
3. Evaluating the Options:
- The options provided are:
1. A geometrical plane like x = 0
2. All molecules exposed to the atmosphere
3. A layer of thickness of the order of 10−8 meters
4. A layer of thickness of the order of 10−4 meters
4. Analyzing Each Option:
- Option 1: A geometrical plane like x = 0 - This does not accurately represent the physical nature of the liquid surface.
- Option 2: All molecules exposed to the atmosphere - This is not entirely correct as not all molecules at the surface are exposed to the atmosphere.
- Option 3: A layer of thickness of the order of 10−8 meters - This is consistent with the known effective depth of surface tension.
- Option 4: A layer of thickness of the order of 10−4 meters - This is too thick compared to the effective molecular depth.
5. Conclusion:
- Based on the analysis, the correct answer is Option 3: A layer of thickness of the order of 10−8 meters. This represents the surface of a liquid in the context of surface tension.
Final Answer:
By a surface of a liquid, we mean a layer of thickness of the order of 10−8 meters.
- Surface tension is a property of liquids that causes the surface to behave like a stretched elastic membrane. It arises due to the cohesive forces between liquid molecules.
- The effective range of surface tension is typically around 10 to 15 molecular layers deep from the surface of the liquid. This means that the properties of the liquid at the surface differ from those in the bulk.
- The options provided are:
1. A geometrical plane like x = 0
2. All molecules exposed to the atmosphere
3. A layer of thickness of the order of 10−8 meters
4. A layer of thickness of the order of 10−4 meters
- Option 1: A geometrical plane like x = 0 - This does not accurately represent the physical nature of the liquid surface.
- Option 2: All molecules exposed to the atmosphere - This is not entirely correct as not all molecules at the surface are exposed to the atmosphere.
- Option 3: A layer of thickness of the order of 10−8 meters - This is consistent with the known effective depth of surface tension.
- Option 4: A layer of thickness of the order of 10−4 meters - This is too thick compared to the effective molecular depth.
- Based on the analysis, the correct answer is Option 3: A layer of thickness of the order of 10−8 meters. This represents the surface of a liquid in the context of surface tension.
By a surface of a liquid, we mean a layer of thickness of the order of 10−8 meters.
Topper's Solved these Questions
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-2
7 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Question For short Anser
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
- SOUND WAVESBOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions
133 Videos
Similar Questions
When a vertical capillary of length l with a sealed upper end was brought in contact with the surface of a liquid, the level of this liquid rose to the height h. The liquid density is ρ, the inside diameter the capillary is d, the contact angle is θ, the atmospheric pressure is ρ0. Find the surface tension of the liquid. (Temperature in this process remains constant.)
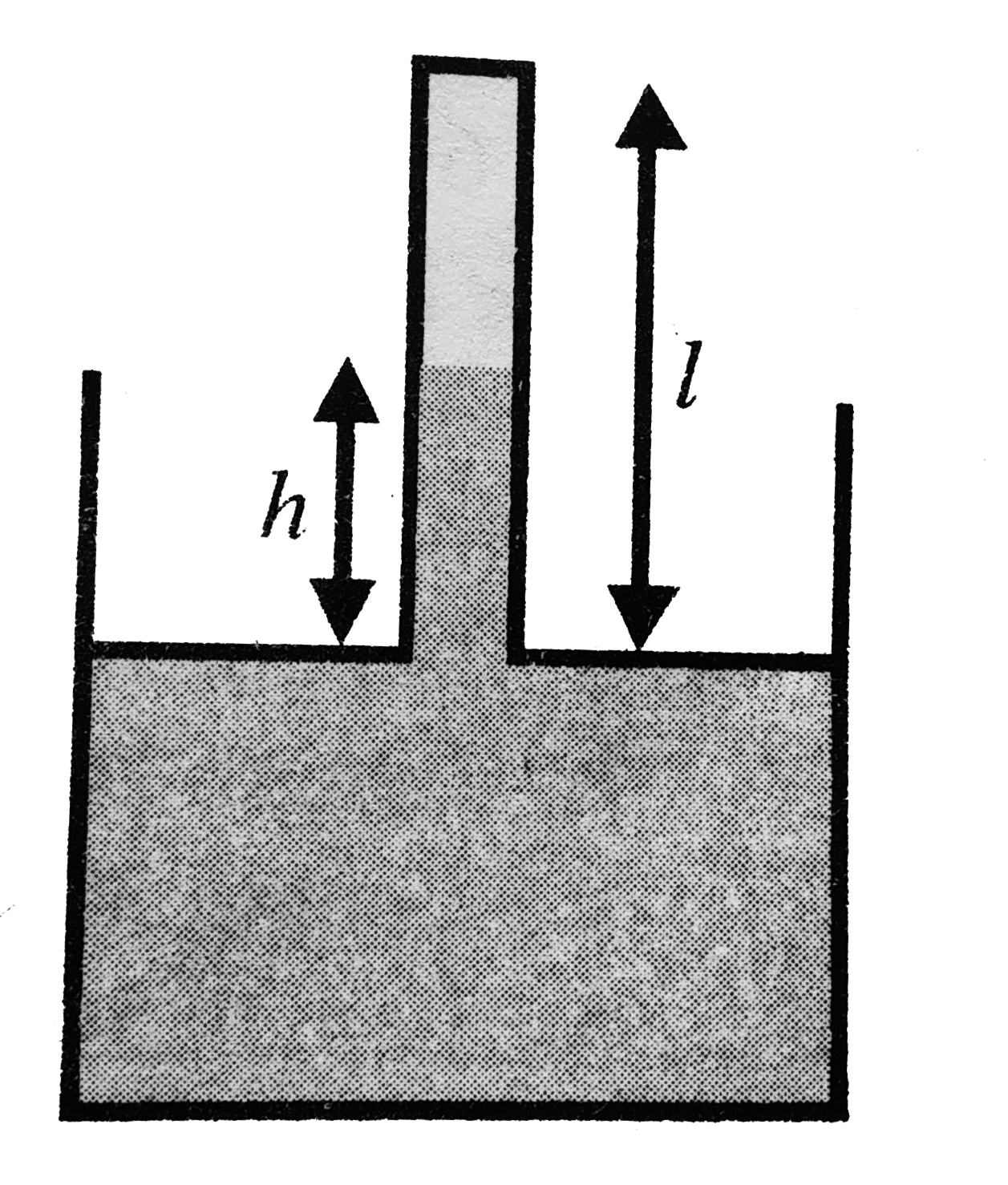
View SolutionWhen a vertical capillary of length with the sealed upper end was brought in contact with the surface of a liquid, the level of this liquid rose to the height h. The liquid density is ρ, the inside diameter the capillary is d, the contact angle is θ, the atmospheric pressure is ρ0. Find the surface tension of the liquid. (Temperature this process remains constant.)

View Solution
Knowledge Check
A body is just floating on the surface of a liquid. The density of the body is the same as that of the liquid. The body is slightly pushed down. What will happen to the body?
Acome back slowly to its earlier positionBremain submerged where it is leftCsink in liquidDcome out vigourslyThe pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth
The force of interaction between the molecules of same substance is called cohesive force hile force of interaction between molecules of different substances is called adhesive force . Both the forces are attracive and have a range of about 10 A . If we consider a molecule . A well inside a liquid we find that it is equally attracted by the surrounding molecules in all the directions ( with a sphere of radius 10 Å ) and so it does not experience any resultant force . On or near the surface of a liquid , the molecules do not exist on the free side and so a molecule ( say B ) experiences a resultant force vertically downwards into the liquid . For this reason , the molecules of the surface have a tendency to go into the liquid . Thus , the surface tends to contract and gives rise to surface tension .
As surface molecules like B experience a net downward force of cohension and have tendency to go down , so in increasing the area of the surface more molecules are to be shifted to the surface from inside . Thus , more mechnical work will have to be done against the net downward force of cohension . This work is stored by the molecules in the surfaces as potential energy called free surface energy .
Furthermore , molcules like P , in contact with the liquid will stick to the solid surface , i.e will meet it if the force of adhesion A is greater than net force of cohension C . The liquid will not the solid surface if C>A
Also , molecules like Q at the surface in contact with the solid will experience a net force of cohension CR . If adhesion A is greater then cohension CR , the resultant force will be F . As the liquid can't support tangential force , F must be perpendicular to the surface of the liquid . So , the menisus will be concave and the angle of contact will be acute (>90∘) . However , if adhesion is lesser than cohension , the resultant force F will be directed into the liquid and so the menisus ( which is perpendicular to F as liquid support tangential force ) will be convex and the angle of contact will be obtuse (>90∘)
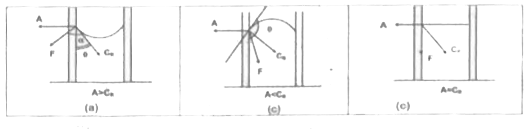
A liquid particle B , which is present in the vicinity particle A can exert a force on A if the distance of B from A is
View SolutionThe force of interaction between the molecules of same substance is called cohesive force hile force of interaction between molecules of different substances is called adhesive force . Both the forces are attracive and have a range of about 10 A . If we consider a molecule . A well inside a liquid we find that it is equally attracted by the surrounding molecules in all the dirctions ( with a sphere of radius 10 Å ) and so it does not experience any resultant force . On or near the surface of a liquid , the molecules do not exist on the free side and so a molecule ( say B ) experiences a resultant force vertically downwards into the liquid . For this reason , the molecules of the surface have a tendency to go into the liquid . Thus , the surface tends to contract and gives rise to surface tension .
As surface molecules like B experience a net downward force of cohension and have tendency to go down , so in increasing the area of the surface more molecules are to be shifted to the surface from inside . Thus , more mechnical work will have to be done against the net downward force of cohension . This work is stored by the molecules in the surfaces as potential energy called free surface energy .
Furthermore , molcules like P , in contact with the liquid will stick to the solid surface , i.e will meet it if the force of adhesion A is greater than net force of cohension C . The liquid will not the solid surface if C>A
Also , molecules like Q at the surface in contact with the solid will experience a net force of cohension CR . If adhesion A is greater then cohension CR , the resultant force will be F . As the liquid can't support tangential force , F must be perpendicular to the surface of the liquid . So , the menisus will be concave and the angle of contact will be acute (>90∘) . However , if adhesion is lesser than cohension , the resultant force F will be directed into the liquid and so the menisus ( which is perpendicular to F as liquid support tangential force ) will be convex and the angle of contact will be obtuse (>90∘)
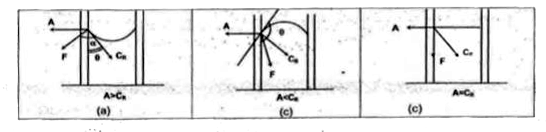
The free suface energy , stored in the surface is because of work done
View SolutionA : The apparent weight of a body floating on the surface of a liquid is zero.
R : The net force on a body floating on the surface of a liquid is zero.
View SolutionThe wettability of a surface by a liquid depends primarily on
View SolutionWhy the surface of a liquid act like a stretched membrane?
View SolutionThe time period (T) of small oscillations of the surface of a liquid drop depends on its surface tension (s), the density (ρ) of the liquid and it's mean radius (r) as T=csxpyrz. If in the measurement of the mean radius of the drop, the error is 2%, the error in the measurement of surface tension and density both are of 1%. Find the percentage error in measurement of the time period.
View Solution
