A 20 cm long capillary tube is dipped in water. The water rises up to 8 cm. If the entire arrangement is put in a freely falling elevator, the length of water column in the capillary tube will be
8 cm
6 cm
10 cm
20 cm
To solve the problem, we need to understand the concept of capillary action and how it is affected by gravity.
1. Understanding Capillary Rise: When a capillary tube is dipped in water, the water rises due to the adhesive forces between the water molecules and the walls of the tube, as well as the cohesive forces between the water molecules themselves. The height to which the water rises (h) can be calculated using the formula:
h=2scosθρrg
where s is the surface tension of the liquid, θ is the contact angle, ρ is the density of the liquid, r is the radius of the tube, and g is the acceleration due to gravity.
2. Initial Conditions: In this case, the capillary tube is 20 cm long, and the water rises to a height of 8 cm when the tube is in a stationary position under normal gravity.
3. Effect of Free Fall: When the entire arrangement is placed in a freely falling elevator, the effective acceleration due to gravity (g) becomes zero. This is because both the tube and the water inside it are falling at the same rate, creating a condition of weightlessness.
4. Analyzing the Capillary Rise Formula: With g=0, the formula for capillary rise becomes:
h=2scosθρr⋅0
Since the denominator becomes zero, the height h theoretically approaches infinity.
5. Maximum Length of Water Column: However, in practical terms, the water can only rise to the maximum length of the capillary tube. Therefore, the water will fill the entire length of the tube.
6. Conclusion: Since the maximum length of the capillary tube is 20 cm, the length of the water column in the capillary tube when in a freely falling elevator will be 20 cm.
Final Answer:
The length of the water column in the capillary tube will be 20 cm.
---
h=2scosθρrg
where s is the surface tension of the liquid, θ is the contact angle, ρ is the density of the liquid, r is the radius of the tube, and g is the acceleration due to gravity.
h=2scosθρr⋅0
Since the denominator becomes zero, the height h theoretically approaches infinity.
The length of the water column in the capillary tube will be 20 cm.
Topper's Solved these Questions
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-2
7 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Question For short Anser
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
- SOUND WAVESBOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions
133 Videos
Similar Questions
In a capillary tube experiment, a vertical 30 cm long capillary tube is dipped in water. The water rises up to a height of 10 cm due to capillary action. If this experiment is conducted in a freely falling elevator, the length of te water column becomes
View SolutionIf a wax coated capillary tube is dipped in water, then water in it will-
View Solution
Knowledge Check
By inserting a capillary tube into a depht l in water, the water rises to a height h. If the lower end of the capillary is closed inside water and the capillary is closed inside water and the capillaryis taken out and closed and opened, to what height the water will remain in the tube ? Here l>h
AzeroBl+HC2 hDh
The height to which water rises in a capillary will be
View SolutionThe lower end of a glass capillary tube is dipped in water. Water rises to a height of 9 cm. The tube is then broken at a height of 5 cm. The height of the water column and angle of contact will be
View SolutionIn a surface tension experiment with a capillary tube water rises upto 0.1m. If the same experiment is repeated in an artificial satellite, which is revolving around the earth, water will rise in the capillary tube upto a height of
View SolutionWater rises in a vertical capillary tube up to a length of 10 cm. If the tube is inclined at 45∘, the length of water risen in the tube will be
View SolutionFigure shows a capillary tube of radius r dipped into The atmospheric pressure is P0 and the capillary rise of water is h. s is the surface tension for water-glass.
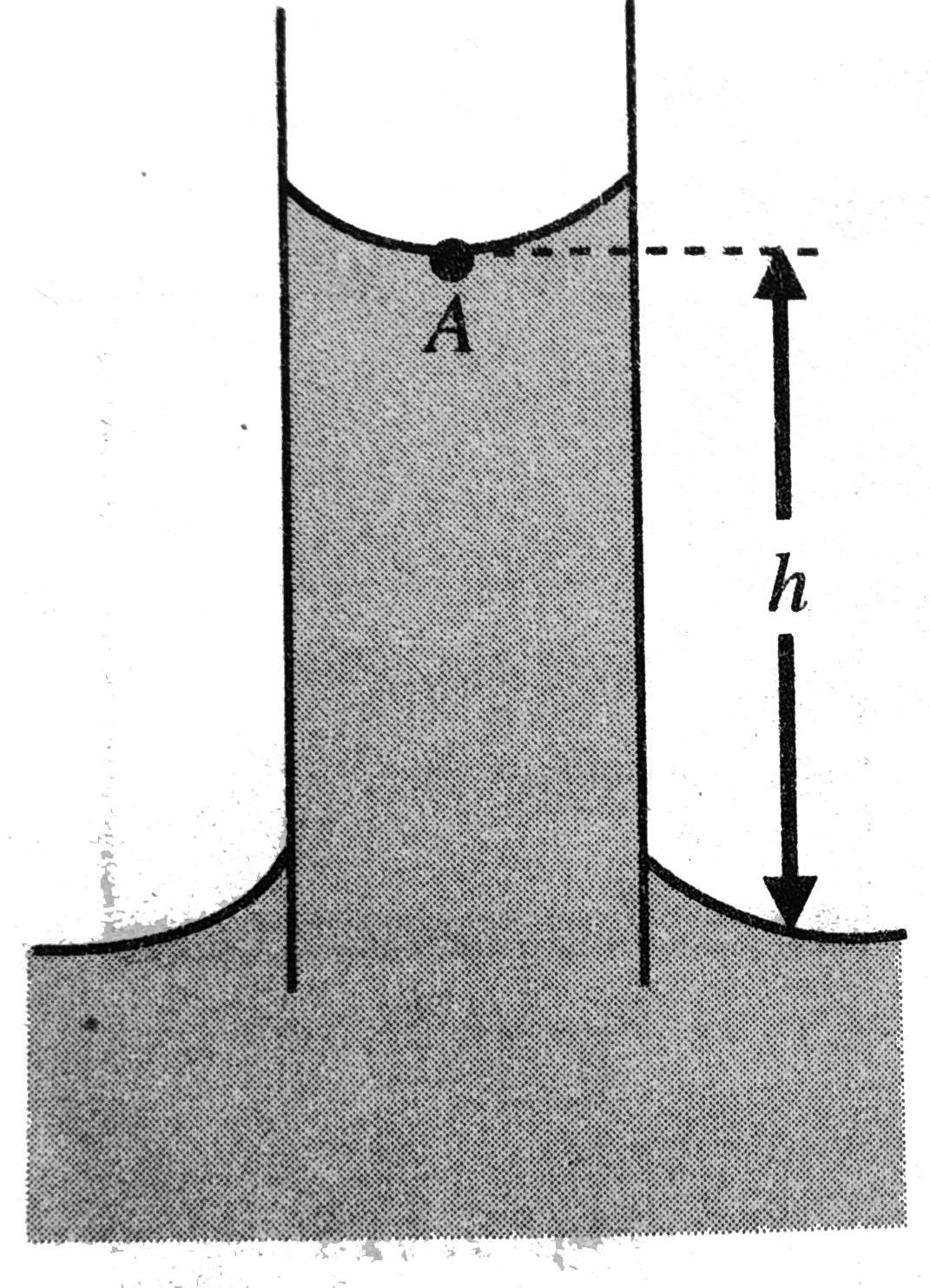
Initially, h=10cm. If the capillary tube is now incline at 45∘, the length of water rising in the tube will be
View SolutionIf a capillary tube of radius 1 mm is immersed in water , the mass of water rising in the capillary tube is M . If the radius tube is doubled , then the mass of water , that rises in the capillary tube will be
View SolutionIn a capillary tube having area of cross - section A, water rises to a height h. If cross-sectional area is reduced to A9, the rise of water in the capillary tube is
View Solution
