A wave travels along the positive x-direction with a speed of 20ms−1. The amplitude of the wave is 0.20 cm and the wavelength 2.0 cm. (a) Write a suitable wave equation which describes this wave. (b) What is the displacement and velocity of the particle at x = 2.0 cm at time t = 0 according to the wave equation written ?
The correct Answer is:A, B, C, D
To solve the problem step by step, we will address both parts of the question.
Part (a): Writing the Wave Equation
1. Identify Given Values:
- Speed of the wave, V=20m/s
- Amplitude, A=0.20cm=0.20×10−2m
- Wavelength, λ=2.0cm=2.0×10−2m
2. Calculate the Wave Number k:
k=2πλ=2π2.0×10−2=2π0.02=100πm−1
3. Calculate the Angular Frequency ω:
- First, find the frequency ν:
ν=Vλ=20m/s2.0×10−2m=1000Hz
- Now calculate ω:
ω=2πν=2π×1000s−1=2000πs−1
4. Write the Wave Equation:
The general form of the wave equation traveling in the positive x-direction is:
y(x,t)=Asin(kx−ωt)
Substituting the values of A, k, and ω:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
Part (b): Finding Displacement and Velocity at x=2.0cm and t=0
1. Substituting Values into the Wave Equation:
- Convert x=2.0cm to meters:
x=2.0cm=2.0×10−2m
- Substitute x and t=0 into the wave equation:
y(2.0×10−2,0)=(0.20×10−2)sin(100π×2.0×10−2−2000π×0)
=(0.20×10−2)sin(2π)=(0.20×10−2)×0=0
2. Finding the Velocity of the Particle:
- The velocity v is given by:
v=dydt=−Aωcos(kx−ωt)
- Substitute A, ω, k, x, and t=0:
v=−(0.20×10−2)(2000π)cos(100π×2.0×10−2−2000π×0)
=−(0.20×10−2)(2000π)cos(2π)
=−(0.20×10−2)(2000π)×1=−0.20×2000π×10−2
=−4πm/s
Final Answers:
- (a) The wave equation is:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
- (b) The displacement at x=2.0cm and t=0 is 0m and the velocity is −4πm/s.
- Speed of the wave, V=20m/s
- Amplitude, A=0.20cm=0.20×10−2m
- Wavelength, λ=2.0cm=2.0×10−2m
k=2πλ=2π2.0×10−2=2π0.02=100πm−1
- First, find the frequency ν:
ν=Vλ=20m/s2.0×10−2m=1000Hz
- Now calculate ω:
ω=2πν=2π×1000s−1=2000πs−1
The general form of the wave equation traveling in the positive x-direction is:
y(x,t)=Asin(kx−ωt)
Substituting the values of A, k, and ω:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
- Convert x=2.0cm to meters:
x=2.0cm=2.0×10−2m
- Substitute x and t=0 into the wave equation:
y(2.0×10−2,0)=(0.20×10−2)sin(100π×2.0×10−2−2000π×0)
=(0.20×10−2)sin(2π)=(0.20×10−2)×0=0
- The velocity v is given by:
v=dydt=−Aωcos(kx−ωt)
- Substitute A, ω, k, x, and t=0:
v=−(0.20×10−2)(2000π)cos(100π×2.0×10−2−2000π×0)
=−(0.20×10−2)(2000π)cos(2π)
=−(0.20×10−2)(2000π)×1=−0.20×2000π×10−2
=−4πm/s
- (a) The wave equation is:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
- (b) The displacement at x=2.0cm and t=0 is 0m and the velocity is −4πm/s.
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -2
9 Videos
- THE FORCESBOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer
9 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
Similar Questions
Transverse waves on a srting have speed 12.0m/s, amplitude 0.05m and wavelength 0.4m. The waves travel in the +x−direction and at t=0 the x=0 end of the string has zero displacement and is moving upwards.
(a) Write a wave function describing the wave.
(b) Find the transverse displacement of a point at x=0.25m at time t=0.15s.
( c ) How much time must elapse from the instant in part (b) until the point at x=0.25m has zero dispacement?
View SolutionA wave propagates in a string in the positive x-direction with velocity v. The shape of the string at t=t0 is given by
f(x,t0)=Asin(x2a2). Then the wave equation at any instant t is given by
View SolutionA wave travelling on a string at a speed of 10ms−1 causes each particle of the string to oscillate with a time period of 20 ms. (a) What is the wavelength of the wave ? (b) If the displacement of a particle is 1.5 mm at a certain instant, what will be the displacement of a particle 10 cm away from it at the same instant ?
View SolutionA wave in a string has an amplitude of 2cm. The wave travels in the +ve direction of x axis with a speed of 128ms−1 and it is noted that 5 complete waves fit in 4m length of the string. The equation describing the wave is
View SolutionA certain transverse sinusoidal wave of wavelength 20 cm is moving in the positive x direction. The transverse velocity of the particle at x=0 as a function of time is shown. The amplitude of the motion is:
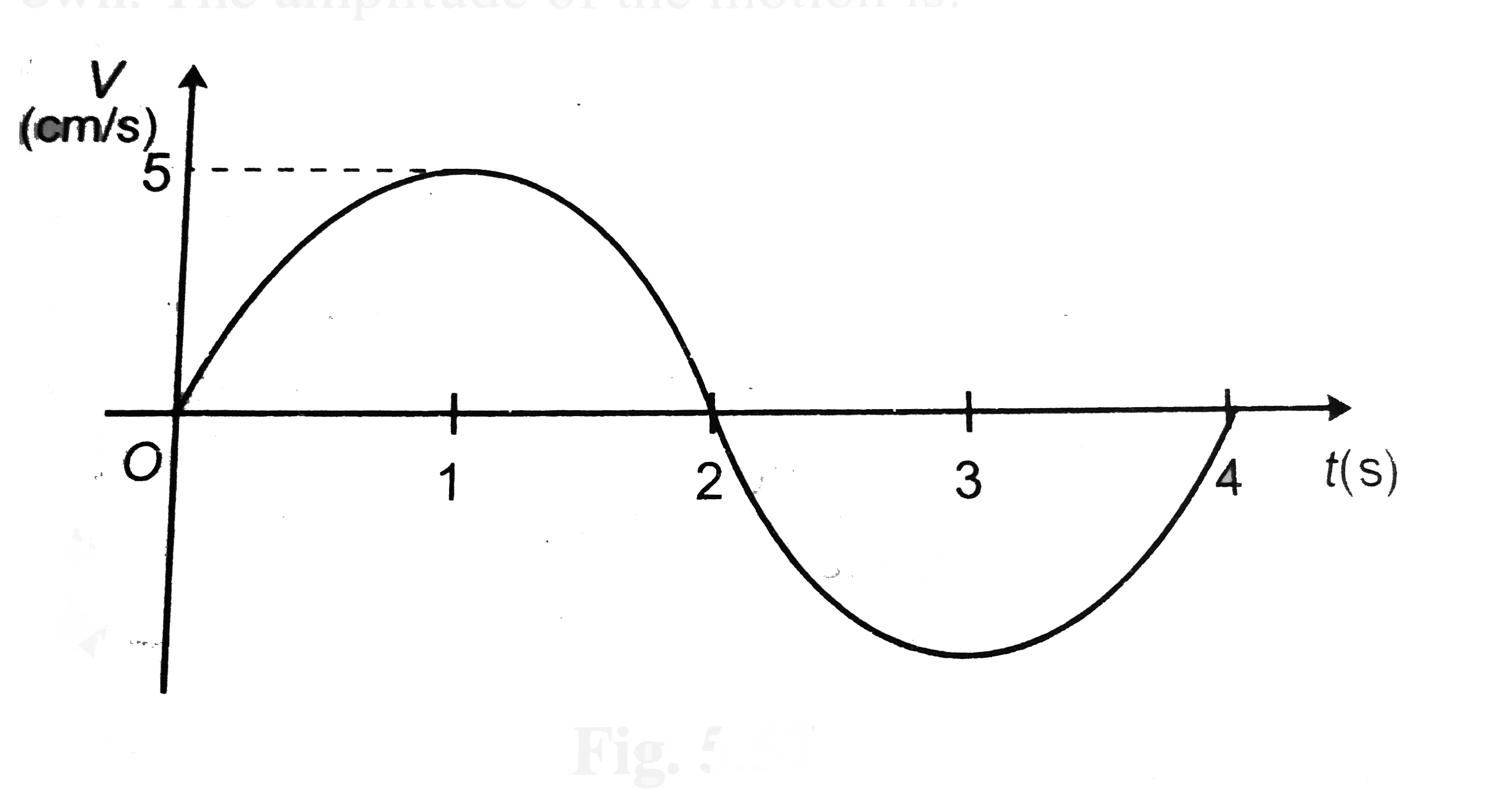
View SolutionA certain transverse sinusoidal wave of wavelength 20 cm is moving in the positive x direction. The transverse velocity of the particle at x=0 as a function of time is shown. The amplitude of the motion is:

View SolutionA sinusoidal wave trsvelling in the positive direction on a stretched string has amplitude 2.0cm, wavelength 1.0m and velocity 5.0m/s. At x=0 and t=0 it is given that y=0 and ∂y∂t<0. Find the wave function y(x,t).
View SolutionA transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. the transverse displacement of the string particle at x=0 as a function of time is shown in figure.
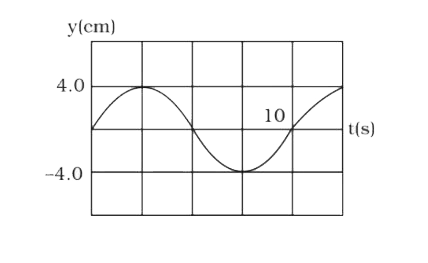
Q. The velocity of propagation of the wave is ?
View SolutionA wave of wavelength 0.60 cm is produced in air and it travels at a speed of 300 ms^-1. Will it be audible?
View SolutionA sinusoidal wave travelling in the positive x-direction has an amplitude of 15.0cm, a wavelength of 40.0cm and a frequency of 8.00Hz.Thevertic≤displacementofthemediumatt = 0 and x = 0isalso15.0 cmasshown∈figure.<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/DCP_V03_C17_E01_054_Q01.pngwh=80%mx-autosty≤=background:white;><br>(a)F∈dtheanga––rwaveνmberk,periodT,anga––requencyomegaandspeedv of the wave.
(b) Write a general expression for wave function.
View Solution
