A thin prism of crown glass (μr=1.515,μv=1.525) and a thin prism of flint glass (μr=1.612,μv=1.632) are placed in contact with each other. Their refracting angles are 5.0∘ each and are similarly directed. Calculate the angular dispersion produced by the combination.
The correct Answer is:A
To solve the problem of calculating the angular dispersion produced by the combination of a thin prism of crown glass and a thin prism of flint glass, we can follow these steps:
Step 1: Understand the Problem
We have two prisms:
- Crown glass with refractive indices:
- μr=1.515 (for red light)
- μv=1.525 (for violet light)
- Flint glass with refractive indices:
- μr=1.612 (for red light)
- μv=1.632 (for violet light)
Both prisms have a refracting angle A=5∘.
Step 2: Calculate the Deviation for Each Prism
The deviation δ produced by a prism can be calculated using the formula:
δ=(μ−1)⋅A
where μ is the refractive index of the prism for the specific color of light, and A is the angle of the prism.
Step 3: Calculate the Deviation for Violet Light
For the crown glass (violet light):
δC=(μvC−1)⋅A=(1.525−1)⋅5∘=0.525⋅5∘=2.625∘
For the flint glass (violet light):
δF=(μvF−1)⋅A=(1.632−1)⋅5∘=0.632⋅5∘=3.16∘
Step 4: Calculate the Total Deviation for Violet Light
The total deviation for violet light when both prisms are placed in contact:
δV=δC+δF=2.625∘+3.16∘=5.785∘
Step 5: Calculate the Deviation for Red Light
For the crown glass (red light):
δC=(μrC−1)⋅A=(1.515−1)⋅5∘=0.515⋅5∘=2.575∘
For the flint glass (red light):
δF=(μrF−1)⋅A=(1.612−1)⋅5∘=0.612⋅5∘=3.06∘
Step 6: Calculate the Total Deviation for Red Light
The total deviation for red light:
δR=δC+δF=2.575∘+3.06∘=5.635∘
Step 7: Calculate the Angular Dispersion
The angular dispersion D is given by:
D=δV−δR
Substituting the values we calculated:
D=5.785∘−5.635∘=0.15∘
Final Answer
The angular dispersion produced by the combination of the two prisms is 0.15∘.
---
We have two prisms:
- Crown glass with refractive indices:
- μr=1.515 (for red light)
- μv=1.525 (for violet light)
- Flint glass with refractive indices:
- μr=1.612 (for red light)
- μv=1.632 (for violet light)
Both prisms have a refracting angle A=5∘.
The deviation δ produced by a prism can be calculated using the formula:
δ=(μ−1)⋅A
where μ is the refractive index of the prism for the specific color of light, and A is the angle of the prism.
For the crown glass (violet light):
δC=(μvC−1)⋅A=(1.525−1)⋅5∘=0.525⋅5∘=2.625∘
δF=(μvF−1)⋅A=(1.632−1)⋅5∘=0.632⋅5∘=3.16∘
The total deviation for violet light when both prisms are placed in contact:
δV=δC+δF=2.625∘+3.16∘=5.785∘
For the crown glass (red light):
δC=(μrC−1)⋅A=(1.515−1)⋅5∘=0.515⋅5∘=2.575∘
δF=(μrF−1)⋅A=(1.612−1)⋅5∘=0.612⋅5∘=3.06∘
The total deviation for red light:
δR=δC+δF=2.575∘+3.06∘=5.635∘
The angular dispersion D is given by:
D=δV−δR
Substituting the values we calculated:
D=5.785∘−5.635∘=0.15∘
The angular dispersion produced by the combination of the two prisms is 0.15∘.
Topper's Solved these Questions
- DISPERSION AND SPECTRABOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Question for short Answer
6 Videos
- DISPERSION AND SPECTRABOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Objective -2
5 Videos
- CAPACITORSBOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - short answer
7 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - questions for short answer
17 Videos
Similar Questions
A thin prism of angle 6.0∘,ω'=0.07andμy'=1.50 is combined with another thin prism having ω=0.08andμy=1.60. The combination produces no deviation in the mean ray. (a) Find the angle of the second prism. (b) Find the net angular dispersion produced by the combination when a beam of white light passes through it. (c) If the prisms are similarly directed, what will be the deviation in the mean ray ? (d) Find the angular dispersion in the situation described in (c).
View SolutionThe refractive index of a material M1 changes by 0.014 and that of another material M2 changes by 0.024 as the colour of the light is changed from red to violet. Two thin prisms one made of M1(A=5.3∘) and other made of M2(A=3.7∘) are combined with their refracting angles oppositely directed.
(a) Find the angular dispesion produced by the combination.
(b) the prisms are now combined with their refracting angles similarly directed. Find the angular dispersion produced by the combination.
View Solution
Knowledge Check
A small angled prism (μ=1.62) gives a deviation of 4.8. Calculate the angle of prism.
A5∘B6.36∘C3∘D7.74∘
A prism of glass (μ=1.5) is dipped in to water as shown in the figure. If the refractive index of water is 4/3, then the incident ray will be totally reflected if

View SolutionTwo prisms A and B have dispersive powers of 0.012 and 0.018 respectively. The two prisms are in contact with each other. The prism A produces a mean deviation of 1.2∘, the mean deviation produced by B if the combination is achromatic is
View SolutionA crown glass prism of angle 5∘ is to be combined with a flint glass prism in such a way that the mean ray passes undeviated. Find (a) the angle of the flint glass prism needed and (b) the angular dispersion produced by the combination when white light goes through it. Refractive indices for red, yellow and viloet light are 1.514, 1.517 and 1.523 respectively for crown glass and 1.613 1.620 and 1.632 for flint glass.
View SolutionWe have to combine a crown glass prism of angle 4∘ with a flint glass prism in such a way that the mean ray passes undeviated, find (i) the angle of the flint glass prism needed and (i) the angular dispersion produced by the combination when white light goes through it. Refractive indices for red, yellow and violet light are 1.514, 1.517 and 1.523 respectively for crown glass and 1.613, 1.620 and 1.632 for flint glass.
View SolutionThree thin prisms are combined as shown in figure. The refractive indices of the crown glass for red, yellow and violet rays are μr,μyandμv respectively and those for the flint glass are μ′r,μ′yandμ′v respectively. Find the ratio A'/A for which (a) there is no net angular dispersion, and (b) there is no net deviation in the yellow ray.
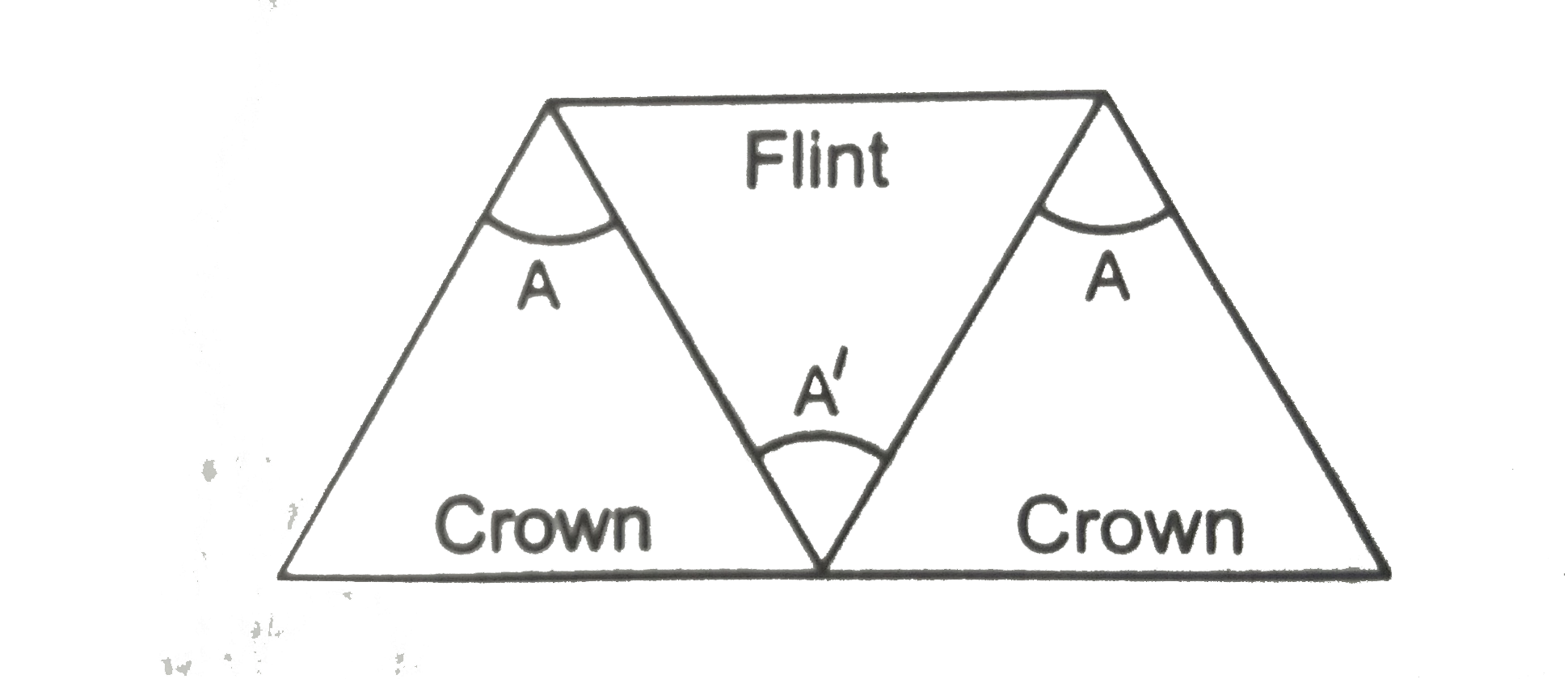
View SolutionA crown glass prism of angle 5∘ is to be combined with a flint prism in such a way that the mean ray passes undeviated. Find (a) the angle of the flint glass prism needed and (b) the angular dispersion produced by the combinatino when white light goes through it. Refractive indices for red, yellow, and violet light are 1.541, 1.517 and 1.523, repectively, for crown flass and 1.613, 1.620 and 1.632 for flint glass.
View SolutionIn a thin prism of glass (refractive index 1.5), which of the following relations between the angle of minimum deviations δm and angle of prism r will be correct?
View Solution
