Suppose you have three resistor each of value (30Ω).List all the different resistances you can obtian using them.
To find all the different resistances that can be obtained using three resistors, each of value 30Ω, we can explore various configurations: series, parallel, and a combination of both. Here’s a step-by-step solution:
Step 1: Calculate Resistance in Series
When resistors are connected in series, the total resistance Rs is the sum of the individual resistances.
Rs=R1+R2+R3
For three resistors of 30Ω:
Rs=30+30+30=90Ω
Step 2: Calculate Resistance in Parallel
When resistors are connected in parallel, the total resistance Rp can be calculated using the formula:
1Rp=1R1+1R2+1R3
For three resistors of 30Ω:
1Rp=130+130+130=330=110
Thus,
Rp=10Ω
Step 3: Calculate Resistance with Two in Parallel and One in Series
Now, let’s consider two resistors in parallel and the third one in series with them.
1. Calculate the equivalent resistance of the two resistors in parallel Rparallel:
1Rparallel=130+130=230=115
Thus,
Rparallel=15Ω
2. Now add the third resistor in series:
Rtotal=Rparallel+R3=15+30=45Ω
Summary of Results
The different resistances that can be obtained using three resistors of 30Ω are:
1. 90 \, \Omega (all in series)
2. 10 \, \Omega (all in parallel)
3. 45 \, \Omega (two in parallel and one in series)
Final Answer
The resistances that can be obtained are:
- 90Ω
- 10Ω
- 45Ω
When resistors are connected in series, the total resistance Rs is the sum of the individual resistances.
When resistors are connected in parallel, the total resistance Rp can be calculated using the formula:
Now, let’s consider two resistors in parallel and the third one in series with them.
The different resistances that can be obtained using three resistors of 30Ω are:
2. 10 \, \Omega (all in parallel)
3. 45 \, \Omega (two in parallel and one in series)
The resistances that can be obtained are:
- 90Ω
- 10Ω
- 45Ω
Topper's Solved these Questions
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Exercises
84 Videos
- DISPERSION AND SPECTRABOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Question for short Answer
6 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Short answer
6 Videos
Similar Questions
Suppose you have three resistor of (20Ω),(50Ω)and(100Ω).what minimum and maximum resistances can you obtain from these resistors?
View SolutionIf a person has five resistors each of value 1/5 ohm, then the maximum resistance he can obtain by connecting them is
(A) 1 ohm
(B) 5 ohm
(C) 10 ohm
(D) 25 ohm
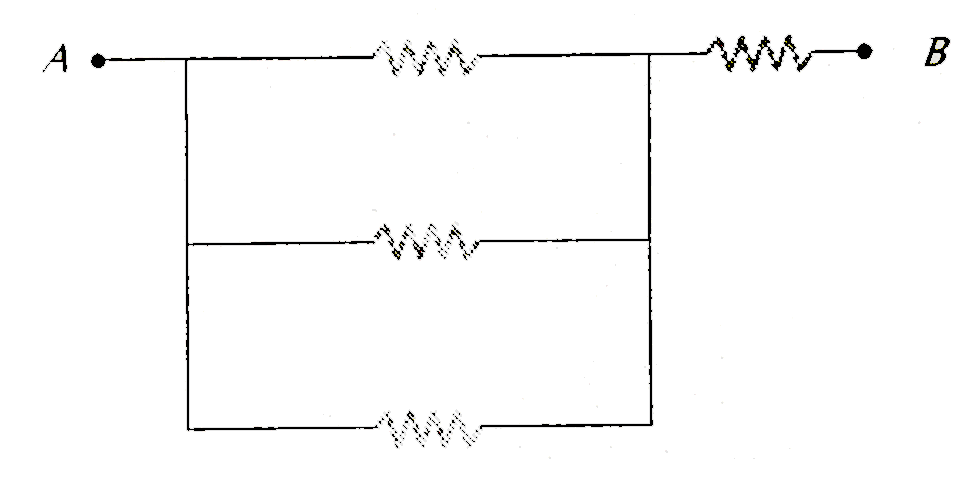
View SolutionIf all the resistors shown have the value 2 ohm each, the equivalent resistance over AB is
View SolutionShow how you would connect three resistors, each of 6Ω, so that the combination has a resistance of (a) 9 Ω, (b) 4 Ω.
View SolutionShow how you would connect three resistors, each of resistance 6Ω, so that the combination has a resistance of
(i) 9Ω
(ii) 2Ω.
View SolutionYou have three resistors of values 2Ω,3Ωand5Ω. How will you join them so that the total resistance is more than 7Ω?
Calculate the equivalent resistance.
View SolutionYou have three resistors of values 2Ω,3Ωand5Ω. How will you join them so that the total resistance is more than 7Ω?
Draw a diagram for the arrangement.
View SolutionYou have three resistors of values 2Ω,3Ω and 5 Ω. How will you join them so that the total resistance is less than 1 Ω ? Draw a diagram and find the total resistance.
View SolutionA set of n identical resistors, each of resistance RΩ, when connected in series have an effective resistance XΩ and when the resistors are connected in parallel, their effective resistance is YΩ. Find the relation between R, X and Y.
View SolutionFor the series combination of three resistors establish the relation :
R=R1+R2+R3
where the symbols have their usual meanings.
Calculate the equivalent resistance of the combination of three resistors of 6Ω,9Ω and 18Ω joined in parallel.
View Solution
