the pressure of a gas change linearly with volume from 10kPa, 200 cc to 50 kPa, 50 cc. (a) calculate the work done by the gas, (b) If no heat is supplied or extracted from the gas, what is the change in the internal energy of the gas ?
The correct Answer is:A, B, D
To solve the problem step by step, we will break it down into two parts as per the question.
Part (a): Calculate the Work Done by the Gas
1. Identify the Points: We have two points given:
- Point 1: Pressure P1=10kPa and Volume V1=200cc (which is 200×10−6m3)
- Point 2: Pressure P2=50kPa and Volume V2=50cc (which is 50×10−6m3)
2. Convert Units: Convert pressures from kPa to Pa:
- P1=10×103Pa=10,000Pa
- P2=50×103Pa=50,000Pa
3. Draw the P-V Diagram: Plot the points on a graph where the x-axis is Volume and the y-axis is Pressure. The points will form a trapezium.
4. Calculate the Area Under the Curve: The work done by the gas is equal to the area under the P-V curve. The area of a trapezium is given by:
Area=12×(P1+P2)×(V2−V1)
5. Substitute Values:
Area=12×(10,000+50,000)×(50×10−6−200×10−6)
=12×60,000×(−150×10−6)
=12×60,000×−0.00015
=−4.5J
6. Interpret the Result: The negative sign indicates that work is done on the system.
Part (b): Change in Internal Energy of the Gas
1. Use the First Law of Thermodynamics: The first law states:
ΔU=Q−W
where ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system.
2. Given Conditions: Since no heat is supplied or extracted from the gas, Q=0.
3. Substitute Values:
ΔU=0−(−4.5)=4.5J
4. Conclusion: The change in internal energy of the gas is 4.5J.
Summary of Results
- (a) The work done by the gas is −4.5J.
- (b) The change in internal energy of the gas is 4.5J.
- Point 1: Pressure P1=10kPa and Volume V1=200cc (which is 200×10−6m3)
- Point 2: Pressure P2=50kPa and Volume V2=50cc (which is 50×10−6m3)
- P1=10×103Pa=10,000Pa
- P2=50×103Pa=50,000Pa
Area=12×(P1+P2)×(V2−V1)
Area=12×(10,000+50,000)×(50×10−6−200×10−6)
=12×60,000×(−150×10−6)
=12×60,000×−0.00015
=−4.5J
ΔU=Q−W
where ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system.
ΔU=0−(−4.5)=4.5J
- (a) The work done by the gas is −4.5J.
- (b) The change in internal energy of the gas is 4.5J.
Topper's Solved these Questions
Similar Questions
400 J of work is done on a gas to reduce its volume by compression adiabatically. What is the change in internal energy of the gas?
View SolutionWork done by a gas in a given process is −20J. Heat given to the gas is 60J. Find change in internal energy of the gas.
View SolutionA gas is taken along the path AB as shown in fig, if 70 cal of heat is extracted from the gas in the process, calculate the change in the internal energy of the system.
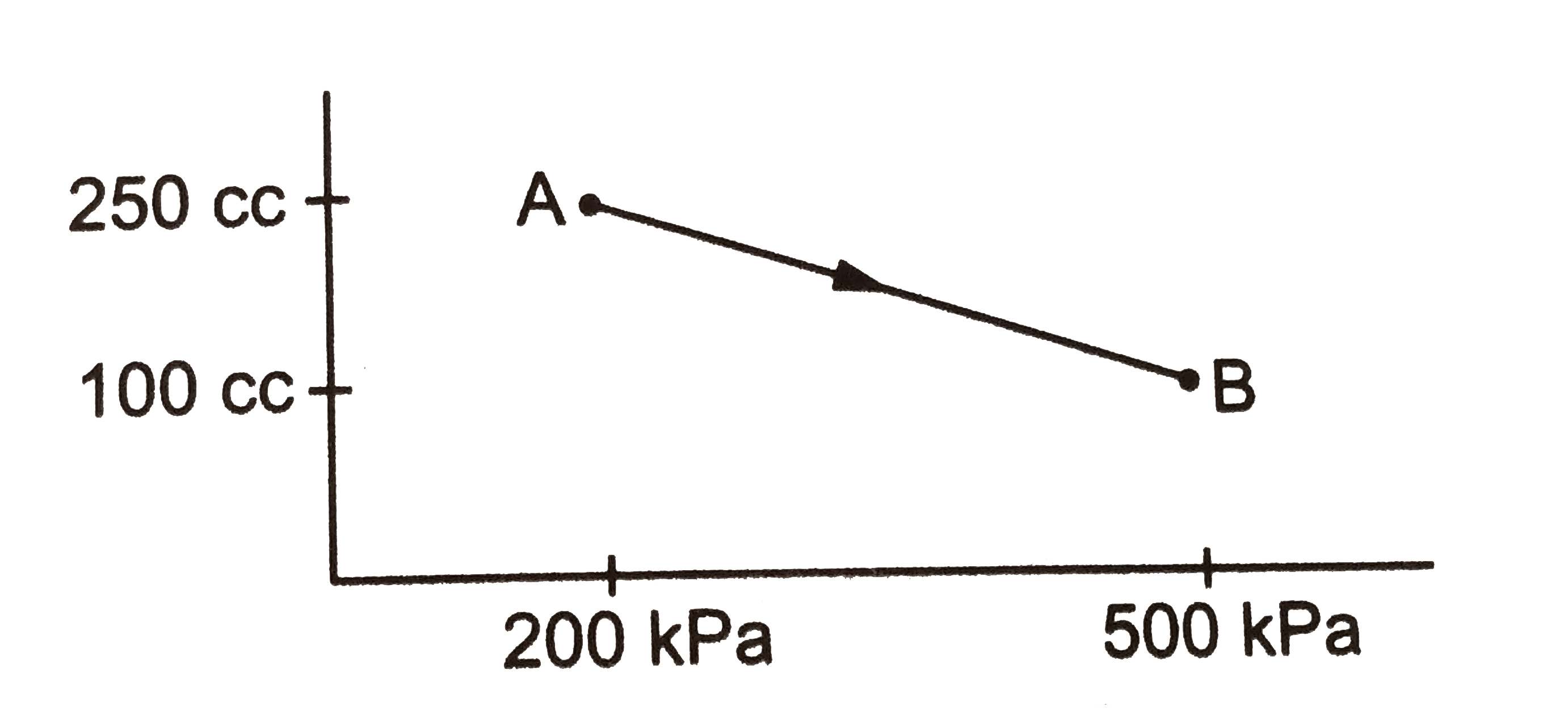 View Solution
View SolutionA sample of ideal gas (y = 1 .4) is heated at constant pressure. If an amount of 140 J heat is supplied to the gas, then change in internal energy of the gas
View SolutionIn a cyclic process shown in the figure an ideal gas is adiabatically taken from BtoA, the work done on the gas during the process BtoA is 30,J when the gas is taken from A to B the heat absorbed by the gas is 20J Then change in internal energy of the gas in the process A to B is :
 View Solution
View SolutionIn a cyclic process shown in the figure an ideal gas is adiabatically taken from BtoA, the work done on the gas during the process BtoA is 30,J when the gas is taken from A to B the heat absorbed by the gas is 20J Then change in internal energy of the gas in the process A to B is :
 View Solution
View SolutionIn a cyclic process shown in the figure an ideal gas is adiabatically taken from BtoA, the work done on the gas during the process BtoA is 30,J when the gas is taken from A to B the heat absorbed by the gas is 20J Then change in internal energy of the gas in the process A to B is :
 View Solution
View SolutionWhen an ideal gas is heated at constant pressure, the fraction of the heat energy supplied whicn increases the internal energy of the gas is :
View SolutionWhen an ideal diatomic gas is heated at constant pressure fraction of the heat energy supplied which increases the internal energy of the gas is
View SolutionA diatomic gas is heated at constant pressure. If 105J of heat is given to the gas, find (a) the change in internal energy of the gas (b) the work done by the gas.
View Solution

