Can we define specific heat capacity at constant temperature?
To solve the question "Can we define specific heat capacity at constant temperature?", we can follow these steps:
Step 1: Understand the Definition of Specific Heat Capacity
Specific heat capacity (C) is defined as the amount of heat (Q) required to change the temperature of a unit mass (n) of a substance by one degree Celsius (or one Kelvin). Mathematically, this is expressed as:
Q=nCΔT
where:
- Q = heat added or removed
- n = mass of the substance
- C = specific heat capacity
- ΔT = change in temperature
Step 2: Analyze the Case of Constant Temperature
In a constant temperature process, the temperature does not change. Therefore, the change in temperature (ΔT) is equal to zero:
ΔT=0
Step 3: Substitute into the Heat Equation
Substituting ΔT=0 into the heat equation gives:
Q=nC(0)
This simplifies to:
Q=0
Step 4: Implications for Specific Heat Capacity
Since Q=0 when there is no change in temperature, we can rearrange the specific heat capacity formula:
C=QnΔT
Substituting ΔT=0 leads to:
C=Qn(0)
This expression is undefined because division by zero is not possible.
Step 5: Conclusion
Thus, we conclude that we cannot define specific heat capacity at constant temperature because it leads to an undefined situation.
Final Answer
No, we cannot define specific heat capacity at constant temperature as it becomes undefined due to division by zero.
---
Specific heat capacity (C) is defined as the amount of heat (Q) required to change the temperature of a unit mass (n) of a substance by one degree Celsius (or one Kelvin). Mathematically, this is expressed as:
Q=nCΔT
where:
- Q = heat added or removed
- n = mass of the substance
- C = specific heat capacity
- ΔT = change in temperature
In a constant temperature process, the temperature does not change. Therefore, the change in temperature (ΔT) is equal to zero:
ΔT=0
Substituting ΔT=0 into the heat equation gives:
Q=nC(0)
This simplifies to:
Q=0
Since Q=0 when there is no change in temperature, we can rearrange the specific heat capacity formula:
C=QnΔT
Substituting ΔT=0 leads to:
C=Qn(0)
This expression is undefined because division by zero is not possible.
Thus, we conclude that we cannot define specific heat capacity at constant temperature because it leads to an undefined situation.
No, we cannot define specific heat capacity at constant temperature as it becomes undefined due to division by zero.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The specific heat capacity of water is :
A4200 J kg−1K−1B420 J g−1K−1C0.42 J g−1K−1D4.2 J kg−1K−1The ratio CpCv=γ for a gas. Its molecular weight is M. Its specific heat capacity at constant pressure is
ARγ−1BγRγ−1CγRM(γ−1)DγRMγ−1Which one of the following graphs represents variation of specific heat capacity of water with temperature?
A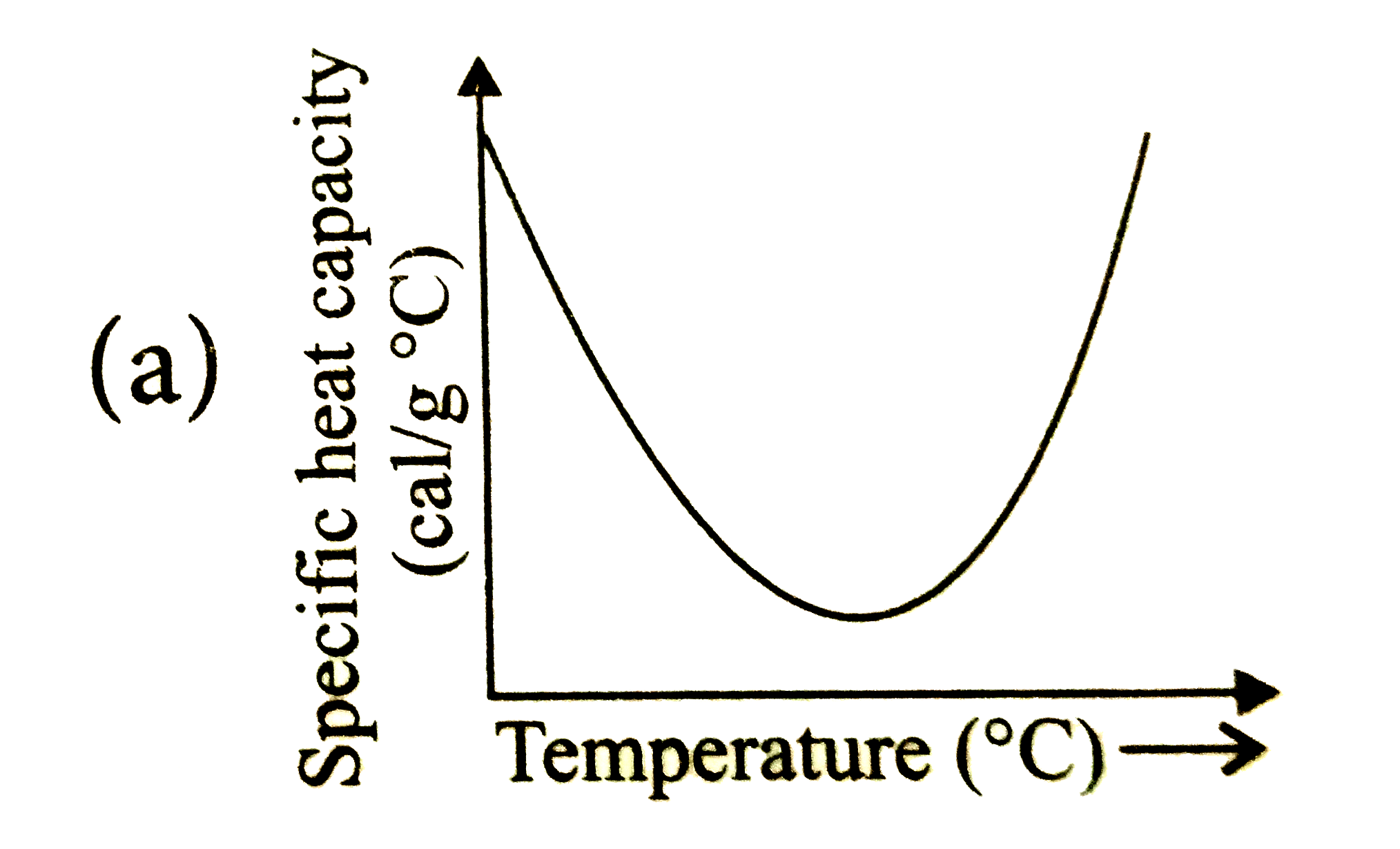 B
B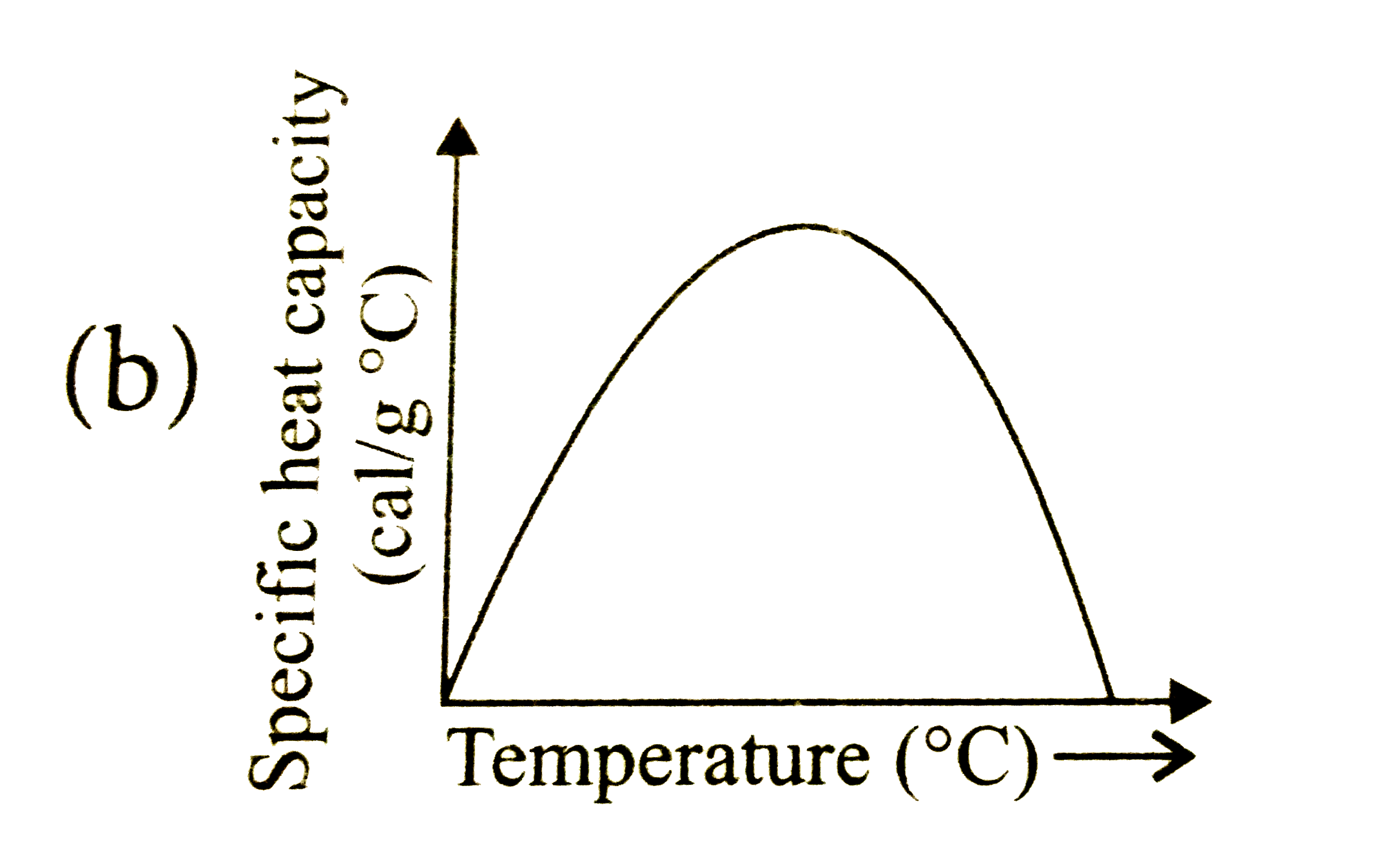 C
C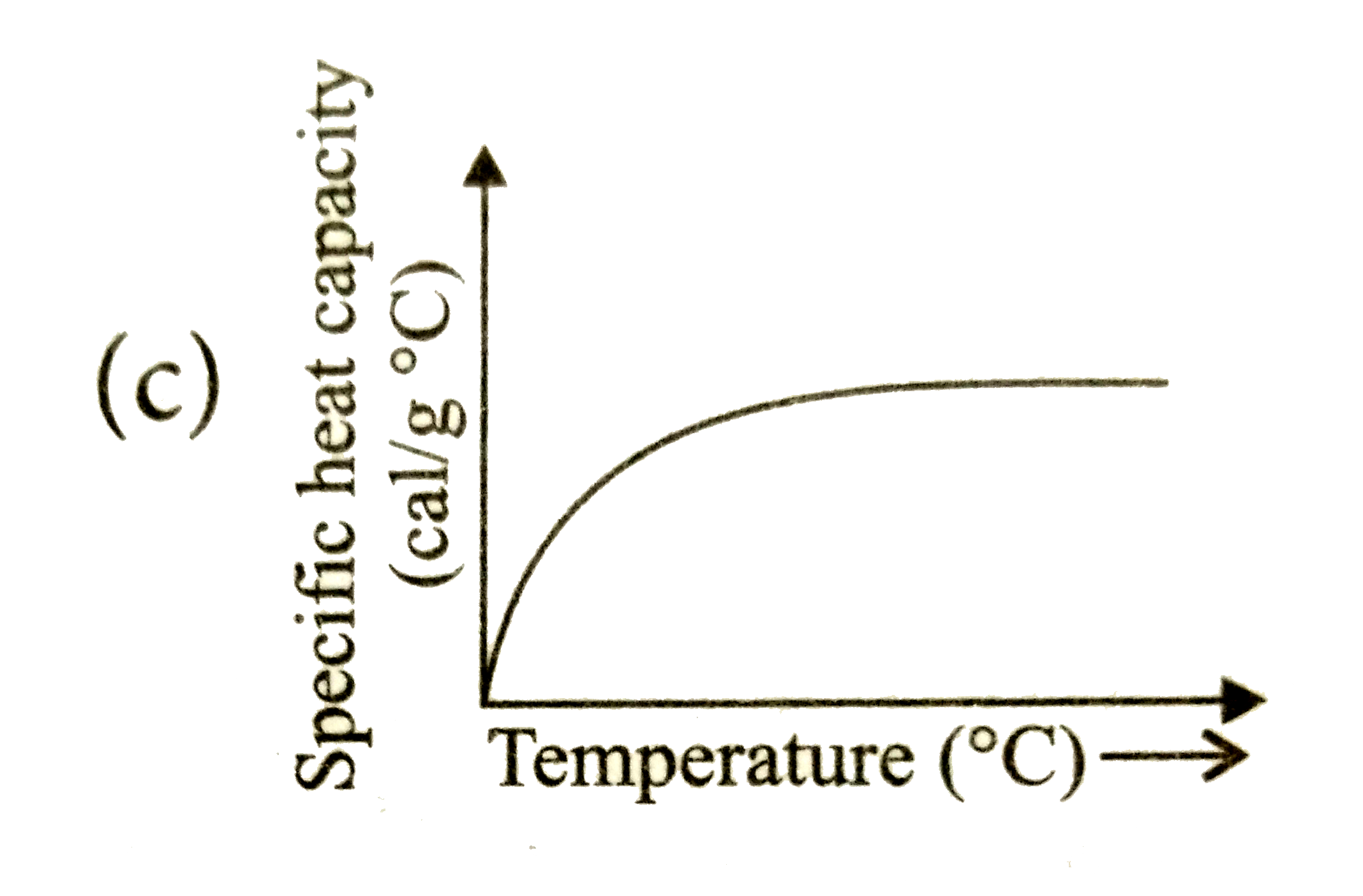 D
D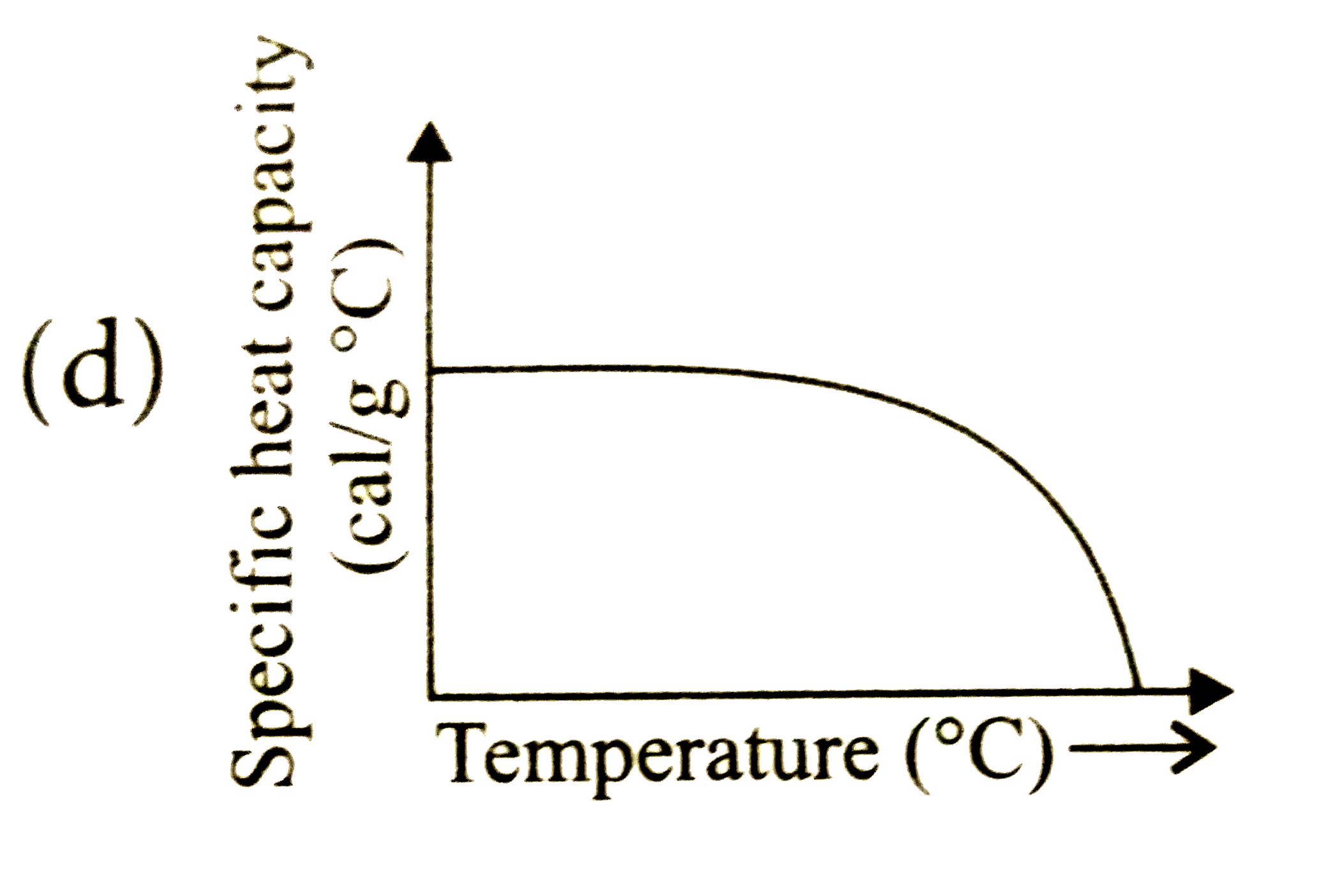
A mass m1 of a substance of specific heat capacity c1 at temperature t1 is mixed with a mass m2 of other substance of specific heat capacity c2 at a lower temperature t2. Deduce the expression for the temperature t of the mixture. State the assumption made, if any.
View SolutionIf the ratio of principal specific heat capacities of a certain gas is 1:4 and its density at STP is 0.09 kgm−3 calculate the values of specific heat capacity at constant pressure and constant volume. [Standard atmospheric pressure =1.01×105Nm−2
View SolutionThe difference between the two specific heat capacities (at constant pressure and volume) of a gas is 5000J kg−1 K−1 and the ratio of these specific heat capacities, i.e., CV and CP is 1.6. Then CV and CP
View SolutionDefine specific heat of body.
View SolutionWhich one of the following substances has highest specific heat capacity at room temperature and atmospheric pressure ? a) Water b) Ice c) Aluminum d) Mercury
View Solution
