A capacitor with no dielectric is connected to a battery at t=0.Consider a point A in the connecting wires and a point B in between the plates. What about the current at A and B ?
(a)There is no current through A.
(b)There is no current through B.
(c )There is a current through A as long as the charging is not complete.
(d)There is a current through B as long as the charging is not complete.
The correct Answer is:B, C
To solve the problem, we need to analyze the behavior of the current at two points: point A in the connecting wire and point B between the plates of the capacitor when the capacitor is connected to a battery at time t=0.
1. Understanding the Circuit Configuration:
- At t=0, a capacitor (with no dielectric) is connected to a battery. The positive terminal of the battery is connected to one plate of the capacitor, and the negative terminal is connected to the other plate.
2. Current at Point A (Connecting Wire):
- Point A is located in the connecting wire. When the switch is closed at t=0, the circuit is completed, allowing current to flow.
- Since the capacitor is initially uncharged, it behaves like a short circuit at this moment. Therefore, there will be a current flowing through the wire at point A.
3. Current at Point B (Between the Plates):
- Point B is situated between the plates of the capacitor. At t=0, the plates of the capacitor are not yet charged.
- The current in the capacitor is defined as the flow of charge. However, between the plates, there is no physical movement of charge carriers (like electrons) because the capacitor is not yet charged. The electric field starts to build up due to the separation of charges on the plates, but no current flows through the dielectric gap.
- Thus, at point B, the current is zero.
4. Conclusion:
- At point A (in the connecting wire), the current is flowing (non-zero).
- At point B (between the plates of the capacitor), the current is zero.
Summary:
- Current at point A: Non-zero (current is flowing)
- Current at point B: Zero (no current)
- At t=0, a capacitor (with no dielectric) is connected to a battery. The positive terminal of the battery is connected to one plate of the capacitor, and the negative terminal is connected to the other plate.
- Point A is located in the connecting wire. When the switch is closed at t=0, the circuit is completed, allowing current to flow.
- Since the capacitor is initially uncharged, it behaves like a short circuit at this moment. Therefore, there will be a current flowing through the wire at point A.
- Point B is situated between the plates of the capacitor. At t=0, the plates of the capacitor are not yet charged.
- The current in the capacitor is defined as the flow of charge. However, between the plates, there is no physical movement of charge carriers (like electrons) because the capacitor is not yet charged. The electric field starts to build up due to the separation of charges on the plates, but no current flows through the dielectric gap.
- Thus, at point B, the current is zero.
- At point A (in the connecting wire), the current is flowing (non-zero).
- At point B (between the plates of the capacitor), the current is zero.
- Current at point A: Non-zero (current is flowing)
- Current at point B: Zero (no current)
Topper's Solved these Questions
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Exercises
84 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - questions for short answer
17 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Objective 1
15 Videos
- DISPERSION AND SPECTRABOOK - HC VERMA ENGLISHCHAPTER - DISPERSION AND SPECTRAEXERCISE - Question for short Answer
6 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Short answer
6 Videos
Similar Questions
The plates of a parallel plate capacitor with no dielectirc are connected to a volatage source. Now a dielectric of dielectric constant K is inserted to fill the whose space between the plates with voltage source remainign connected to the capacitor-
View SolutionAn LR circuit with a battery connected at t =0. Which of the following quantities will be zero just after the connection?
View Solution
Knowledge Check
A parallel plate capacitor without any dielectric within its plates, has a capacitance C, and is connected to a battery of emf V. The battery is disconnected and the plates of the capacitor are pulled apart until the separation between the plates is doubled. What is the work done by the agent pulling the plates apart, in this process ?
A12CV2B32CV2C−32CV2DCV2The potential difference between points A and B is
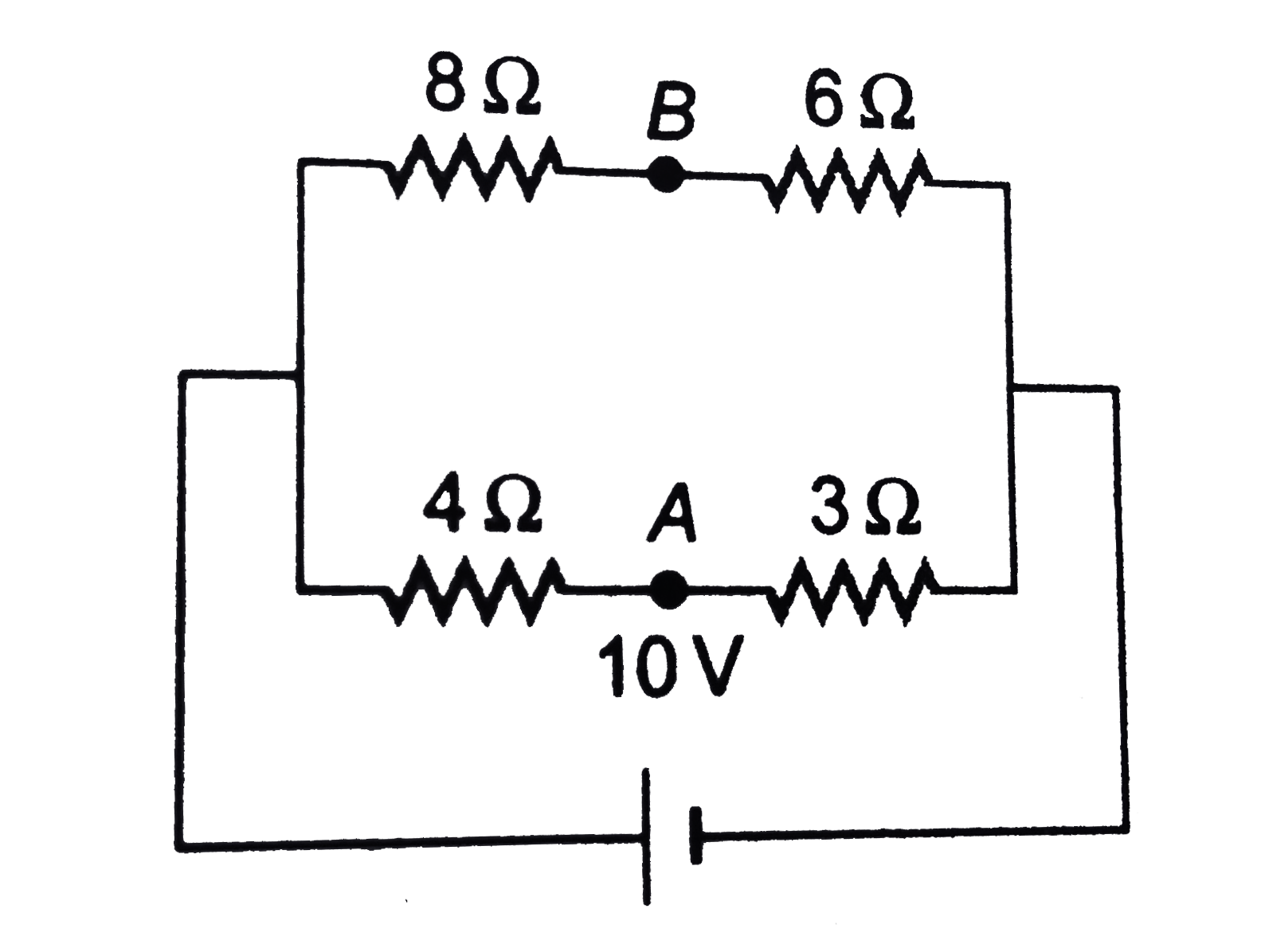 A2B5C11D18
A2B5C11D18
A capacitor is connected to a battery. The force of attraction between the plates when the separation between them is halved.
View SolutionA parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
View SolutionA parallel plate capacitor (without dielectric) is charged by a battery and kept connected to the battery. A dielectric salb of dielectric constant 'k' is inserted between the plates fully occupying the space between the plates. The energy density of electric field between the plates will:
View SolutionA parallel plate capacitor of capacitance 90 pF is connected to a battery of emf 20 V. If a dielectric material of dielectric constant K=53 is inserted between the plates, the magnitude of the induced charge will be :
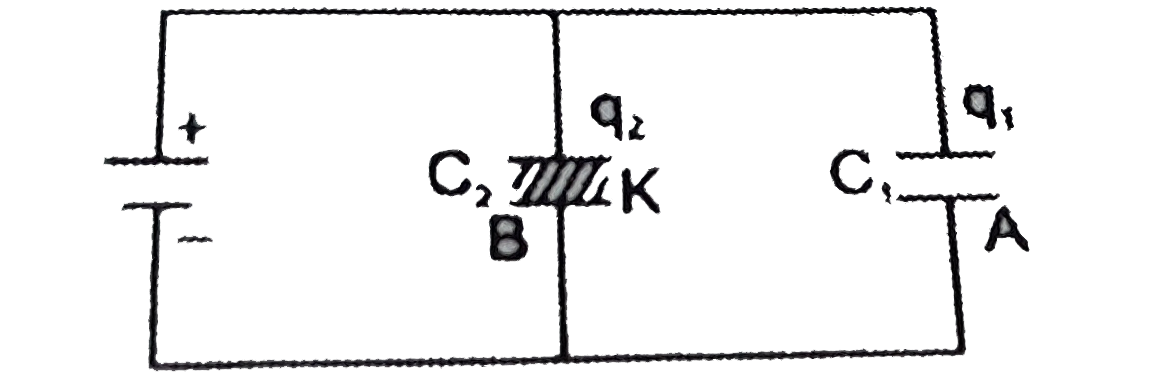
View SolutionIn the adjoining diagram two geometrically identical capacitors A and B are connected to a battery. Air is filled between the plates of C1 and a dielectric is filled between the plates of C2, then -
View SolutionA parallel plate capacitor is connected to a battery of emf V volts. Now a slab of dielectric constant k=2 is inserted between the plates of capacitor without disconnecting the battery. The electric field between the plates of capacitor after inserting the slab is E=PV2d. Find the value of P.
View Solution
