Show that AB+¯¯¯¯¯¯AB is always 1.
To show that AB+¯¯¯¯¯¯¯¯AB=1, we can follow these steps:
Step 1: Define the Variables
Let x=AB. Then, we can express the complement of x as ¯¯¯x=¯¯¯¯¯¯¯¯AB.
Step 2: Understand the Values of x
The value of x (which is AB) can either be 0 or 1:
- If AB=0, then A and B are not both true.
- If AB=1, then both A and B are true.
Step 3: Determine the Value of ¯¯¯x
The complement ¯¯¯x will have the following values based on x:
- If x=0 (i.e., AB=0), then ¯¯¯x=1.
- If x=1 (i.e., AB=1), then ¯¯¯x=0.
Step 4: Add x and ¯¯¯x
Now, we can add x and ¯¯¯x:
- Case 1: If x=0, then x+¯¯¯x=0+1=1.
- Case 2: If x=1, then x+¯¯¯x=1+0=1.
Step 5: Conclusion
In both cases, we find that x+¯¯¯x=1. Therefore, we conclude that:
AB+¯¯¯¯¯¯¯¯AB=1
Let x=AB. Then, we can express the complement of x as ¯¯¯x=¯¯¯¯¯¯¯¯AB.
The value of x (which is AB) can either be 0 or 1:
- If AB=0, then A and B are not both true.
- If AB=1, then both A and B are true.
The complement ¯¯¯x will have the following values based on x:
- If x=0 (i.e., AB=0), then ¯¯¯x=1.
- If x=1 (i.e., AB=1), then ¯¯¯x=0.
Now, we can add x and ¯¯¯x:
- Case 1: If x=0, then x+¯¯¯x=0+1=1.
- Case 2: If x=1, then x+¯¯¯x=1+0=1.
In both cases, we find that x+¯¯¯x=1. Therefore, we conclude that:
AB+¯¯¯¯¯¯¯¯AB=1
Topper's Solved these Questions
- SEMICONDUCTOR AND SEMICONDUCTOR DEVICESBook:HC VERMA ENGLISHChapter:SEMICONDUCTOR AND SEMICONDUCTOR DEVICESExercise:Question for short answer
Explore 11 Videos
- SEMICONDUCTOR AND SEMICONDUCTOR DEVICESBook:HC VERMA ENGLISHChapter:SEMICONDUCTOR AND SEMICONDUCTOR DEVICESExercise:objective II
Explore 8 Videos
- PHOTOMETRYBook:HC VERMA ENGLISHChapter:PHOTOMETRYExercise:All Questions
Explore 38 Videos
- SPEED OF LIGHTBook:HC VERMA ENGLISHChapter:SPEED OF LIGHTExercise:Question for short Answer
Explore 5 Videos
Similar Questions
Show that if A and B are symmetric and commute ,then
AB−1
View SolutionConsider △ABC and △A1B1C1 in such a way that ¯¯¯¯¯¯AB=¯¯¯¯¯¯¯¯¯A1B1 and M,N,M1,N1 be the midpoints of AB,BC,A1B1andB1C1 respectively, then
View SolutionShow that : logam÷logabm=1+logab
View SolutionShow that , if A and B are square matrices such that AB=BA, then (A+B)2=A2+2AB+B2.
View Solution1a,1band1c are in A.P. Show that bc, ca, and ab are also in A.P.
View SolutionIn a quadrilateral ABCD, it is given that AB∣∣CD and the diagonals ACandBD are perpendicular to each other. Show that AD.BC≥AB.CD.
View SolutionFor two non-singular matrices A&B, show that adj (AB)=adj(AB)=(adjB)(adjA)
View Solution(i)ifA=[1−431]andb=[105−243],then show that :
(AB)'=B'A'
(ii)ifA=[2301]andB=[3421],then prove that :
(AB)'=B'A'
View SolutionIf →a and →b are two unit vectors and θ is the angle between them, then the unit vector along the angular bisector of →a and →b will be given by
View SolutionIn the adjoining figure
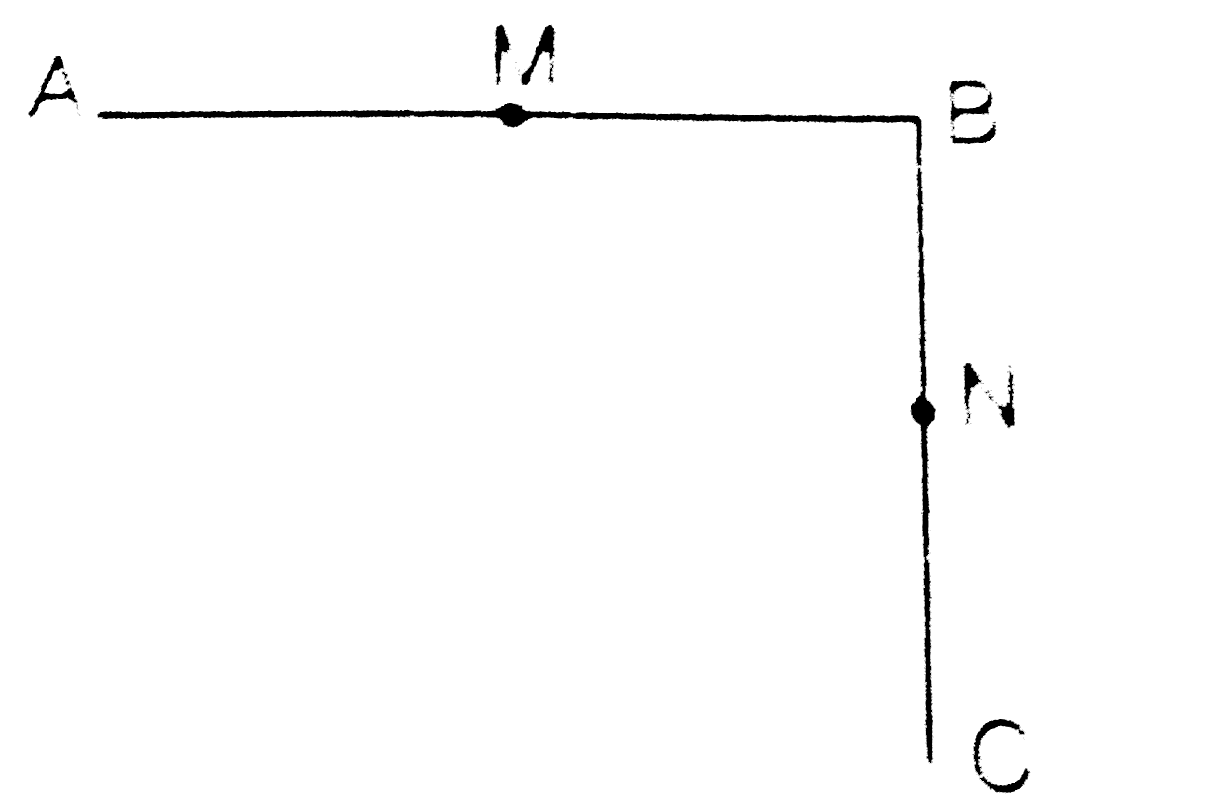
(i) AB=BC, M is the mid -point of AB and N is the mid- point of BC. Show that AM=NC.
(ii) BM=BN,M is the mid- point of AB and N is the mid - point of BC. Show that AB=BC.
View Solution