Consider the beta decay
^198Au→198Hg∗+Bη−1+→v.
where ^198Hg∗ represents a mercury nucleus in an excited state at energy 1.088MeV above the ground state. What can be the maximum kinetic energy of the electron emitted? The atomic mass of ^198Au is 197.968233u and that of ^198Hg is 197.966760u.
To find the maximum kinetic energy of the emitted electron during the beta decay of gold to mercury, we can follow these steps:
Step 1: Understand the Beta Decay Process
The beta decay process can be represented as:
198Au→198Hg∗+β−+ν
where 198Hg∗ is the excited state of mercury, β− is the emitted electron, and ν is the anti-neutrino.
Step 2: Identify the Masses
We are given:
- Atomic mass of 198Au=197.968233u
- Atomic mass of 198Hg=197.966760u
Step 3: Calculate the Q-value
The Q-value for the decay is given by the difference in mass-energy between the initial and final states. Since the mercury is in an excited state, we need to account for the excitation energy of 1.088MeV.
The Q-value can be calculated as:
Q=(mAu−mHg)c2−Eexcitation
where Eexcitation=1.088MeV.
Step 4: Convert Mass Difference to Energy
First, calculate the mass difference:
mAu−mHg=197.968233u−197.966760u=0.001473u
Now, convert this mass difference to energy using the conversion factor:
1u≈931MeV/c2
Thus,
(mAu−mHg)c2=0.001473u×931MeV/u
Calculating this gives:
(mAu−mHg)c2≈1.372MeV
Step 5: Calculate the Maximum Kinetic Energy
Now, substitute the values into the Q-value equation:
Q=1.372MeV−1.088MeV=0.284MeV
Step 6: Conclusion
The maximum kinetic energy of the emitted electron is approximately:
KEmax≈0.284MeV
The beta decay process can be represented as:
198Au→198Hg∗+β−+ν
where 198Hg∗ is the excited state of mercury, β− is the emitted electron, and ν is the anti-neutrino.
We are given:
- Atomic mass of 198Au=197.968233u
- Atomic mass of 198Hg=197.966760u
The Q-value for the decay is given by the difference in mass-energy between the initial and final states. Since the mercury is in an excited state, we need to account for the excitation energy of 1.088MeV.
Q=(mAu−mHg)c2−Eexcitation
where Eexcitation=1.088MeV.
First, calculate the mass difference:
mAu−mHg=197.968233u−197.966760u=0.001473u
1u≈931MeV/c2
Thus,
(mAu−mHg)c2=0.001473u×931MeV/u
Calculating this gives:
(mAu−mHg)c2≈1.372MeV
Now, substitute the values into the Q-value equation:
Q=1.372MeV−1.088MeV=0.284MeV
The maximum kinetic energy of the emitted electron is approximately:
KEmax≈0.284MeV
Topper's Solved these Questions
- THE NUCLEOUSBOOK - HC VERMA ENGLISHCHAPTER - THE NUCLEOUSEXERCISE - Objective 1
19 Videos
- THE NUCLEOUSBOOK - HC VERMA ENGLISHCHAPTER - THE NUCLEOUSEXERCISE - Objective 2
10 Videos
- THE NUCLEOUSBOOK - HC VERMA ENGLISHCHAPTER - THE NUCLEOUSEXERCISE - Short answer
12 Videos
- SPEED OF LIGHTBOOK - HC VERMA ENGLISHCHAPTER - SPEED OF LIGHTEXERCISE - Question for short Answer
5 Videos
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMA ENGLISHCHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Short answer
2 Videos
Similar Questions
Find the maximum energy that a beta particle can have in the following decay
^176Lu→176Hf+e+→v.
Alomic mass of ^176Lu is 175.942694u and that of ^176Hf is 175.941420u.
View SolutionDuring β-decay of stationary nucleus , an electron is observed with a kinetic energy 1.0 MeV . From this ,what can be concluded about the Q-value of the decay ?
View SolutionObtain the maximum kinetic energy of β-particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. 14.6. You are given that m(.198Au)=197.968233u,m(.198Hg)=197.966760u
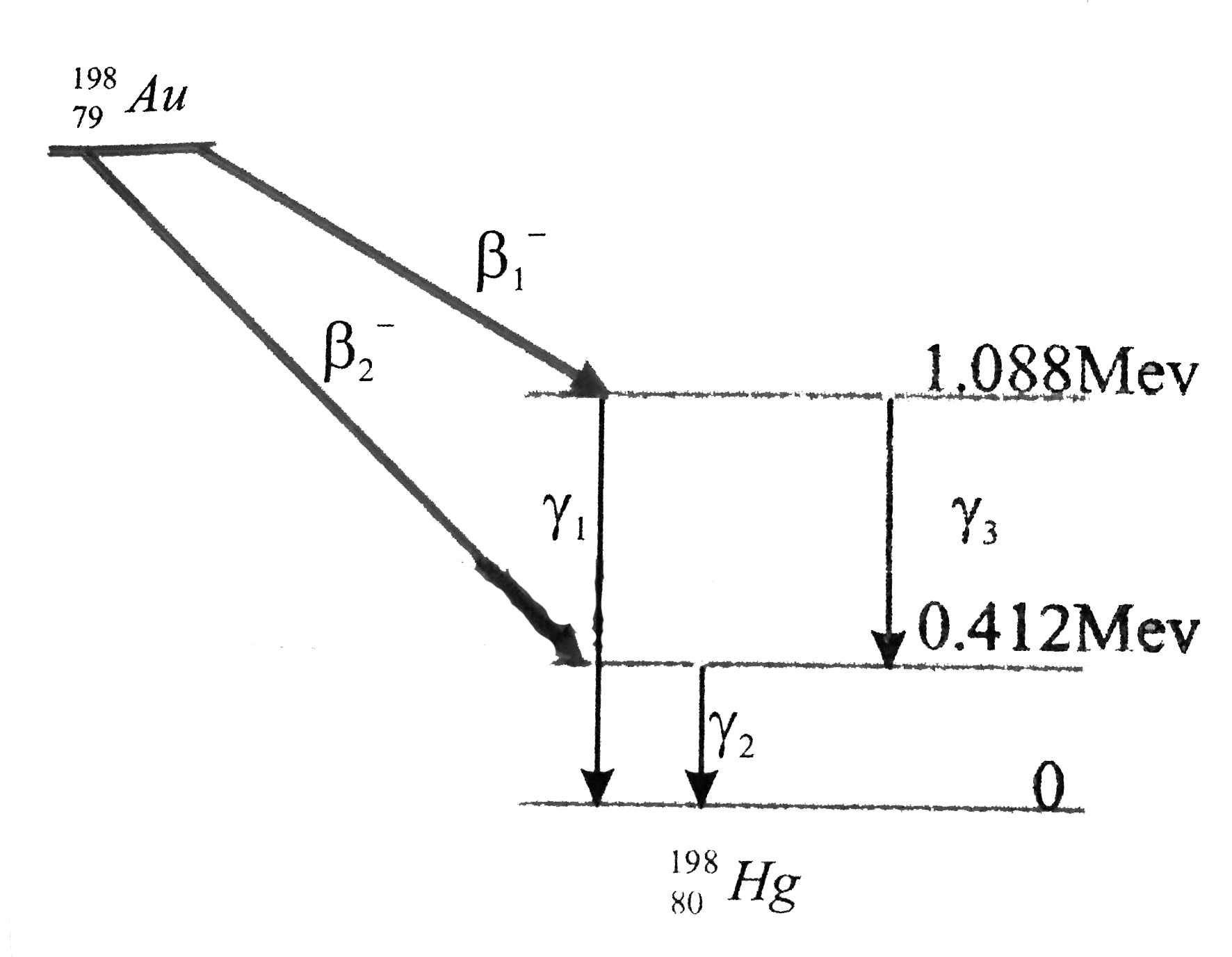
View SolutionThe isotope (5)12B having a mass 12.014uundergoes beta - decay to _(6)12C126Chas an excited state of the nucleus (_(6)12C∗at4.041MeV above its ground state if _(5)12E decay to _(6)12C∗ , the maximum kinetic energy of the β - particle in unit of MeV is (1u=931.5MeV/c2 where c is the speed of light in vaccuum) .
View SolutionGold .19879Au undergoes β− decay to an excited state of .19880Hg. If the excited state decays by emission of a γ-photon with energy 0.412MeV, the maximum kinetic energy of the electron emitted in the decay is (This maximum occurs when the antineutrino has negligble energy. The recoil enregy of the .19880Hg nucleus can be ignored. The masses of the neutral atoms in their ground states are 197.968255u for .19879Hg).
View SolutionNeon-23 decays in the following way,
.2310Ne→2311Na+0−1e+¯v
Find the minimum and maximum kinetic energy that the beta particle(.0−1e)can have. The atomic masses of .23Ne and .23Na are 22.9945u and 22.9898u, respectively.
View SolutionThe nucleus .23Ne deacays by β-emission into the nucleus .23Na. Write down the β-decay equation and determine the maximum kinetic energy of the electrons emitted. Given,(m(.2310Ne)=22.994466amu and m(.2311Na=22.989770amu. Ignore the mass of antineuttino (¯v).
View SolutionThe nucleus .23Ne deacays by β-emission into the nucleus .23Na. Write down the β-decay equation and determine the maximum kinetic energy of the electrons emitted. Given,(m(.2311Ne)=22.994466amu and m(.2311Na=22.989770amu. Ignore the mass of antineuttino (¯v).
View SolutionNeon-23 decays in the following way,
_1023Ne→2311Na+0−1e+¯v
Find the minimum and maximum kinetic energy that the beta particle(_(−1)0e)can have. The atomic masses of ^23Ne and ^23Na are 22.9945u and 22.9898u, respectively.
View SolutionConsider a nuclear reaction A+B→C. A nucleus A moving with kinetic energy of 5MeV collides with a nucleus B moving with kinetic energy of 3MeV and forms a nucleus C in exicted state. Find the kinetic energy of nucleus C just after its fromation if it is formed in a state with excitation energy 10MeV. Take masses of nuclei of A,B and C as 25.0,10.0,34.995aμ, respectively.
(1amu=930MeV/c2).
View Solution