For the homogenous gaseous reaction A→3B, if pressure after time t was Pt and after completion of reaction, pressure was P∞ then select correct relation
k=1tin(P∞3(P∞−Pt))
k=1tin(2P∞(P∞−PT))
k=1tin(3P∞2P∞−Pt)
k=1tin(P∞3(P∞−PT))
The correct Answer is:D
To solve the problem regarding the homogeneous gaseous reaction A→3B, we need to establish the relationships between the pressures at different stages of the reaction. Here’s a step-by-step breakdown of the solution:
Step 1: Define Initial Conditions
- Let the initial pressure of gas A be P0.
- At the start of the reaction (when t=0), the pressure of B is 0.
Step 2: Establish Pressure Changes Over Time
- As the reaction progresses, let P be the change in pressure due to the conversion of A to B.
- At time t, the pressure of A will be P0−P.
- The pressure of B will be 3P (since 1 mole of A produces 3 moles of B).
Step 3: Total Pressure at Time t
- The total pressure at time t can be expressed as:
Pt=(P0−P)+3P=P0+2P
Step 4: Pressure at Completion of Reaction
- When the reaction is complete (as t→∞), all of A is converted to B.
- Thus, the pressure at completion P∞ will be:
P∞=3P0
Step 5: Relate P and P∞
- From the expression for Pt, we can rearrange to find P:
P=Pt−P02
Step 6: Substitute P in Terms of P∞
- Since P∞=3P0, we can express P0 as:
P0=P∞3
Step 7: Substitute P0 into the Equation for P
- Substitute P0 into the equation for P:
P=Pt−P∞32
Step 8: Substitute P back into the expression for Pt
- Now we can substitute P back into the equation for Pt:
Pt=P0+2⎛⎜⎝Pt−P∞32⎞⎟⎠
- This simplifies to:
Pt=P0+Pt−P∞3
- Rearranging gives us:
Pt=P0+Pt−P∞3
Step 9: Final Relation
- After simplification, we find the relationship:
Pt=2P∞3
Summary of the Solution
The correct relation derived from the problem is:
Pt=2P∞3
- Let the initial pressure of gas A be P0.
- At the start of the reaction (when t=0), the pressure of B is 0.
- As the reaction progresses, let P be the change in pressure due to the conversion of A to B.
- At time t, the pressure of A will be P0−P.
- The pressure of B will be 3P (since 1 mole of A produces 3 moles of B).
- The total pressure at time t can be expressed as:
Pt=(P0−P)+3P=P0+2P
- When the reaction is complete (as t→∞), all of A is converted to B.
- Thus, the pressure at completion P∞ will be:
P∞=3P0
- From the expression for Pt, we can rearrange to find P:
P=Pt−P02
- Since P∞=3P0, we can express P0 as:
P0=P∞3
- Substitute P0 into the equation for P:
P=Pt−P∞32
- Now we can substitute P back into the equation for Pt:
Pt=P0+2⎛⎜⎝Pt−P∞32⎞⎟⎠
- This simplifies to:
Pt=P0+Pt−P∞3
- Rearranging gives us:
Pt=P0+Pt−P∞3
- After simplification, we find the relationship:
Pt=2P∞3
The correct relation derived from the problem is:
Pt=2P∞3
Similar Questions
For a first order homogeneous gaseous reaction
A→2B+C
if the total pressure after time t was P1 and after long time (t→∞) was P∞ then K in terms of Pt1 P∞ t is
View SolutionFor a first order homogenous gaseous reaction, A→2B+C
then initial pressure was Pi while total pressure after time 't' was Pt. The right expression for the rate constants k in terms of Pi,Pt and t is :
View SolutionFor a homogeneous gaseous reaction A→B+C+D , the initial pressure was P0 white pressure after time 't' was P. if (P>P0) The expression for the constant K is
View SolutionIn a gaseous reaction, an increase in the pressure of the reactant gases increases the rate of reaction.T or F
View SolutionBenene diazonium chloride in aqueous solution decomposes as : C6H5−N=N+Cl−aq+H2Oaq→C6H5OHaq+N2(g)+HClaq The reaction follows first order kinetics. If Pt is the pressure of N2 at time t and Pf that after completion of the reaction, at constant volume and temperature, then which of the following graphs conforms to the kinetics of the reaction ?
View SolutionIn the given (V-T) diagram, what is the relation between pressure P1andP2 ?
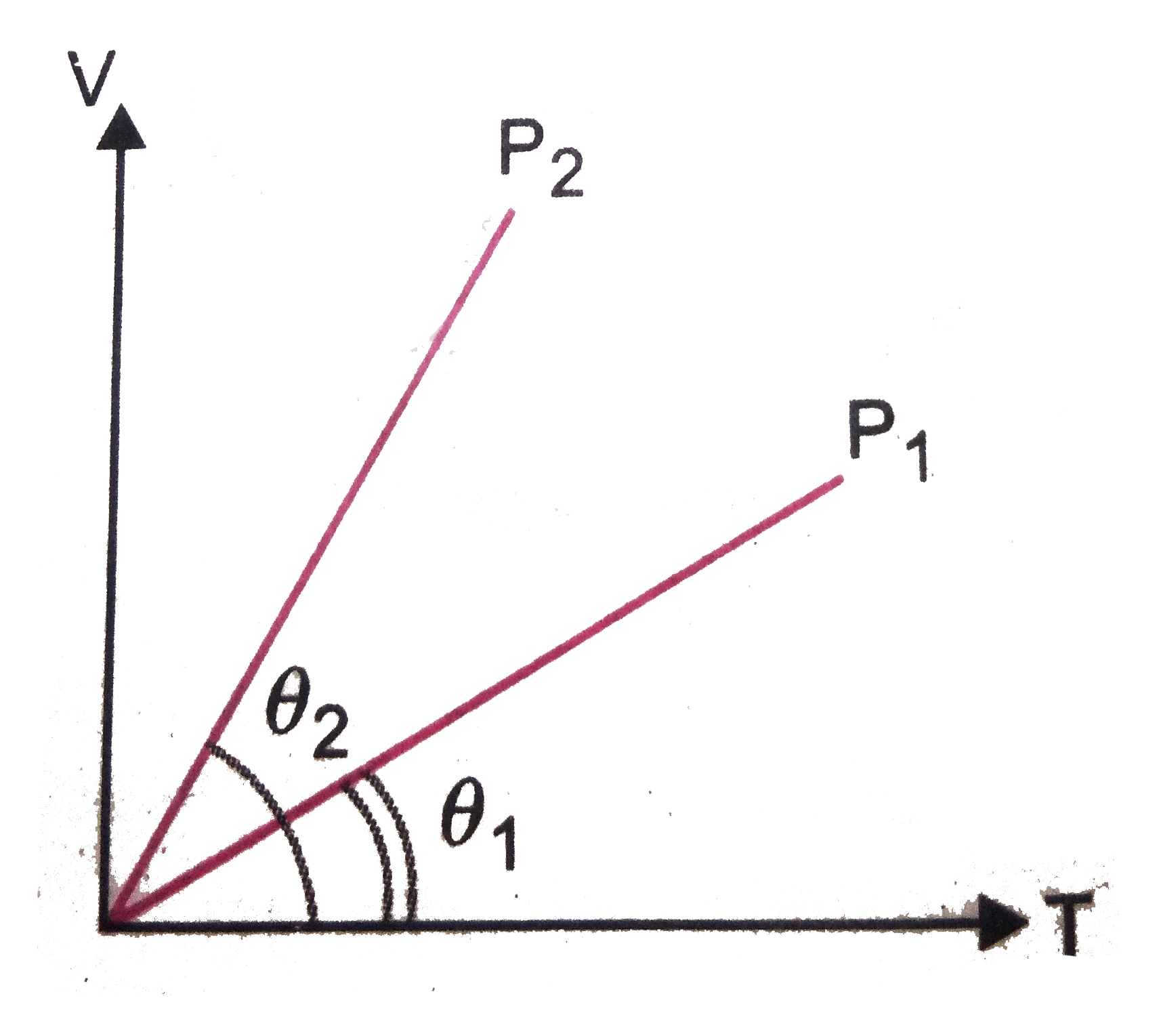
View SolutionIn the given (V-T) diagram, what is the relation between pressure P1andP2 ?

View SolutionIn the given (V-T) diagram, what is the relation between pressure P1andP2 ?

View SolutionFor a first order gas phase reaction :
A(g)→2B(g)+C(g)
P0 be initial pressure of A and Pt the total pressure at time 't'. Integrated rate equation is :
View SolutionThere is no relation between the stoichiometry of the reaction and order of the reaction, order is always experimently calculated. Monitoring of the progress of the reaction can be done by pressure measurement (P0,Pt & P∞ are total pressure of reaction mixture at time, t=0, t=t & t=∞) .All following reactions in Column-1 have half life 5 minutes.

Select incorrect combination for Ist order reaction :
View Solution
