A rigid and insulated tank of 3m3 volume is divided into two compartments. One compartment of volume of 2m3 contains an ideal gas at 0.8314 Mpa and 400 K while the second compartment of volume of 1m3 contains the same gas at 8.314 Mpa and 500 K. If the partition between the two compartments is rptured, the final temperature of the gas is :
420 K
450 K
480 K
None of these
The correct Answer is:C
To solve the problem step by step, we will follow these calculations:
Step 1: Write down the given data
- For compartment 1:
- Volume (V1) = 2 m³
- Pressure (P1) = 0.8314 MPa = 0.8314 × 10^6 Pa
- Temperature (T1) = 400 K
- For compartment 2:
- Volume (V2) = 1 m³
- Pressure (P2) = 8.314 MPa = 8.314 × 10^6 Pa
- Temperature (T2) = 500 K
Step 2: Calculate the number of moles in each compartment using the ideal gas equation
The ideal gas equation is given by:
PV=nRT
Where:
- P = pressure
- V = volume
- n = number of moles
- R = gas constant = 8.314 J/(mol·K)
- T = temperature
For compartment 1:
n1=P1V1RT1
Substituting the values:
n1=(0.8314×106Pa)×(2m3)(8.314J/(mol⋅K))×(400K)
Calculating:
n1=16626803325.6≈500moles
For compartment 2:
n2=P2V2RT2
Substituting the values:
n2=(8.314×106Pa)×(1m3)(8.314J/(mol⋅K))×(500K)
Calculating:
n2=83140004157≈2000moles
Step 3: Apply the first law of thermodynamics
Since the tank is insulated and rigid, the heat transfer Q=0 and work done W=0. Therefore, the change in internal energy ΔU=0.
The change in internal energy can be expressed as:
ΔU=n1Cv(Tf−T1)+n2Cv(Tf−T2)
Since Cv is the same for both compartments, we can factor it out:
0=Cv(n1(Tf−T1)+n2(Tf−T2))
Step 4: Rearranging the equation
Rearranging gives:
n1(Tf−T1)+n2(Tf−T2)=0
Expanding this:
n1Tf−n1T1+n2Tf−n2T2=0
(n1+n2)Tf=n1T1+n2T2
Step 5: Solve for the final temperature Tf
Substituting the values:
Tf=n1T1+n2T2n1+n2
Tf=(500×400)+(2000×500)500+2000
Tf=200000+10000002500
Tf=12000002500
Tf=480K
Final Answer:
The final temperature of the gas after the partition is ruptured is 480 K.
---
- For compartment 1:
- Volume (V1) = 2 m³
- Pressure (P1) = 0.8314 MPa = 0.8314 × 10^6 Pa
- Temperature (T1) = 400 K
- Volume (V2) = 1 m³
- Pressure (P2) = 8.314 MPa = 8.314 × 10^6 Pa
- Temperature (T2) = 500 K
The ideal gas equation is given by:
PV=nRT
Where:
- P = pressure
- V = volume
- n = number of moles
- R = gas constant = 8.314 J/(mol·K)
- T = temperature
n1=P1V1RT1
Substituting the values:
n1=(0.8314×106Pa)×(2m3)(8.314J/(mol⋅K))×(400K)
n1=16626803325.6≈500moles
n2=P2V2RT2
Substituting the values:
n2=(8.314×106Pa)×(1m3)(8.314J/(mol⋅K))×(500K)
n2=83140004157≈2000moles
Since the tank is insulated and rigid, the heat transfer Q=0 and work done W=0. Therefore, the change in internal energy ΔU=0.
ΔU=n1Cv(Tf−T1)+n2Cv(Tf−T2)
Since Cv is the same for both compartments, we can factor it out:
0=Cv(n1(Tf−T1)+n2(Tf−T2))
Rearranging gives:
n1(Tf−T1)+n2(Tf−T2)=0
n1Tf−n1T1+n2Tf−n2T2=0
(n1+n2)Tf=n1T1+n2T2
Substituting the values:
Tf=n1T1+n2T2n1+n2
Tf=(500×400)+(2000×500)500+2000
Tf=200000+10000002500
Tf=12000002500
Tf=480K
The final temperature of the gas after the partition is ruptured is 480 K.
Topper's Solved these Questions
Similar Questions
A sound wave passing volume 1m3 is divided into two equal compartments by a partition. One of these compartments contains an ideal gas at 300K. The other compartment is vacuum. The whole system is thermally isolated from its surroundings. The partition is removed and the gas expands to occupy the whole volume of the container. Its temperature now would be
View SolutionAn insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
View Solution
Knowledge Check
Two moles of an ideal monoatomic gas occupy a volume 2V at temperature 300K, it expands to a volume 4V adiabatically, then the final temperature of gas is
Aa.179 KBb.189 KCc.199 KDd.219 K
An insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
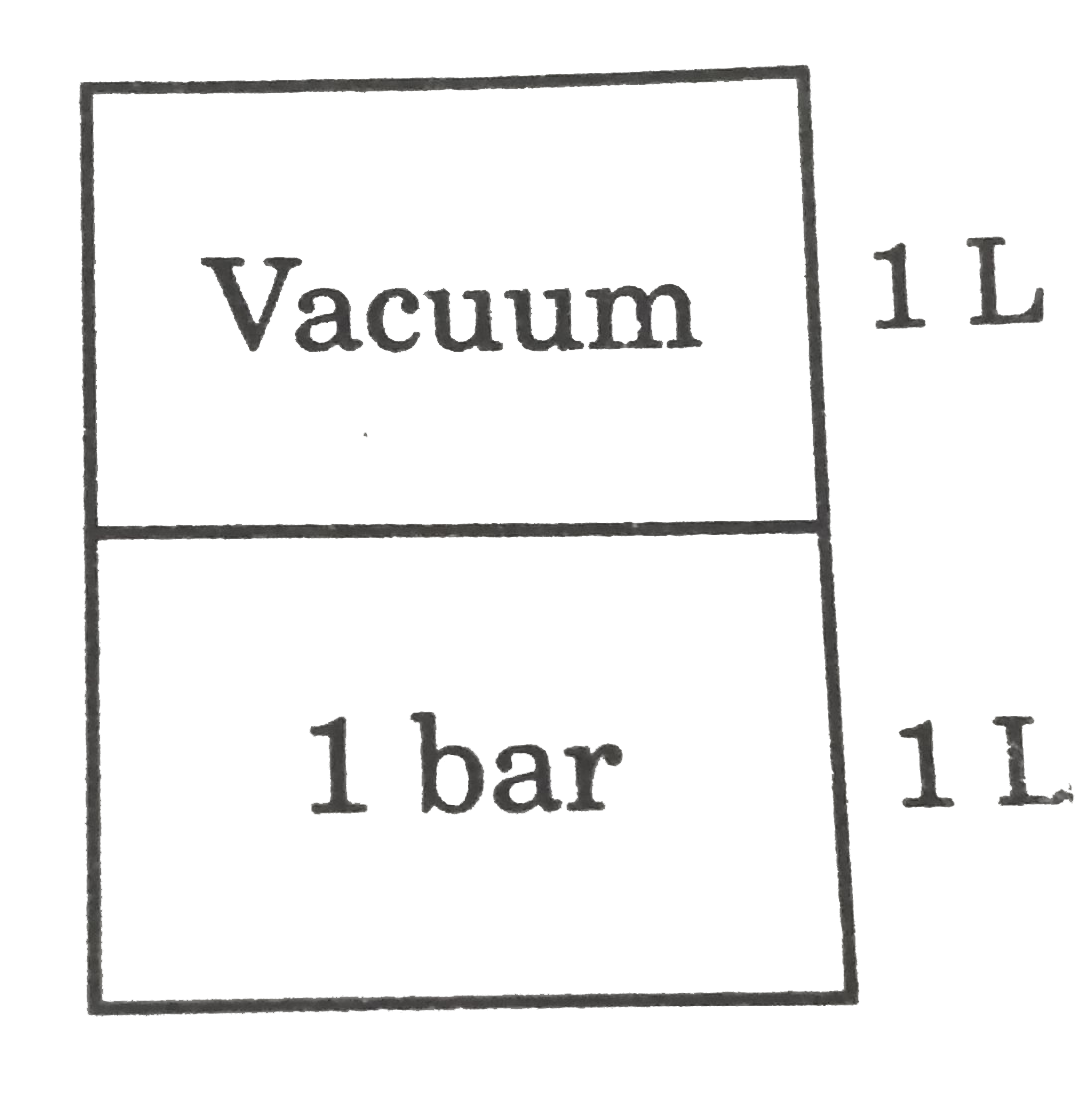
View SolutionA container of volume 2L is seperated into equal compartments. In one compartment, one mole of an ideal monoatomic gas is filled at 1 bar pressure and the other compartment is completely evacuted. A pinhole is made in the seperator so gas expands to occupy full 2 L and heat is supplied to gas so that finally pressure of gas equals 1 bar. Then :
View SolutionIn the figure, a container is shown to have a movable (without friction) piston on top. The container and the piston are all made of perfectly insulated material allowing no heat transfer between outside and inside the container. The container is divided into two compartments by a rigid partition made of a thermally conducting material that allows slow transfer of heat. The lower compartment of the container is filled with 2 moles of an ideal monoatomic gas at 700K and the upper compartment is filled with 2 moles of an ideal diatomic gas at 400K. The heat capacities per mole of an ideal monoatomic gas are CV=32R,CP=52R, and those for an ideal diatomic gas are CV=52R,CP=72R,

Consider the partition to be rigidly fixed so that it does not move. When equilibrium is achieved, the final temperature of the gasses will be
View SolutionIn the figure, a container is shown to have a movable (without friction) piston on top. The container and the piston are all made of perfectly insulated material allowing no heat transfer between outside and inside the container. The container is divided into two compartments by a rigid partition made of a thermally conducting material that allows slow transfer of heat. The lower compartment of the container is filled with 2 moles of an ideal monoatomic gas at 700K and the upper compartment is filled with 2 moles of an ideal diatomic gas at 400K. The heat capacities per mole of an ideal monoatomic gas are CV=32R,CP=52R, and those for an ideal diatomic gas are CV=52R,CP=72R,

Consider the partition to be rigidly fixed so that it does not move. When equilibrium is achieved, the final temperature of the gasses will be
View SolutionA container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionA container of volume 1m3 is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be .......
View SolutionContainer A holds an ideal gas at a pressure 1×105 Pa and at 300 K. Container B whose volume is 4 times the volume of A has the same ideal gas at 400 K and at a pressure of 5×105
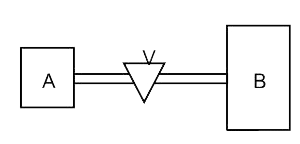
Before the valve V is opened, the ratio of number of moles of gas in A and B is :
View Solution
