Let a parabola be y=12−x2. Find the maximum area of rectangle whose base lie on x-axis and two points lie on parabola. (A) 8 (B) 4 (C) 32 (D) 34
To find the maximum area of a rectangle whose base lies on the x-axis and whose upper corners lie on the parabola defined by the equation y=12−x2, we can follow these steps:
Step 1: Define the Rectangle
Let the coordinates of the points where the rectangle touches the parabola be (−α,12−α2) and (α,12−α2). The base of the rectangle lies on the x-axis between these two points.
Step 2: Calculate the Area of the Rectangle
The width of the rectangle is the distance between the two points on the x-axis, which is 2α. The height of the rectangle is the y-coordinate of the points on the parabola, which is 12−α2. Therefore, the area A of the rectangle can be expressed as:
A=width×height=2α(12−α2)
This simplifies to:
A=24α−2α3
Step 3: Differentiate the Area Function
To find the maximum area, we need to differentiate A with respect to α and set the derivative equal to zero:
dAdα=24−6α2
Setting the derivative equal to zero gives:
24−6α2=0
Step 4: Solve for α
Rearranging the equation:
6α2=24⟹α2=4⟹α=2(since α must be positive)
Step 5: Calculate the Maximum Area
Now substitute α=2 back into the area formula:
A=2(2)(12−22)=4(12−4)=4×8=32
Conclusion
The maximum area of the rectangle is 32.
Let the coordinates of the points where the rectangle touches the parabola be (−α,12−α2) and (α,12−α2). The base of the rectangle lies on the x-axis between these two points.
The width of the rectangle is the distance between the two points on the x-axis, which is 2α. The height of the rectangle is the y-coordinate of the points on the parabola, which is 12−α2. Therefore, the area A of the rectangle can be expressed as:
A=width×height=2α(12−α2)
This simplifies to:
A=24α−2α3
To find the maximum area, we need to differentiate A with respect to α and set the derivative equal to zero:
dAdα=24−6α2
Setting the derivative equal to zero gives:
24−6α2=0
Rearranging the equation:
6α2=24⟹α2=4⟹α=2(since α must be positive)
Now substitute α=2 back into the area formula:
A=2(2)(12−22)=4(12−4)=4×8=32
The maximum area of the rectangle is 32.
Topper's Solved these Questions
Similar Questions
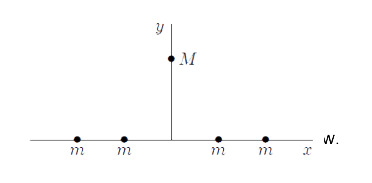
Four particles, each with mass m are arranged symmetrically about the origin on the x axis. A fifth particle, with mass M, is on the y axis. The direction of the gravitational force on M is:
View SolutionFind the area of the largest rectangle with lower base on the x-axis and upper vertices on the curve y=12−x2.
View SolutionThe focal distance of a point on a parabola y2=8x is 8. Find it .
View SolutionFind the area lying above the x-axis and under the parabola y=4x−x2
View SolutionFind the area of the region bounded by the parabola y2=4ax, its axis and two ordinates x=a and x=2a.
View SolutionFind the area of the region bounded by the parabola y2=4ax, its axis and two ordinates x=4 and x=9
View SolutionFind the area enclosed by the parabola 4y=3x2 and the line 2y=3x+12.
View SolutionFind the equation of the parabola whose focus is (−3,2) and the directrix is x+y=4.
View SolutionFind the angle between two tangents drawn from the point ( 1,4) to the parabola y2=12x
View SolutionProve that points of the curve y2=4a{x+asin(xa)} at which tangents are parallel to x-axis lie on the parabola.
View Solution