Let the set of all function f:[0,1]→R, which are continuous on [0,1] and differentiable on (0,1). Then for every f in S, there exists a c ∈(0,1) depending on f, such that :
|f(c)−f(1)|<(1−c)|f'(c)|
f(1)−f(c)1−c=f'(c)
|f(c)−f(1)|<|f'(c)|
|f(c)+f(1)|<(1+c)|f'(c)|
To solve the problem, we will use the Mean Value Theorem (MVT) which states that if a function is continuous on a closed interval (a, b) and differentiable on the open interval (a, b), then there exists at least one point c in (a, b) such that:
f′(c)=f(b)−f(a)b−a
In our case, we have the function f:(0,1)→R which is continuous on (0, 1) and differentiable on (0, 1). We can apply the MVT to the interval (0, 1).
1. Identify the function and the interval:
We have f defined on the interval (0, 1). According to the MVT, since f is continuous on (0, 1) and differentiable on (0, 1), we can apply the theorem.
2. Apply the Mean Value Theorem:
By the MVT, there exists a point c∈(0,1) such that:
f′(c)=f(1)−f(0)1−0=f(1)−f(0)
3. Interpret the result:
This means that the derivative of the function at the point c is equal to the difference in the function values at the endpoints of the interval divided by the length of the interval (which is 1 in this case).
4. Formulate the inequality:
We can also analyze the behavior of the derivative. Since f is continuous and differentiable, we can consider the slopes of secant lines and their relationship to the derivative at point c. Specifically, we can say:
|f(1)−f(c)|<(1−c)|f′(c)|
This inequality arises from the properties of the derivative and the continuity of f.
5. Conclusion:
Therefore, we conclude that for every function f in the set S, there exists a c∈(0,1) such that:
|f(1)−f(c)|<(1−c)|f′(c)|
We have f defined on the interval (0, 1). According to the MVT, since f is continuous on (0, 1) and differentiable on (0, 1), we can apply the theorem.
By the MVT, there exists a point c∈(0,1) such that:
f′(c)=f(1)−f(0)1−0=f(1)−f(0)
This means that the derivative of the function at the point c is equal to the difference in the function values at the endpoints of the interval divided by the length of the interval (which is 1 in this case).
We can also analyze the behavior of the derivative. Since f is continuous and differentiable, we can consider the slopes of secant lines and their relationship to the derivative at point c. Specifically, we can say:
|f(1)−f(c)|<(1−c)|f′(c)|
This inequality arises from the properties of the derivative and the continuity of f.
Therefore, we conclude that for every function f in the set S, there exists a c∈(0,1) such that:
|f(1)−f(c)|<(1−c)|f′(c)|
Topper's Solved these Questions
- JEE MAINBOOK - JEE MAINS PREVIOUS YEAR ENGLISHCHAPTER - JEE MAINEXERCISE - MATHEMATICS
121 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - JEE MAINS PREVIOUS YEAR ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - All Questions
2 Videos
- JEE MAINSBOOK - JEE MAINS PREVIOUS YEAR ENGLISHCHAPTER - JEE MAINSEXERCISE - All Questions
445 Videos
Similar Questions
If f(x) is continuous on [0,2] , differentiable in (0,2),f(0)=2,f(2)=8 and f'(x)≤3 for all x∈(0,2), then find the value of f(1).
View SolutionLet fandg be function continuous in [a,b]and differentiable on [a,b].If f(a)=f(b)=0 then show that there is a point c∈(a,b) such that g'(c)f(c)+f'(c)=0.
View SolutionIf f continuous and differentiable on [0,1] with f(0)= 0 and f(1)=1, then the minimum value of ∫10(f′(x))2dx is
View SolutionLeg f be continuous on the interval [0,1] to R such that f(0)=f(1). Prove that there exists a point c in [0,12] such that f(c)=f(c+12).
View SolutionLet f:[0,2]→R be a function which is continuous on [0,2] and is differentiable on (0,2) with f(0)=1 Let:F(x)=∫x20f(√t)dtforx∈[0,2].IfF′(x)=f′(x) . for all x∈(0,2), then F(2) equals (a)e2−1 (b) e4−1 (c)e−1 (d) e4
View SolutionIf f is a continuous function on [0,1], differentiable in (0, 1) such that f(1)=0, then there exists some c∈(0,1) such that cf′(c)−f(c)=0 cf′(c)+cf(c)=0 f′(c)−cf(c)=0 cf′(c)+f(c)=0
View SolutionLet f be a real-valued function defined on interval (0,∞),by f(x)=lnx+∫x0√1+sint.dt. Then which of the following statement(s) is (are) true? (A). f"(x) exists for all ∈ (0,∞). (B). f'(x) exists for all x ∈ (0,∞) and f' is continuous on (0,∞), but not differentiable on (0,∞). (C). there exists α>1 such that |f'(x)|<|f(x)| for all x ∈ (α,∞). (D). there exists β>1 such that |f(x)|+|f'(x)|≤β for all x ∈ (0,∞).
View SolutionIf f(x)=√1−√1−x2 , then f(x) is (a) continuous on [-1, 1] and differentiable on (-1, 1) (b) continuous on [-1, 1] and differentiable on ( − 1 , 0 ) ∪ ( 0 , 1 ) (c) continuous and differentiable on [-1, 1] (d) none of these
View SolutionLet f(x)be continuous on [a,b], differentiable in (a,b) andf(x)≠0for allx∈[a,b]. Then prove that there exists one c∈(a,b)such thatf'(c)f(c)=1a−c+1b−c.
View SolutionLet f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
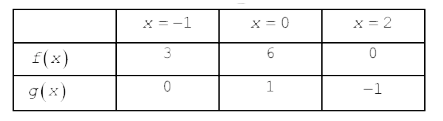
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View Solution
