Ship A moving with velocity →V1=30ˆi+50ˆj from position (0, 0) and ship B moving with velocity →V2=−10ˆi from position (80, 150). The time for minimum separation is : (A) 2.6 (B) 2.2 (C) 2.4 (D) None
To find the time for minimum separation between the two ships, we will follow these steps:
Step 1: Define the positions and velocities of the ships
- Ship A is moving with a velocity:
→V1=30^i+50^j
from the position (0, 0).
- Ship B is moving with a velocity:
→V2=−10^i
from the position (80, 150).
Step 2: Determine the relative velocity
The relative velocity of ship B with respect to ship A is given by:
→VBA=→V2−→V1
Substituting the values:
→VBA=(−10^i)−(30^i+50^j)=−40^i−50^j
Step 3: Determine the initial position vector of ship B with respect to ship A
The initial position of ship B relative to ship A is:
→RBA=(80−0)^i+(150−0)^j=80^i+150^j
Step 4: Find the time for minimum separation
The minimum separation occurs when the relative position vector is perpendicular to the relative velocity vector. This can be found using the dot product:
→RBA⋅→VBA=0
Calculating the dot product:
(80^i+150^j)⋅(−40^i−50^j)=80⋅(−40)+150⋅(−50)
=−3200−7500=−10700
Step 5: Calculate the magnitude of the relative velocity
The magnitude of the relative velocity is:
|→VBA|=√(−40)2+(−50)2=√1600+2500=√4100
Step 6: Calculate the time for minimum separation
The time t for minimum separation can be calculated using:
t=|→RBA⋅→VBA||→VBA|2
Substituting the values:
t=107004100≈2.6
Final Answer
The time for minimum separation is approximately 2.6 hours.
- Ship A is moving with a velocity:
→V1=30^i+50^j
from the position (0, 0).
- Ship B is moving with a velocity:
→V2=−10^i
from the position (80, 150).
The relative velocity of ship B with respect to ship A is given by:
→VBA=→V2−→V1
Substituting the values:
→VBA=(−10^i)−(30^i+50^j)=−40^i−50^j
The initial position of ship B relative to ship A is:
→RBA=(80−0)^i+(150−0)^j=80^i+150^j
The minimum separation occurs when the relative position vector is perpendicular to the relative velocity vector. This can be found using the dot product:
→RBA⋅→VBA=0
Calculating the dot product:
(80^i+150^j)⋅(−40^i−50^j)=80⋅(−40)+150⋅(−50)
=−3200−7500=−10700
The magnitude of the relative velocity is:
|→VBA|=√(−40)2+(−50)2=√1600+2500=√4100
The time t for minimum separation can be calculated using:
t=|→RBA⋅→VBA||→VBA|2
Substituting the values:
t=107004100≈2.6
The time for minimum separation is approximately 2.6 hours.
Similar Questions
A portion is fired from origin with velocity →v=v0ˆj+v0ˆk in a uniform magnetic field →B=B0ˆj. In the subsequent motion of the proton
View SolutionA conductor AB of length l moves in xy plane with velocity →v=v0(ˆi−ˆj). A magnetic field →B=B0(ˆi+ˆj) exists in the region. The induced emf is
View Solution
Knowledge Check
If →a=3ˆi+ˆj+2ˆkand→b=2ˆi−2ˆj+4ˆk, then the magnitude of →b×→a is
A8B−8C8√3DNone of these
In a region of space , suppose there exists a uniform electric field →E=10ˆi(Vm) . If a positive charge moves with a velocity →v=−2ˆj, its potential energy
View SolutionA particle travels so that its acceleration is given by →a=5costˆi−3sintˆj. If the particle is located at (−3,2) at time t=0 and is moving with a velocity given by (−3ˆi+2ˆj). Find
(i) The velocity [→v=∫→a.dt] at time t and
(ii) The position vector [→r=∫→v.dt] of the particle at time t(t>0).
View SolutionA particle is moving with a constant acceleration →a=ˆi−2ˆjm/s2 and instantaneous velocity →v=ˆi+2ˆj+2ˆkm/s then rate of change of speed of particle 1 second after this instant will be :
View SolutionIf →F=ˆi+2ˆj+ˆkand→V=4ˆi−ˆj+7ˆk what is →F.→v ?
View SolutionA magnetic field →B=B0ˆj , exists in the region a<x<2a , and →B=−B0ˆj , in the region 2a<x<3a, where B0 is a positive constant . A positive point charge moving with a velocity →v=v0ˆi , where v0 is a positive constant , enters the magnetic field at x=a . The trajectory of the charge in this region can be like
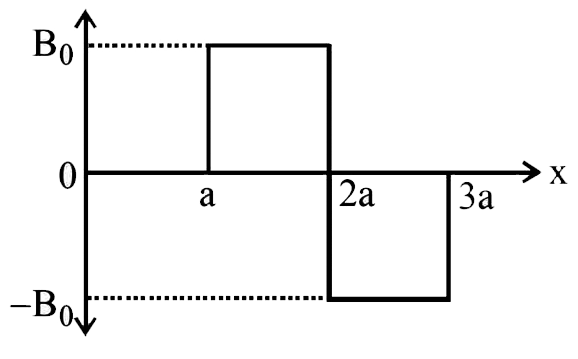
View SolutionA charged particle moves with velocity →v=aˆi+dˆj in a magnetic field →B=Aˆi+Dˆj. The force acting on the particle has magnitude F. Then,
View SolutionA charged particle moves with velocity →v=aˆi+dˆj in a magnetic field →B=Aˆi+Dˆj. The force acting on the particle has magnitude F. Then,
View Solution
