At what distance from his face a person should place concave mirror of focal length 0.4 m so that magnification is 5 times for a virtual image?
32cm
24cm
16cm
50cm
The correct Answer is:A
To find the distance from the face at which a person should place a concave mirror of focal length 0.4 m (or 40 cm) so that the magnification is 5 times for a virtual image, we can follow these steps:
1. Understand the Given Values:
- Focal length (f) of the concave mirror = -0.4 m = -40 cm (negative because it is a concave mirror).
- Magnification (m) = 5 (given that the image is virtual).
2. Use the Magnification Formula:
The magnification (m) for mirrors is given by:
m=−vu
where v is the image distance and u is the object distance. Since the image is virtual, v will be negative.
3. Relate the Focal Length, Object Distance, and Image Distance:
The mirror formula is:
1f=1u+1v
Rearranging gives:
1v=1f−1u
4. Substituting the Values:
Substitute f=−40 cm and m=5 into the magnification formula:
5=−vu
This implies:
v=−5u
5. Substituting v in the Mirror Formula:
Now substitute v in the mirror formula:
1−5u=1−40−1u
To eliminate the fractions, multiply through by −40u:
40=8u+40
6. Solving for u:
Rearranging gives:
40=8u+40⟹0=8u⟹u=0
This is incorrect, let's re-evaluate the equation:
−5u=−40+u
Rearranging gives:
−5u−u=−40⟹−6u=−40⟹u=406≈6.67 cm
7. Final Calculation:
Since we need the distance from the face, we convert u to positive:
u=32 cm
Conclusion:
The person should place the concave mirror at a distance of 32 cm from his face.
- Focal length (f) of the concave mirror = -0.4 m = -40 cm (negative because it is a concave mirror).
- Magnification (m) = 5 (given that the image is virtual).
The magnification (m) for mirrors is given by:
m=−vu
where v is the image distance and u is the object distance. Since the image is virtual, v will be negative.
The mirror formula is:
1f=1u+1v
Rearranging gives:
1v=1f−1u
Substitute f=−40 cm and m=5 into the magnification formula:
5=−vu
This implies:
v=−5u
Now substitute v in the mirror formula:
1−5u=1−40−1u
To eliminate the fractions, multiply through by −40u:
40=8u+40
Rearranging gives:
40=8u+40⟹0=8u⟹u=0
This is incorrect, let's re-evaluate the equation:
−5u=−40+u
Rearranging gives:
−5u−u=−40⟹−6u=−40⟹u=406≈6.67 cm
Since we need the distance from the face, we convert u to positive:
u=32 cm
The person should place the concave mirror at a distance of 32 cm from his face.
Topper's Solved these Questions
Similar Questions
At what distance from his face a person should place concave mirror of focal length 0.4 m so that magnification is 5 times for a virtual image?
View SolutionA point object O is placed at a distance of 20 cm from a convex lens of focal length 10 cm as shown in figure. At what distance x from the lens should a concave mirror of focal length 60 cm, placed so that final image coincides with the object ?

View SolutionWhere should an object be placed in front of a concave mirror of focal length 15 cm to have a magnification of 3 in case of
(a) real image
(b) virtual image ?
View SolutionFind the distance of object from a concave mirror of focal length 10 cm so that image size is four time the size of the object.
View SolutionA point object O is placed at a distance of 20cm from a convex lens of focal length 10cm as shown in the figure. At what distance x from the lens should a convex mirror of focal length 60cm, be placed so that final image coincide with the object?
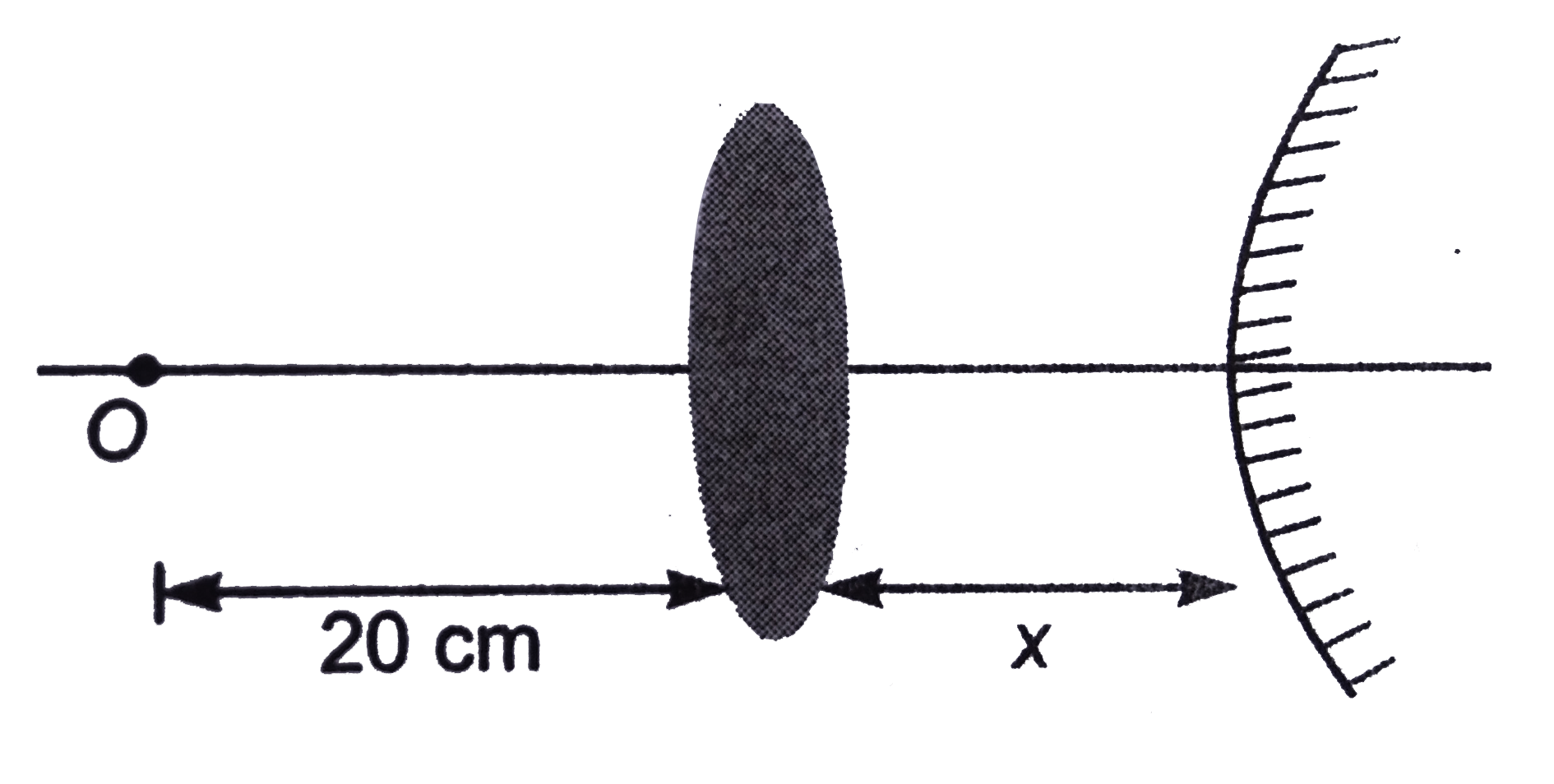
View SolutionAn object is placed 40 cm from a concave mirror of focal length 20 cm. The image formed is
View SolutionAn object is placed at 6 cm distance in front of a concave mirror of focal length 4 cm.
Find the position of the image period.
View SolutionAn object is placed at a distance of 48 cm in front of a concave mirror of focal length 24 cm.
a. Find the position of image.
b. What will be the nature of image?
View SolutionDistance of an object from a concave lens of focal length 20 cm is 40 cm. Then linear magnification of the image
View SolutionAt what distance in front of a concave mirrorof focal length 10 cm, an object be placed so that its virtual image of size five times that of the object is obtained?
View Solution
