A (3,0,−1),B(2,10,6) and (1,2,1) are the vertices of a triangle. M is the mid point of the line segment joining AC and G is a point on line segment BM dividing 2:1 ratio internally find cos(∠GOA)
2√5
1√15
1√10
1√3
The correct Answer is:B
Step by step video & image solution for A (3,0,-1),B(2,10,6) and (1,2,1) are the vertices of a triangle. M is the mid point of the line segment joining AC and G is a point on line segment BM dividing 2:1 ratio internally find cos(angleGOA) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Find the mid point of the line segment joining (3,6) and (5,-2).
View SolutionThe mid point of the line segment joining the points (−5,7)and(−1,3) is
View SolutionFind the mid-point of the line segment joining the points :
(5, -3) and (-1, 7)
View SolutionLet A(3,0,-1) ,B(2,10,6) and C(1,2,1) be the vertices of a triangle and M be the mid- point of AC.
If G divides BM in the ratio 2:1 then cos (∠GOA) (O being the origin) is equal to
View SolutionFind the co-ordinates of the mid point of the line segment joining the points P (4, -6) and (-2, 4).
View SolutionFind the co-ordinates of the mid-point of the line segment joining the points A(3,−5)andB(1,1).
View SolutionThe mid-point of the line segment joining (4a, 23) and (-4, 3b) is (2, -2a). Find the values of a and b
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
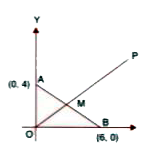
co-ordinates of M
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
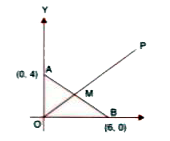
co-ordinates of P
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
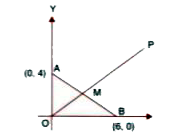
length of BP
View Solution
