Question

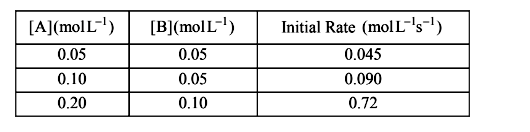
For the reaction $2A+B\to C$, the values of initial rate at different reactant concentrations are given in the table below. The rate law for the reaction is : $\begin{array}{ccc}\left[A\right]\left(\text{mol}{L}^{-1}\right)& \left[B\right]\left(\text{mol}{L}^{-1}\right)& \begin{array}{c}\text{Initial Rate}\\ {\text{mol}}^{-1}{s}^{-1}\end{array}\\ 0.05& 0.05& 0.045\\ 0.10& 0.05& 0.090\\ 0.20& 0.10& 0.72\end{array}$

Options

A. Rate`=K[A][B]^(2)`
B. Rate=`k[A]^(2)[B]^(2)`
C. Rate`=K[A][B]`
D. Rate `=k[A]^(2)[B]`

Answer

B

Explanation

To determine the rate law for the reaction $$2A+B\to C$$ based on the provided initial rates and concentrations, we will follow these steps:

Step 1: Write the general form of the rate law
The rate law can be expressed as:
\( \text{Rate} = k (A)^p (B)^q \)
where \(k\) is the rate constant, \(p\) is the order with respect to reactant \(A\), and \(q\) is the order with respect to reactant \(B\).

Step 2: Analyze the data from the table
The initial rates and concentrations are given as follows:

| (A) (mol L\(^{-1}\)) | (B) (mol L\(^{-1}\)) | Initial Rate (mol L\(^{-1}\) s\(^{-1}\)) |
|-----------------------|-----------------------|-------------------------------------------|
| 0.05 | 0.05 | 0.045 |
| 0.10 | 0.05 | 0.090 |
| 0.20 | 0.10 | 0.72 |

Step 3: Set up equations using the data
Using the first experiment:
\( 0.045 = k (0.05)^p (0.05)^q \quad \text{(Equation 1)} \)

Using the second experiment:
\( 0.090 = k (0.10)^p (0.05)^q \quad \text{(Equation 2)} \)

Using the third experiment:
\( 0.72 = k (0.20)^p (0.10)^q \quad \text{(Equation 3)} \)

Step 4: Divide equations to eliminate \(k\)
To find \(p\), divide Equation 2 by Equation 1:
\( \frac{0.090}{0.045} = \frac{k (0.10)^p (0.05)^q}{k (0.05)^p (0.05)^q} \)
This simplifies to:
\( 2 = \frac{(0.10)^p}{(0.05)^p} \implies 2 = 2^p \implies p = 1 \)

Step 5: Find \(q\)
Now, we will use the value of \(p\) to find \(q\). Divide Equation 3 by Equation 2:
\( \frac{0.72}{0.090} = \frac{k (0.20)^p (0.10)^q}{k (0.10)^p (0.05)^q} \)
This simplifies to:
\( 8 = \frac{(0.20)^1 (0.10)^q}{(0.10)^1 (0.05)^q} \)
\( 8 = \frac{0.20}{0.10} \cdot \frac{(0.10)^q}{(0.05)^q} \implies 8 = 2 \cdot \left(\frac{0.10}{0.05}\right)^q \)
\( 8 = 2 \cdot 2^q \implies 4 = 2^q \implies q = 2 \)

Step 6: Write the final rate law
Now that we have \(p = 1\) and \(q = 2\), we can write the rate law:
\( \text{Rate} = k (A)^1 (B)^2 \implies \text{Rate} = k (A) (B)^2 \)

Conclusion
The rate law for the reaction \(2A + B \rightarrow C\) is:
\( \text{Rate} = k (A) (B)^2 \)