Question

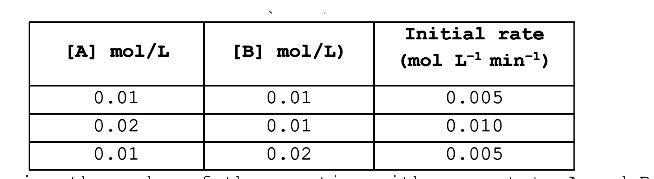
Rate of a reaction $A+B\to$ Product, is given as a function of different initial concentration of $A$ and $B$.
$\left|\begin{array}{cccc}\left[A\right]\left(mol{L}^{-1}\right)& \left(B\right)\left(mol{L}^{-1}\right)& \text{Initial rate}\left(mol{L}^{-1}\stackrel{-1}{min}\right)& \\ 0.01& 0.01& 0.005& \\ 0.02& 0.01& 0.010& \\ 0.01& 0.02& 0.005& \end{array}\right|$
Determine the order of the reaction with respect to $A$ and with respect to $B$. What is the half life of $A$ in the reaction ?

Explanation

To determine the order of the reaction with respect to A and B, and to find the half-life of A, we can follow these steps:

Step 1: Write the Rate Law
The rate law for the reaction can be expressed as:
\( \text{Rate} = k(A)^x(B)^y \)
where \( x \) is the order with respect to A and \( y \) is the order with respect to B.

Step 2: Set Up the Experiments
We have the following data from the experiments:

| (A) (mol L^(-1)) | (B) (mol L^(-1)) | Initial Rate (mol L^(-1) min^(-1)) |
|-------------------|-------------------|-------------------------------------|
| 0.01 | 0.01 | 0.005 |
| 0.02 | 0.01 | 0.010 |
| 0.01 | 0.02 | 0.005 |

Step 3: Determine the Order with Respect to A
Using the first and second experiments:
\( \frac{\text{Rate}_1}{\text{Rate}_2} = \frac{0.005}{0.010} = \frac{(A)_1^x (B)_1^y}{(A)_2^x (B)_2^y} \)

Substituting the concentrations:
\( \frac{0.005}{0.010} = \frac{(0.01)^x (0.01)^y}{(0.02)^x (0.01)^y} \)

This simplifies to:
\( \frac{1}{2} = \frac{(0.01)^x}{(0.02)^x} \)
\( \frac{1}{2} = \left(\frac{0.01}{0.02}\right)^x \)
\( \frac{1}{2} = \left(\frac{1}{2}\right)^x \)

From this, we can conclude:
\( x = 1 \)

Step 4: Determine the Order with Respect to B
Using the first and third experiments:
\( \frac{\text{Rate}_1}{\text{Rate}_3} = \frac{0.005}{0.005} = \frac{(A)_1^x (B)_1^y}{(A)_3^x (B)_3^y} \)

Substituting the concentrations:
\( 1 = \frac{(0.01)^x (0.01)^y}{(0.01)^x (0.02)^y} \)

This simplifies to:
\( 1 = \frac{(0.01)^y}{(0.02)^y} \)
\( 1 = \left(\frac{0.01}{0.02}\right)^y \)
\( 1 = \left(\frac{1}{2}\right)^y \)

From this, we can conclude:
\( y = 0 \)

Step 5: Write the Rate Law
Now we can write the rate law:
\( \text{Rate} = k(A)^1(B)^0 \)
or simply:
\( \text{Rate} = k(A) \)

Step 6: Calculate the Rate Constant (k)
Using the data from the first experiment:
\( \text{Rate} = k(A) \)
\( 0.005 = k(0.01) \)
\( k = \frac{0.005}{0.01} = 0.5 \, \text{min}^{-1} \)

Step 7: Calculate the Half-Life of A
For a first-order reaction, the half-life \( t_{1/2} \) is given by:
\( t_{1/2} = \frac{0.693}{k} \)
Substituting the value of \( k \):
\( t_{1/2} = \frac{0.693}{0.5} = 1.386 \, \text{minutes} \)

Final Answer
- The order of the reaction with respect to A is 1.
- The order of the reaction with respect to B is 0.
- The half-life of A in the reaction is 1.386 minutes.