A metal wire of resistance is elongated to make a unform wire of double its previous length. This new wire is now bent and the ends joined to make a circle. If two points on this circle make an angle at the centre, the equivalent resistance between these two points will be :
The correct Answer is:A
To solve the problem step by step, we will follow the reasoning provided in the video transcript.
Step 1: Determine the new resistance after elongation
The original resistance of the wire is given as R=3Ω. When the wire is elongated to double its length, the new length L′ becomes 2L. Since the volume of the wire remains constant, the cross-sectional area A′ must change.
Using the formula for resistance:
R=ρLA
If the length doubles, the area must halve to keep the volume constant:
A′=A2
Now, substituting the new values into the resistance formula:
R′=ρ2LA/2=ρ4LA=4R
Since R=3Ω:
R′=4×3=12Ω
Step 2: Understand the circular wire and angle
The wire is then bent to form a circle. The total resistance of the circular wire is 12Ω. When two points on the circle make an angle of 60∘ at the center, we need to find the equivalent resistance between these two points.
Step 3: Calculate the lengths of the wire segments
The angle 60∘ corresponds to 16 of the total circle (since 360∘ is a full circle):
Length of arc PQ=60360×Circumference=16×2πr=πr3
The remaining arc (the rest of the circle) will be:
Remaining arc=2πr−πr3=6πr3−πr3=5πr3
Step 4: Calculate the resistances of the segments
The resistance is proportional to the length of the wire:
- For the arc PQ (length πr3):
RPQ=12Ω2πr×πr3=126=2Ω
- For the remaining arc (length 5πr3):
Rremaining=12Ω2πr×5πr3=12×56=10Ω
Step 5: Calculate the equivalent resistance
The two resistances RPQ and Rremaining are in parallel:
1Req=1RPQ+1Rremaining=12+110
Finding a common denominator (10):
1Req=510+110=610=35
Thus,
Req=53Ω
Final Answer
The equivalent resistance between the two points on the circle is:
53Ω
The original resistance of the wire is given as R=3Ω. When the wire is elongated to double its length, the new length L′ becomes 2L. Since the volume of the wire remains constant, the cross-sectional area A′ must change.
R=ρLA
If the length doubles, the area must halve to keep the volume constant:
A′=A2
R′=ρ2LA/2=ρ4LA=4R
Since R=3Ω:
R′=4×3=12Ω
The wire is then bent to form a circle. The total resistance of the circular wire is 12Ω. When two points on the circle make an angle of 60∘ at the center, we need to find the equivalent resistance between these two points.
The angle 60∘ corresponds to 16 of the total circle (since 360∘ is a full circle):
Length of arc PQ=60360×Circumference=16×2πr=πr3
Remaining arc=2πr−πr3=6πr3−πr3=5πr3
The resistance is proportional to the length of the wire:
- For the arc PQ (length πr3):
RPQ=12Ω2πr×πr3=126=2Ω
Rremaining=12Ω2πr×5πr3=12×56=10Ω
The two resistances RPQ and Rremaining are in parallel:
1Req=1RPQ+1Rremaining=12+110
1Req=510+110=610=35
Thus,
Req=53Ω
The equivalent resistance between the two points on the circle is:
53Ω
Topper's Solved these Questions
Similar Questions
A metal wire of resistance 6Ω is stretched so that its length is increased to twice its original length. Calculate its new resistance.
View SolutionA uniform wire of resistance =3Ω and length l is stretched to double its length. Now it is bent to form a circular loop and two point P&Q lies on the loop such that they subtend 60∘ angle at centre. The equivalent resistance between two point P & Q is:
View Solution
Knowledge Check
- Question 1 - Select One
A uniform wire of resistance 36Ω is bent in the form of a circle. The effective resistance across the points A and B is
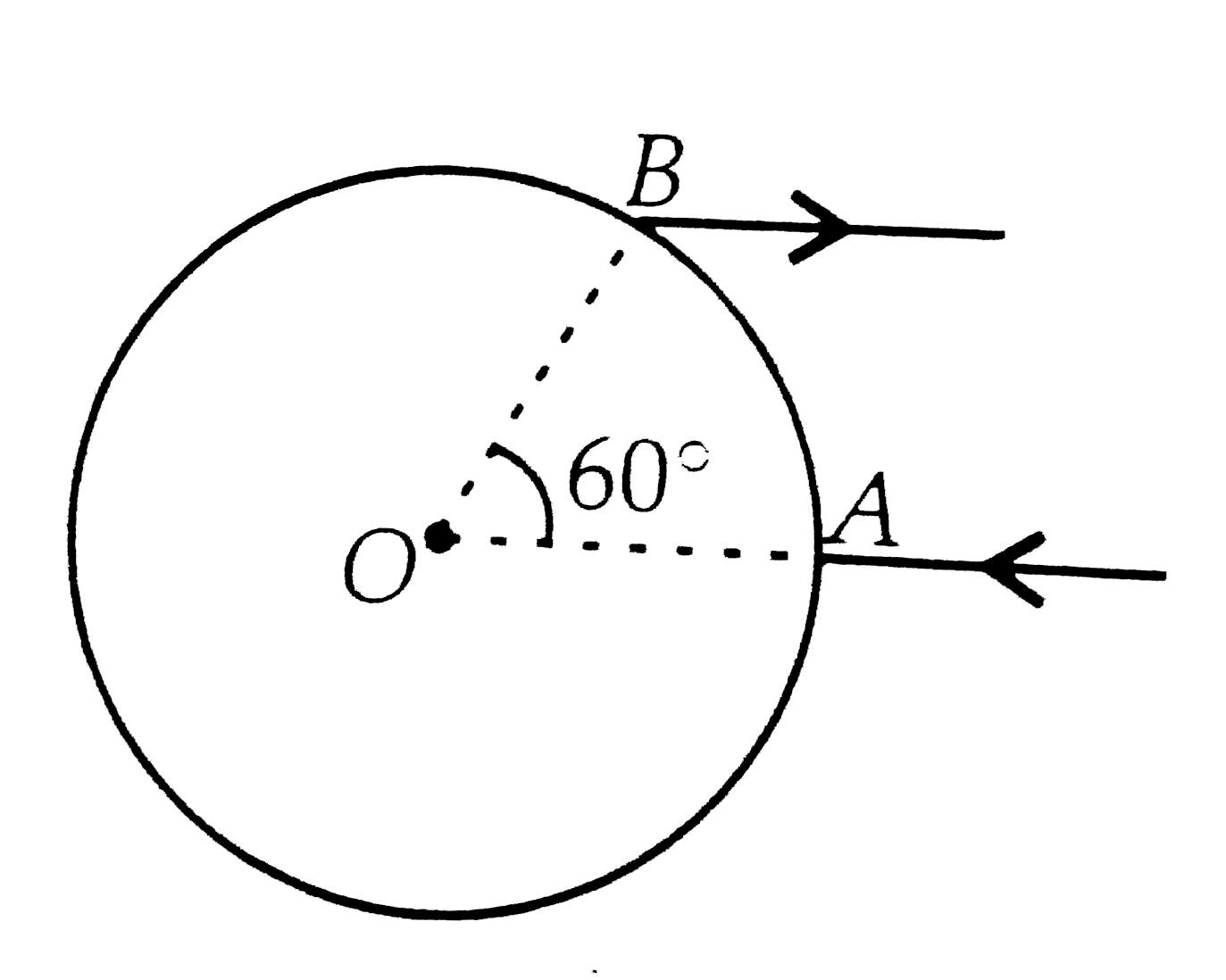 A5ΩB15ΩC7.2ΩD30Ω
A5ΩB15ΩC7.2ΩD30Ω
A metal wire has a resistance of 35Ω. If its length is increased to double by drawing it, then its new resistance will be
View SolutionA wire of resistance 4Ω is bent to form a circle. The resistance point A and B will be
_PHY_E05_303_Q01.png) View Solution
View SolutionA metal wire of resistance 62 Ohms is stretched so that its length increased to twice of original length. Calculate its new resistance
View SolutionA wire is bent in the form of a triangle now the equivalent resistance between its one end and the mid point of the side is
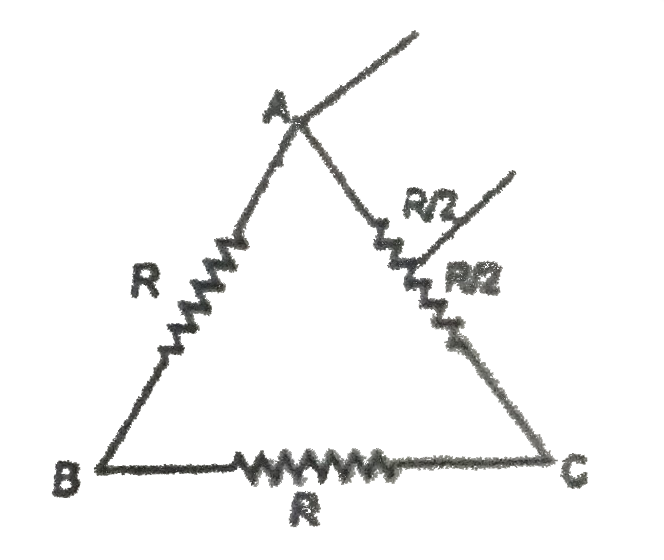 View Solution
View SolutionA given wire of resistance 1 Ω is stretched to double its length. What will be its new resistance ?
View SolutionA uniform wire of resistance 36Ω is bent in the form of a circle. The Effective resistance between A and B is (O is the centre of circle):
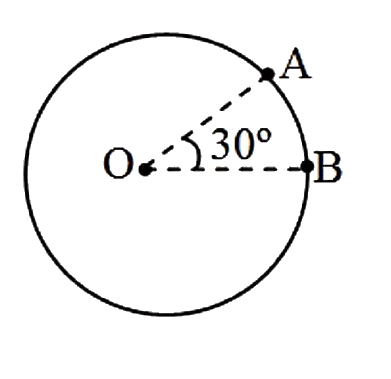 View Solution
View SolutionA uniform wire of resistance 18Ω is bent in the form of a circle. The effective resistance across the points a and b is
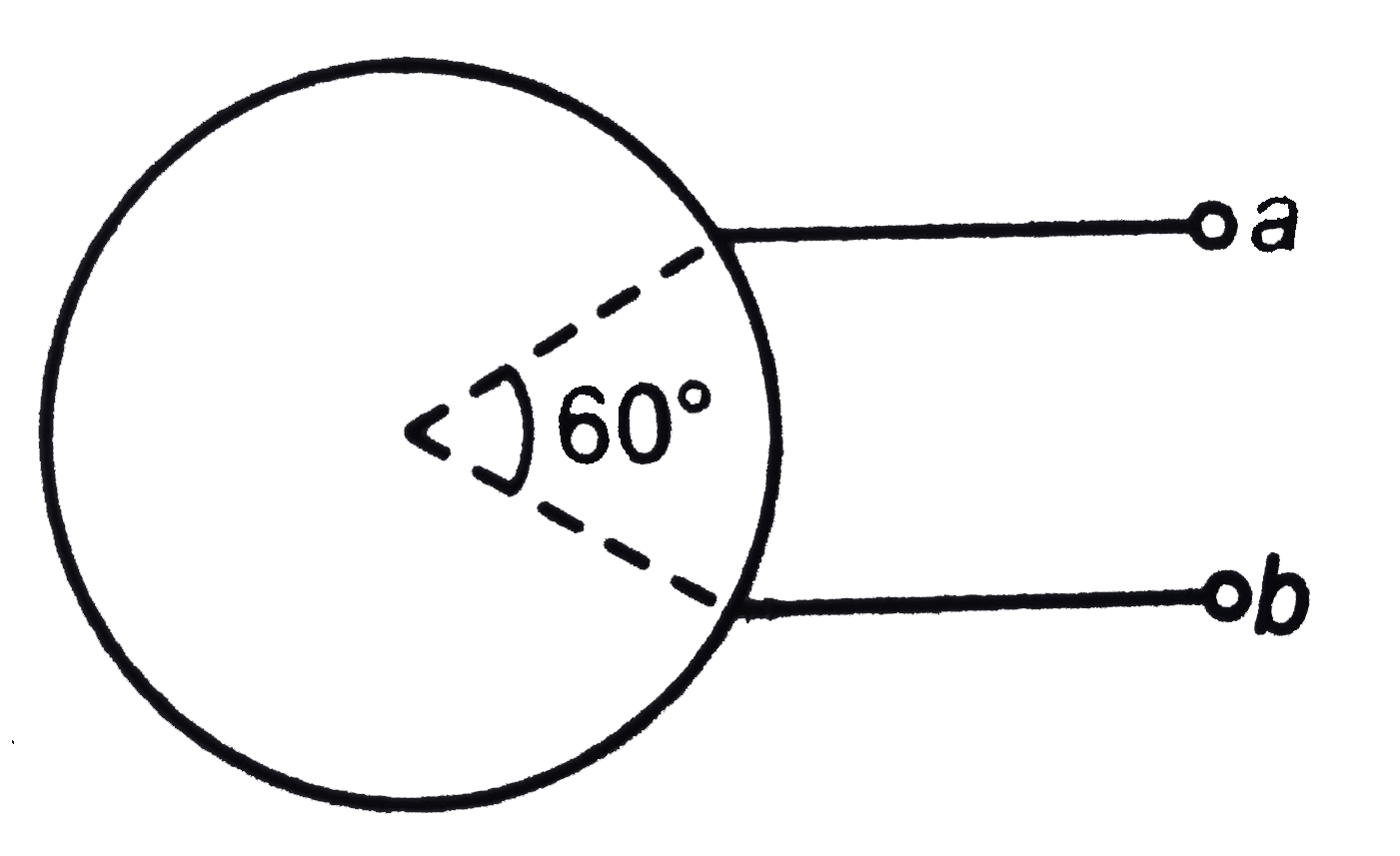 View Solution
View Solution

