Four points charges -q,+q,+q and -q are placed on y-axis at y=-2d, y=-d,y=+d and y=+2d, respectively. The magnitude of the electric field E at a point on the x-axis at x=D, with D>>d, will behave as :
E∝1D3
E∝1D
E∝1D4
E∝1D2
The correct Answer is:A
To solve the problem, we need to calculate the electric field E at a point on the x-axis at x=D due to the four point charges located on the y-axis. The charges are positioned at y=−2d (charge −q), y=−d (charge +q), y=+d (charge +q), and y=+2d (charge −q).
Step 1: Identify the distances from the point on the x-axis to each charge
The point on the x-axis is at (D,0). The distances from this point to each charge can be calculated using the Pythagorean theorem:
1. For the charge at y=−2d:
r1=√D2+(2d)2=√D2+4d2
2. For the charge at y=−d:
r2=√D2+d2
3. For the charge at y=+d:
r3=√D2+d2
4. For the charge at y=+2d:
r4=√D2+4d2
Step 2: Calculate the electric field due to each charge
The electric field E due to a point charge is given by:
E=k|q|r2
where k is Coulomb's constant.
1. Electric field due to the charge at y=−2d (charge −q):
E1=kqr21=kqD2+4d2
2. Electric field due to the charge at y=−d (charge +q):
E2=kqr22=kqD2+d2
3. Electric field due to the charge at y=+d (charge +q):
E3=kqr23=kqD2+d2
4. Electric field due to the charge at y=+2d (charge −q):
E4=kqr24=kqD2+4d2
Step 3: Determine the direction of the electric fields
- The electric field due to the negative charges (−q) will point towards the charge.
- The electric field due to the positive charges (+q) will point away from the charge.
Step 4: Calculate the net electric field at point (D,0)
The net electric field E at point (D,0) is the vector sum of the individual electric fields. Since the electric fields due to +q charges at y=−d and y=+d are in the same direction (away from the charges), and the electric fields due to −q charges at y=−2d and y=+2d are in the opposite direction, we can express the net electric field as:
Enet=E2+E3−E1−E4
Substituting the expressions for E1,E2,E3, and E4:
Enet=kqD2+d2+kqD2+d2−kqD2+4d2−kqD2+4d2
This simplifies to:
Enet=2kqD2+d2−2kqD2+4d2
Step 5: Factor out common terms
Factoring out 2kq:
Enet=2kq(1D2+d2−1D2+4d2)
Step 6: Simplify the expression
To combine the fractions:
Enet=2kq((D2+4d2)−(D2+d2)(D2+d2)(D2+4d2))
This simplifies to:
Enet=2kq(3d2(D2+d2)(D2+4d2))
Step 7: Analyze the behavior as D≫d
As D becomes much larger than d, we can approximate:
Enet≈6kqd2D4
Thus, the electric field E behaves as:
E∝1D4
Conclusion
The magnitude of the electric field E at the point on the x-axis at x=D behaves as inversely proportional to D4.
r1=√D2+(2d)2=√D2+4d2
r2=√D2+d2
r3=√D2+d2
r4=√D2+4d2
E=k|q|r2
where k is Coulomb's constant.
E1=kqr21=kqD2+4d2
E2=kqr22=kqD2+d2
E3=kqr23=kqD2+d2
E4=kqr24=kqD2+4d2
- The electric field due to the positive charges (+q) will point away from the charge.
Topper's Solved these Questions
Similar Questions
Point charges +4q,−q and +4q are kept on the x-axis at points x=0,x=a and x=2a respectively, then
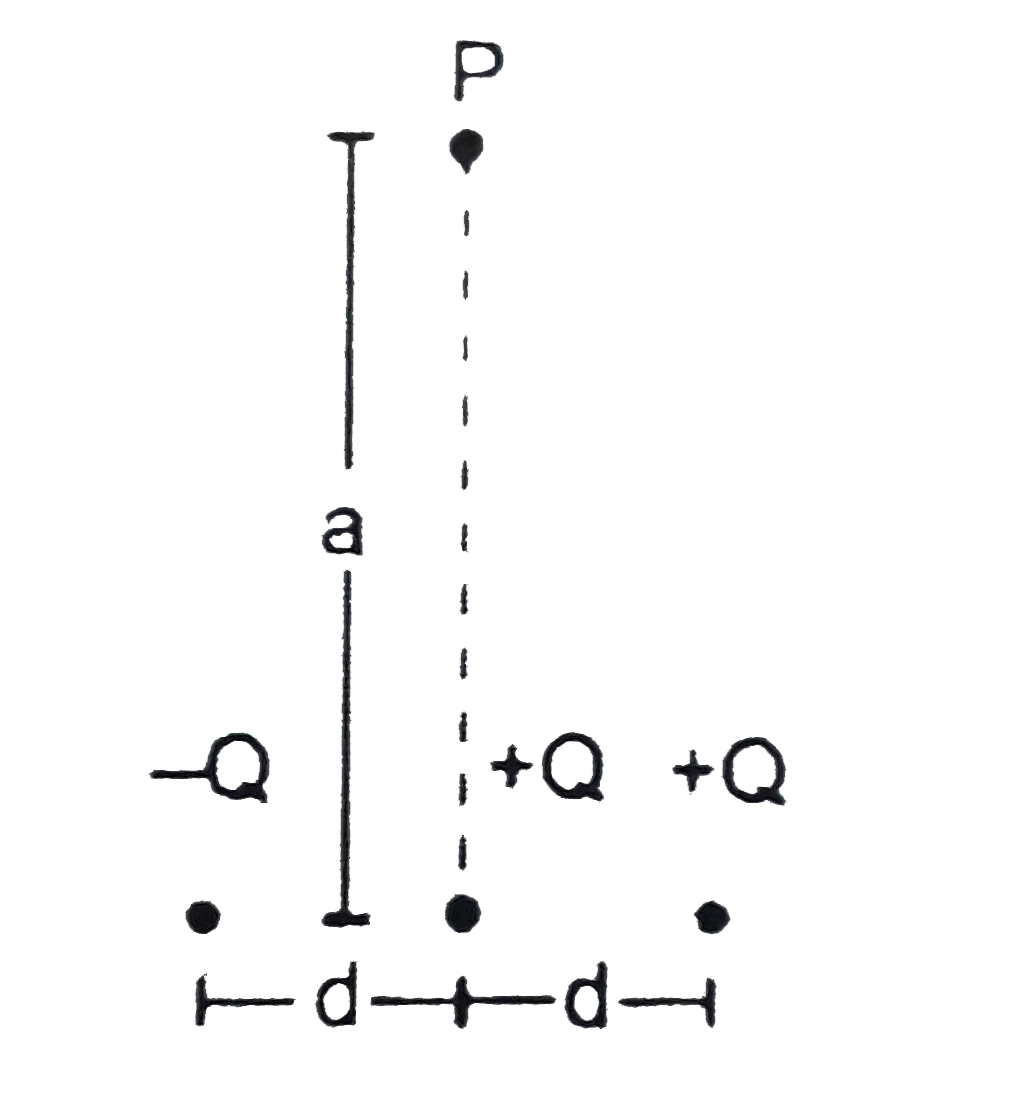
View SolutionThree point charges -Q, Q and Q are placed on a straight line with distance d between charges as shown. Find the magnitude of the electric field at the point P in the configuration shown which is at a distance a from middle charge Q in the system provided that a>>d. Take 2Qd=p.
View SolutionTwo point charges q and –q are at positions (0,0,d) and (0,0, –d) respectively . What is the electric field at (a,0,0 ) ?
View SolutionTwo point charges q and –q are at positions (0,0,d) and (0,0, –d) respectively . What is the electric field at (a,0,0 ) ?
View SolutionIn the figure, two point charges +qand−q are placed on the x-axis at (+a,0)and(−a,0) respectively. A tiny dipole of dipolem moment (p) is kept at the origin along the y-axis. The torque on the dipole equals

View SolutionTwo point charges +8q and −2q are located at x=0 and x=L respectively. The location of a point on the x axis at which the net electric field due to these two point charges is zero is
View SolutionFour charges of +q, +q +q and +q are placed at the corners A, B, C and D of s square. The resultant force on the charge at D
View SolutionThe point charges +q,−2q and +q are placed at point (x=0,y=a,z=0),(x=0,y=0,z=0) and (x=a,y=0,z=0), repectively. The magnitude and direction of the electric dipole moment vector of this charge assembly are
View SolutionTwo charges q1andq2 are placed at (0,0,d) and (0,0,-d) respectively. Find locus of points where the potential is zero.
View SolutionTwo point charges of magnitude +qand−q are placed at (−d/2,0,0)and(d/2,0,0) are respectively. Find the equation of the euipotential surface where the potential is zero.
View Solution
