A thin disc of mass M and radius R has mass per unit area σ(r)=kr2 where r is the distance from its centre. Its moment of inertia about an axis going through its centre of mass and perpendicular to its plane is : a) MR33 b) 2MR23 c) MR26 d) MR22
MR33
2MR23
MR26
MR22
The correct Answer is:A
To find the moment of inertia of a thin disc with a mass per unit area given by σ(r)=kr2, we can follow these steps:
Step 1: Define the mass element
Consider a thin ring of radius r and thickness dr within the disc. The area of this ring is given by:
dA=2πrdr
The mass of this ring, dm, can be expressed in terms of the mass per unit area:
dm=σ(r)⋅dA=kr2⋅(2πrdr)=2πkr3dr
Step 2: Define the moment of inertia of the ring
The moment of inertia dI of the thin ring about the axis perpendicular to the plane of the disc and through its center is given by:
dI=r2dm=r2⋅(2πkr3dr)=2πkr5dr
Step 3: Integrate to find the total moment of inertia
To find the total moment of inertia I of the disc, we integrate dI from 0 to R:
I=∫R0dI=∫R02πkr5dr
Calculating the integral:
I=2πk∫R0r5dr=2πk(r66)R0=2πk⋅R66=2πkR66=πkR63
Step 4: Find the total mass of the disc
Next, we need to find the total mass M of the disc:
M=∫R0dm=∫R02πkr3dr
Calculating this integral:
M=2πk∫R0r3dr=2πk(r44)R0=2πk⋅R44=πkR42
Step 5: Solve for k in terms of M
From the equation for total mass, we can express k:
k=2MπR4
Step 6: Substitute k back into the moment of inertia
Now substitute k back into the expression for I:
I=πkR63=π(2MπR4)R63=2MR63R4=2MR23
Final Result
Thus, the moment of inertia of the disc about the axis through its center and perpendicular to its plane is:
I=2MR23
Answer
The correct option is (b) 2MR23.
Consider a thin ring of radius r and thickness dr within the disc. The area of this ring is given by:
dA=2πrdr
The mass of this ring, dm, can be expressed in terms of the mass per unit area:
dm=σ(r)⋅dA=kr2⋅(2πrdr)=2πkr3dr
The moment of inertia dI of the thin ring about the axis perpendicular to the plane of the disc and through its center is given by:
dI=r2dm=r2⋅(2πkr3dr)=2πkr5dr
To find the total moment of inertia I of the disc, we integrate dI from 0 to R:
I=∫R0dI=∫R02πkr5dr
Calculating the integral:
I=2πk∫R0r5dr=2πk(r66)R0=2πk⋅R66=2πkR66=πkR63
Next, we need to find the total mass M of the disc:
M=∫R0dm=∫R02πkr3dr
Calculating this integral:
M=2πk∫R0r3dr=2πk(r44)R0=2πk⋅R44=πkR42
From the equation for total mass, we can express k:
k=2MπR4
Now substitute k back into the expression for I:
I=πkR63=π(2MπR4)R63=2MR63R4=2MR23
Thus, the moment of inertia of the disc about the axis through its center and perpendicular to its plane is:
I=2MR23
The correct option is (b) 2MR23.
Topper's Solved these Questions
Similar Questions
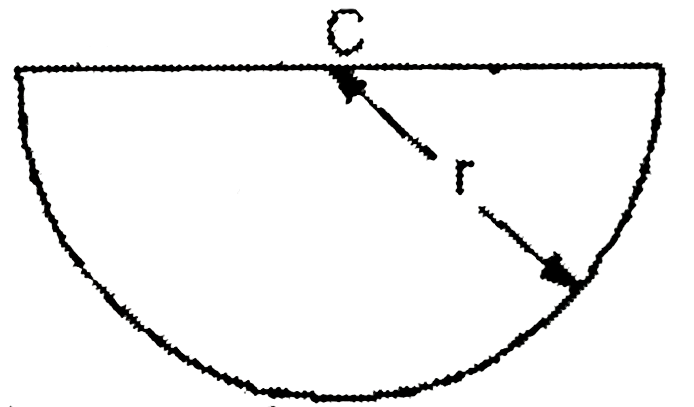
A semicircular lamina of mass m and radius r and centre C. Its center of mass is at a distance x from C. Its moment of inertia about an axis through its center of mass and perpendicular to its plane is:
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionA disc of mass m and radius R has a concentric hole of radius r. Its moment of inertia about an axis through its center and perpendicular to its plane is
View SolutionA disc of radius R has a concentric hole of radius R /2 and its mass is M. Its moment of inertia about an axis through its centre and perpendicular to its plane, is
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
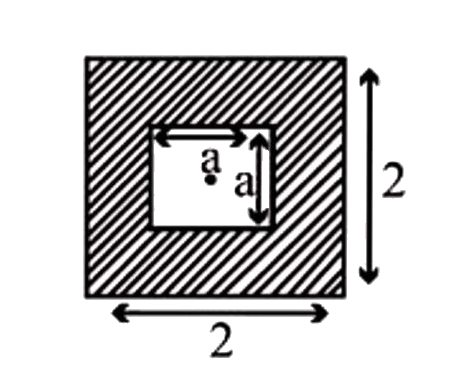
View SolutionA square of a side a is cut from a square of side 2a as shown in the figure. Mass of this square with a hole is M. Then its moment of inertia about an axis passing through its centre of mass and perpendicular to its plane will be
View SolutionThe moment of inertia of a uniform cicrular disc about its diameter is 200gcm2. Its moment of inertia about an axis passing through its centre and perpendicular to its circular face is
View SolutionThe moment of inertia of a uniform cicrular disc about its diameter is 200gcm2. Its moment of inertia about an axis passing through its centre and perpendicular to its circular face is
View SolutionA uniform thin bar of mass 6m and length 12L is bend to make a regular hexagon. Its moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the hexagon is :
View Solution
