A cubical block of side 0.5 M floats on water with 30% of its volume under water. What is the maximum weight that can be put on the block without fully submerging it under water?
[Take density of water =103kg/m3]
46.3kg
87.5 kg
65.4 kg
30.1 kg
To solve the problem, we need to determine the maximum weight that can be placed on the cubical block without fully submerging it in water. We will use the principles of buoyancy and the relationship between weight and the volume of water displaced.
1. Determine the volume of the cube:
The volume V of a cube is given by the formula:
V=side3
Given the side of the cube is 0.5m:
V=(0.5)3=0.125m3
2. Calculate the volume submerged:
Since 30% of the cube's volume is submerged, the volume submerged Vs is:
Vs=0.3×V=0.3×0.125=0.0375m3
3. Calculate the weight of the water displaced:
The weight of the water displaced is equal to the buoyant force acting on the block. The buoyant force Fb can be calculated using the formula:
Fb=Vs×ρw×g
where:
- ρw=1000kg/m3 (density of water)
- g=9.81m/s2 (acceleration due to gravity)
Substituting the values:
Fb=0.0375m3×1000kg/m3×9.81m/s2
Fb=367.875N
4. Convert buoyant force to mass:
To find the maximum mass m that can be placed on the block without submerging it, we can use the relationship between weight and mass:
Fb=(m+m0)×g
where m0 is the mass of the block itself. Since we are only interested in the additional mass that can be added without submerging, we can rearrange this to:
m=Fbg−m0
5. Calculate the mass of the block:
The mass of the block m0 can be calculated using the volume of the block and the density of the material (assuming it is just floating):
m0=V×ρblock
However, since we are not given the density of the block, we can assume it is just floating and thus the mass of the block is equal to the weight of the water displaced when 30% is submerged.
6. Calculate the maximum additional mass:
Since the block is floating with 30% submerged, we can assume the weight of the block is equal to the buoyant force:
m0=Fbg=367.8759.81≈37.5kg
Therefore, the maximum weight that can be added without fully submerging is:
m=Fbg−m0=37.5kg−37.5kg=0kg
Since we are looking for the total weight that can be added:
Maximum weight=Fb=367.875N
Final Answer:
The maximum weight that can be put on the block without fully submerging it under water is approximately 367.875 N.
The volume V of a cube is given by the formula:
V=side3
Given the side of the cube is 0.5m:
V=(0.5)3=0.125m3
Since 30% of the cube's volume is submerged, the volume submerged Vs is:
Vs=0.3×V=0.3×0.125=0.0375m3
The weight of the water displaced is equal to the buoyant force acting on the block. The buoyant force Fb can be calculated using the formula:
Fb=Vs×ρw×g
where:
- ρw=1000kg/m3 (density of water)
- g=9.81m/s2 (acceleration due to gravity)
Fb=0.0375m3×1000kg/m3×9.81m/s2
Fb=367.875N
To find the maximum mass m that can be placed on the block without submerging it, we can use the relationship between weight and mass:
Fb=(m+m0)×g
where m0 is the mass of the block itself. Since we are only interested in the additional mass that can be added without submerging, we can rearrange this to:
m=Fbg−m0
The mass of the block m0 can be calculated using the volume of the block and the density of the material (assuming it is just floating):
m0=V×ρblock
However, since we are not given the density of the block, we can assume it is just floating and thus the mass of the block is equal to the weight of the water displaced when 30% is submerged.
Since the block is floating with 30% submerged, we can assume the weight of the block is equal to the buoyant force:
m0=Fbg=367.8759.81≈37.5kg
m=Fbg−m0=37.5kg−37.5kg=0kg
Maximum weight=Fb=367.875N
The maximum weight that can be put on the block without fully submerging it under water is approximately 367.875 N.
Topper's Solved these Questions
Similar Questions
A rubber ball floats on water with its 1/3 rd volume outside water. What is the density of rubber?
View SolutionA rubber ball floats on water with its 1/3rd volume outside water. What is the density of rubber?
View SolutionA cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
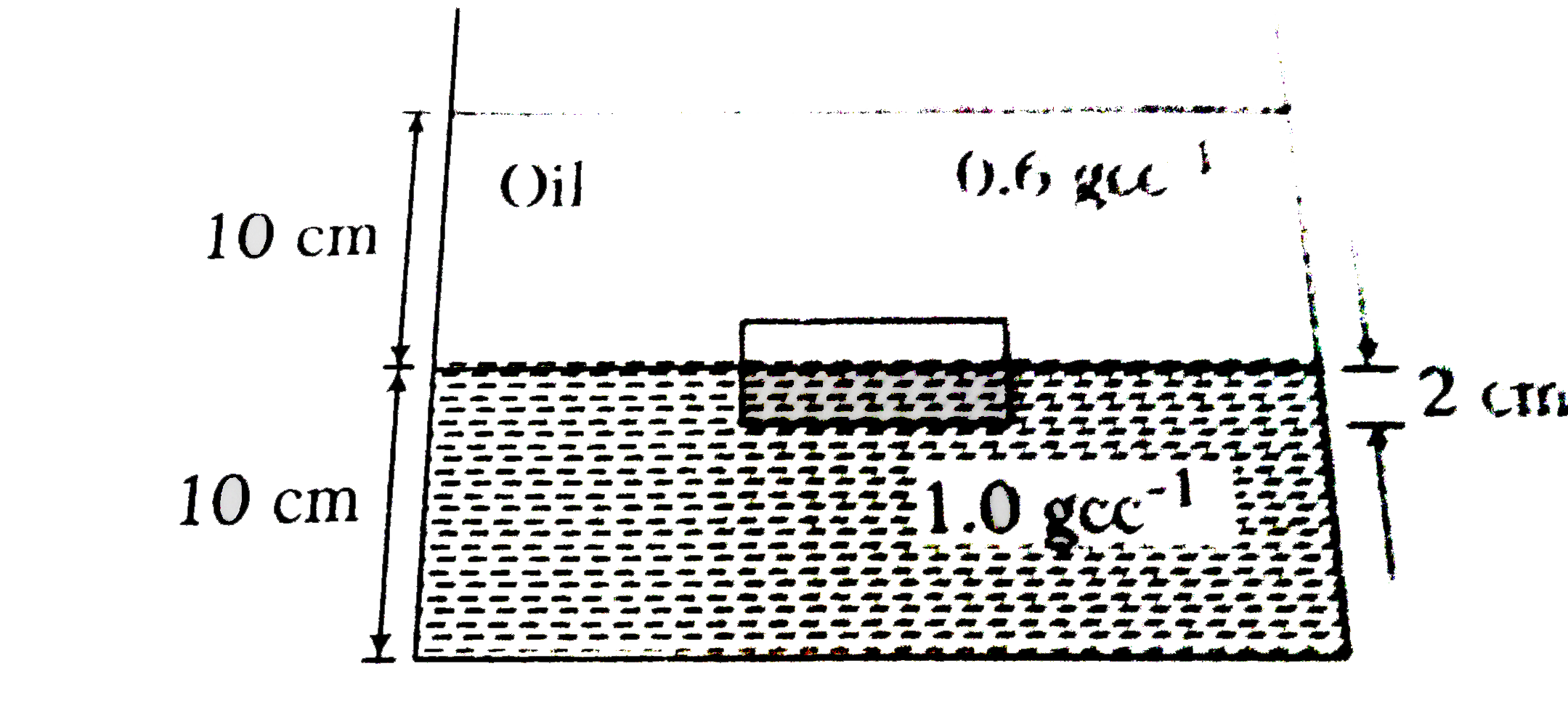
View SolutionA block of wood floats on water with 25 th of its volume above the water surface. Calculate the density of wood.
View SolutionA block of wood of volume 30cm3 floats in water with 20cm3 of its volume immersed.
Calculate the weight of the block of wood .
View SolutionThe density of water is ........ kg/m3.
View SolutionA block of wood of volume 30cm3 floats it water with 20cm3 of its volume immersed.
Calculate the density.
View SolutionA body floats in water with 40% of its volume outside water. When the same body floats in an oil. 60% of its volume remians outside oil. The relative density of oil is
View SolutionA block of wood of volume 25cm3 floats on water with 20cm3 of its volume immersed. Calculate (i) the density, and (ii) the weight of block of wood.
View SolutionA piece of wood floats in water with two-thirds of its volume submerged in water. In a certain oil it has 0.95 of its volume submerged. What is the density of wood and the oll?
View Solution
