The number density of molecules of a gas depends on their distance r from the origin as n(r)=n0e−α,r4 . Then the total number of molecules is proportional to:
n0α−3/4
√n0α½
n0αa/4
n0α−3
The correct Answer is:A
Step by step video & image solution for The number density of molecules of a gas depends on their distance r from the origin as n (r) = n_0 e^(-alpha, r^4) . Then the total number of molecules is proportional to: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
The mean free path of molecules of a gas (radius r) is inversely proportional to
View SolutionA : Number of air molecules in a room in winter is more than the number of molecules in the same room in summer.
R : At a given pressure and volume, the number of molecules of a given mass of a gas is directly proportional to the absolute temperature.View SolutionThe electric field inside a sphere having charge density related to the distance from the centre asρ=αr (α is a constant) is :
View SolutionA large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
View SolutionThe distribution of the molecular velocities of gas molecules at any temperature T is shown below. (The plot below is known as Maxwell's distribution of molecular speeds.)
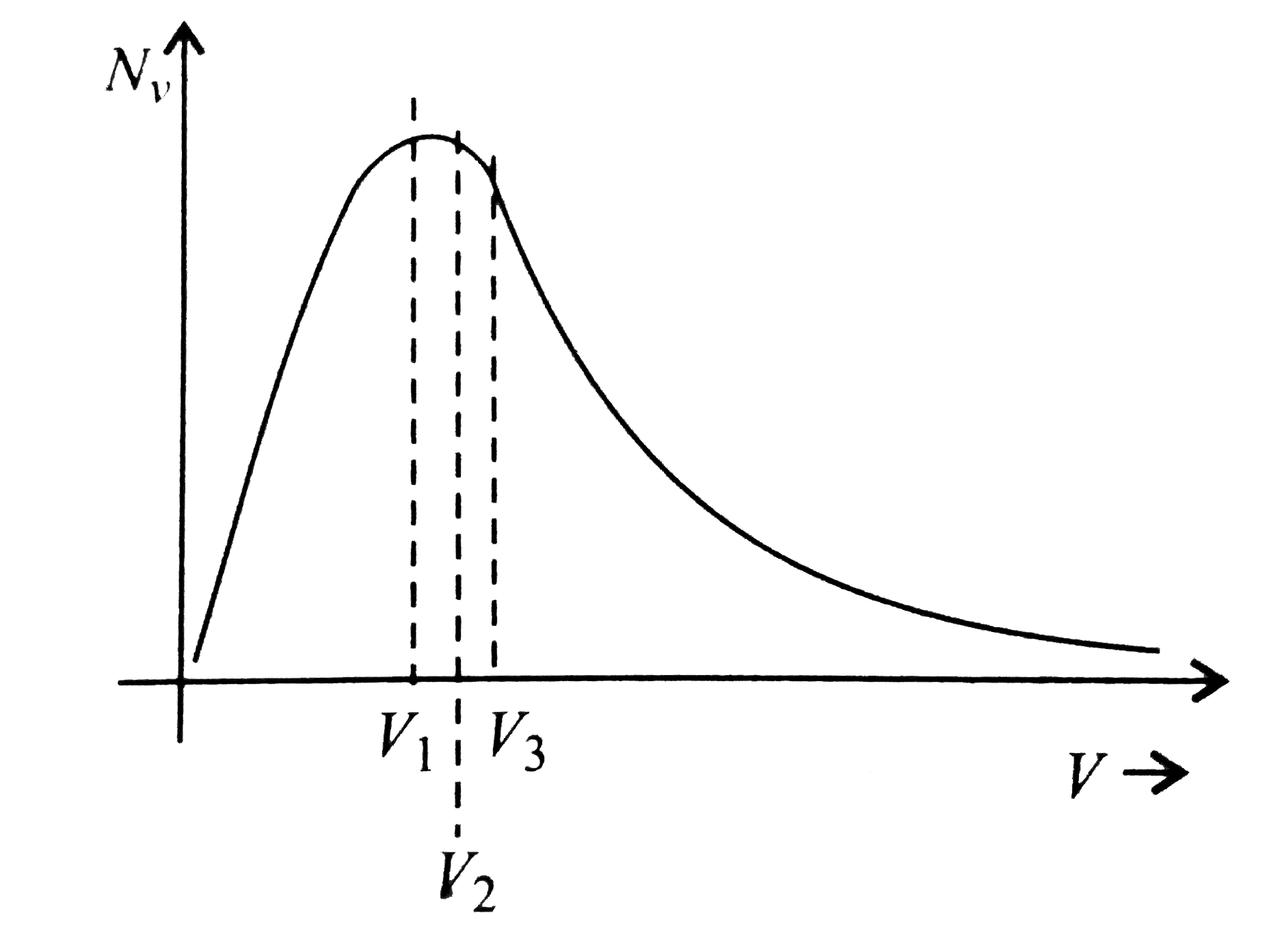
where
v is molecular velocity
n is number of molecules having velocity v
Let us define ΔNv, which is equal to the number of molecules between the velocity range v and v+Δv, given by
ΔNv=4πNa3e−bv2v2Δv
where
N is total number of molecules
a=√M02πRT and b=M02RT
R is universal gas constant
T is temperature of the gas
M0 is molecular weight of the gas
Answer the following question:
SI units of b areView SolutionThe distribution of the molecular velocities of gas molecules at any temperature T is shown below. (The plot below is known as Maxwell's distribution of molecular speeds.)
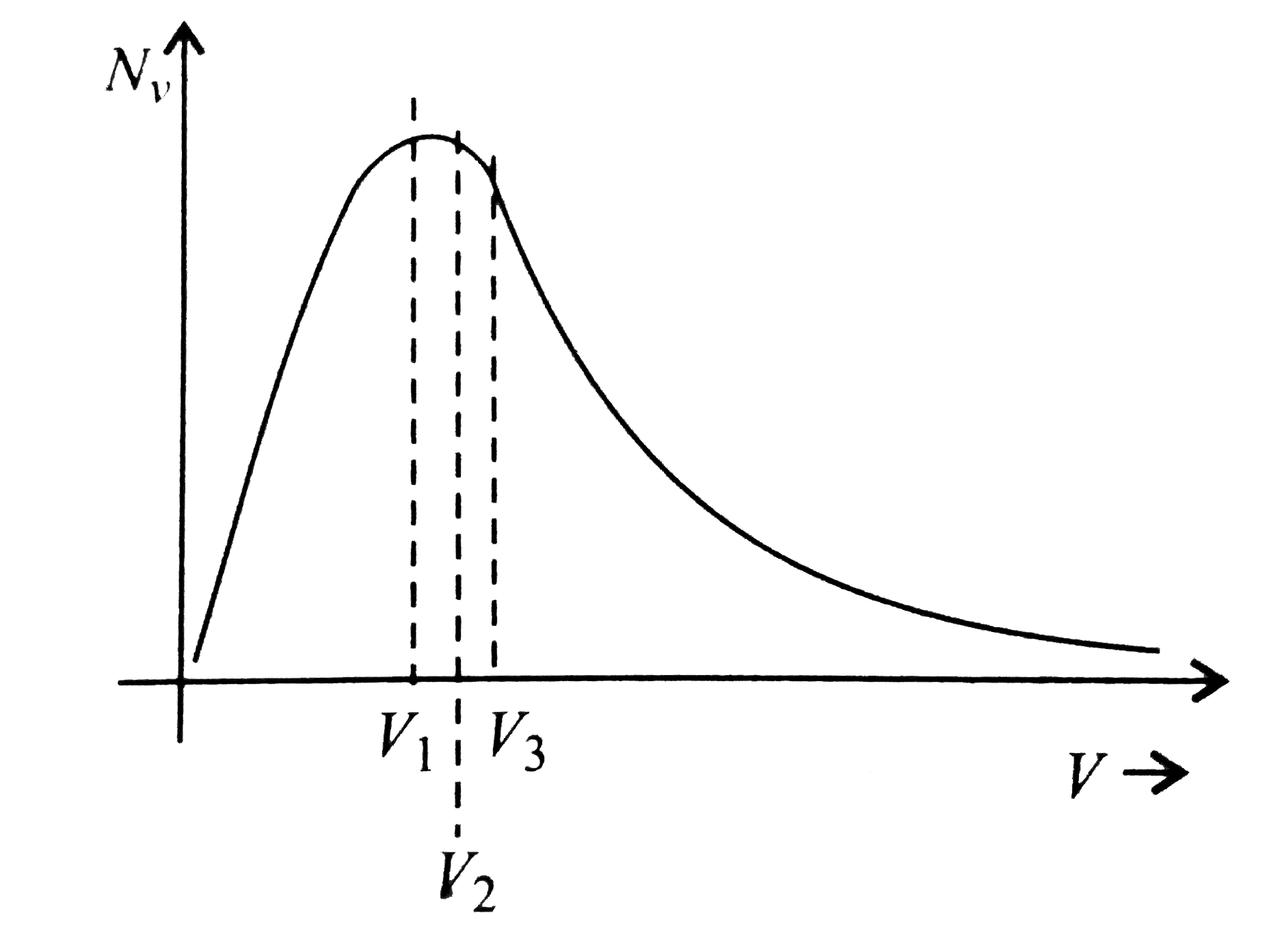
where
v is molecular velocity
n is number of molecules having velocity v
Let us define ΔNv, which is equal to the number of molecules between the velocity range v and v+Δv, given by
ΔNv=4πNa3e−bv2v2Δv
where
N is total number of molecules
a=√M02πRT and b=M02RT
R is universal gas constant
T is temperature of the gas
M0 is molecular weight of the gas
Answer the following question:
SI units of a areView SolutionCharge density of a sphere of radius R is ρ=ρ0r where r is distance from centre of sphere.Total charge of sphere will be
 View Solution
View Solutionif the mean free path of a molecule of an ideal gas having diameter 10−8cm is 10−4cm, calculate the number density of the gas molecule.
View SolutionThe electric field in a region is directed outward and is proportional to the distance r from the origin, Taking the electric potential at origin to be zero,
View SolutionLet f(n)=n∑r=0n∑k=r(kr). Find the total number of divisors of f(9).
View Solution

